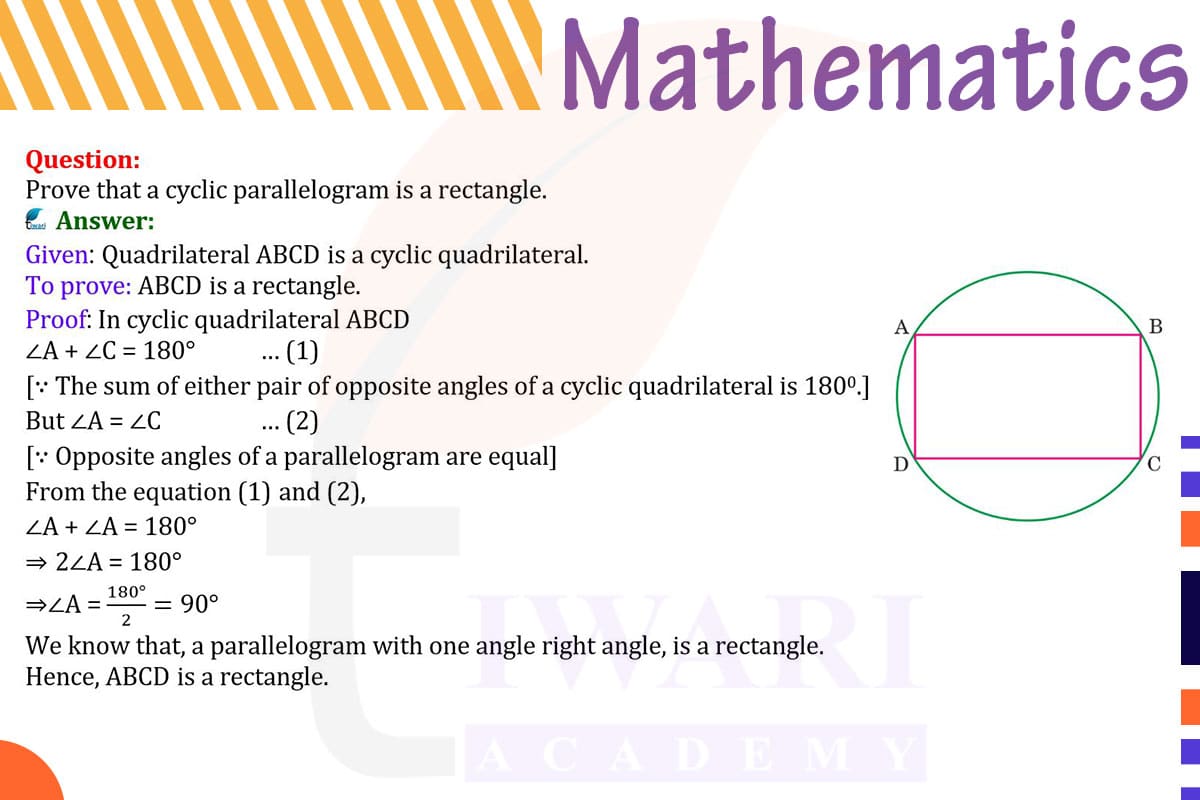
To prove that a cyclic parallelogram is a rectangle, consider a parallelogram ABCD inscribed in a circle. In a cyclic quadrilateral (a quadrilateral inscribed in a circle), the opposite angles are supplementary, meaning they add up to 180°.
In parallelogram ABCD, opposite angles are equal (a property of parallelograms). Let’s say ∠A and ∠C are two opposite angles. Since ABCD is cyclic, ∠A and ∠C must add up to 180°. But since they are equal (being opposite angles of a parallelogram), each must be 90°.
Therefore, all angles in the parallelogram are right angles, making it a rectangle. This proves that a cyclic parallelogram is always a rectangle.
Let’s discuss in detail
Cyclic Parallelograms and Geometric Properties
In the realm of geometry, the concept of a cyclic parallelogram presents an intriguing case study. A cyclic parallelogram is a four-sided figure (parallelogram) that can be inscribed within a circle. The challenge lies in proving that such a parallelogram is, in fact, a rectangle. This proof requires an understanding of both the properties of parallelograms and the characteristics of cyclic quadrilaterals. By exploring the interplay between these geometric principles, we can establish the conditions under which a cyclic parallelogram must necessarily be a rectangle.
Understanding Parallelograms and Their Properties
A parallelogram is a quadrilateral with opposite sides that are parallel and equal in length. Additionally, in a parallelogram, opposite angles are equal, and consecutive angles are supplementary (their sum equals 180°). These properties are fundamental to the nature of parallelograms and form the basis for further exploration. In our scenario, we are dealing with a parallelogram inscribed in a circle, which adds another layer of geometric properties to consider.
The Concept of Cyclic Quadrilaterals
A cyclic quadrilateral is a quadrilateral that can be inscribed within a circle, meaning all its vertices lie on the circumference of the circle. One of the key properties of cyclic quadrilaterals is that the sum of each pair of opposite angles is 180° (supplementary angles). This property is crucial in our proof, as it applies to any quadrilateral that can be inscribed in a circle, including our parallelogram.
Applying Cyclic Properties to the Parallelogram
In our cyclic parallelogram ABCD, by the property of cyclic quadrilaterals, the opposite angles are supplementary. Let’s consider angles ∠A and ∠C. According to the cyclic quadrilateral property, ∠A + ∠C = 180°. However, since ABCD is a parallelogram, opposite angles are also equal, which means ∠A = ∠C. The only way for two equal angles to sum up to 180° is if they are both right angles (90° each).
Concluding that All Angles are Right Angles
Since ∠A and ∠C are right angles, by the properties of a parallelogram, the other set of opposite angles (∠B and ∠D) must also be right angles. This is because in a parallelogram, consecutive angles are supplementary. Therefore, if one angle is 90°, the adjacent angle must also be 90° to satisfy the supplementary condition. This leads to the conclusion that all angles in the parallelogram are right angles.
Proving the Parallelogram is a Rectangle
In conclusion, we have established that in a cyclic parallelogram, all angles are right angles. A quadrilateral with four right angles is defined as a rectangle. Therefore, we have proven that a cyclic parallelogram is always a rectangle. This proof not only demonstrates a fascinating aspect of geometric figures but also highlights the elegant interplay between different geometric properties and principles. It underscores the beauty and logical coherence inherent in the study of geometry.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.3 in Detail

