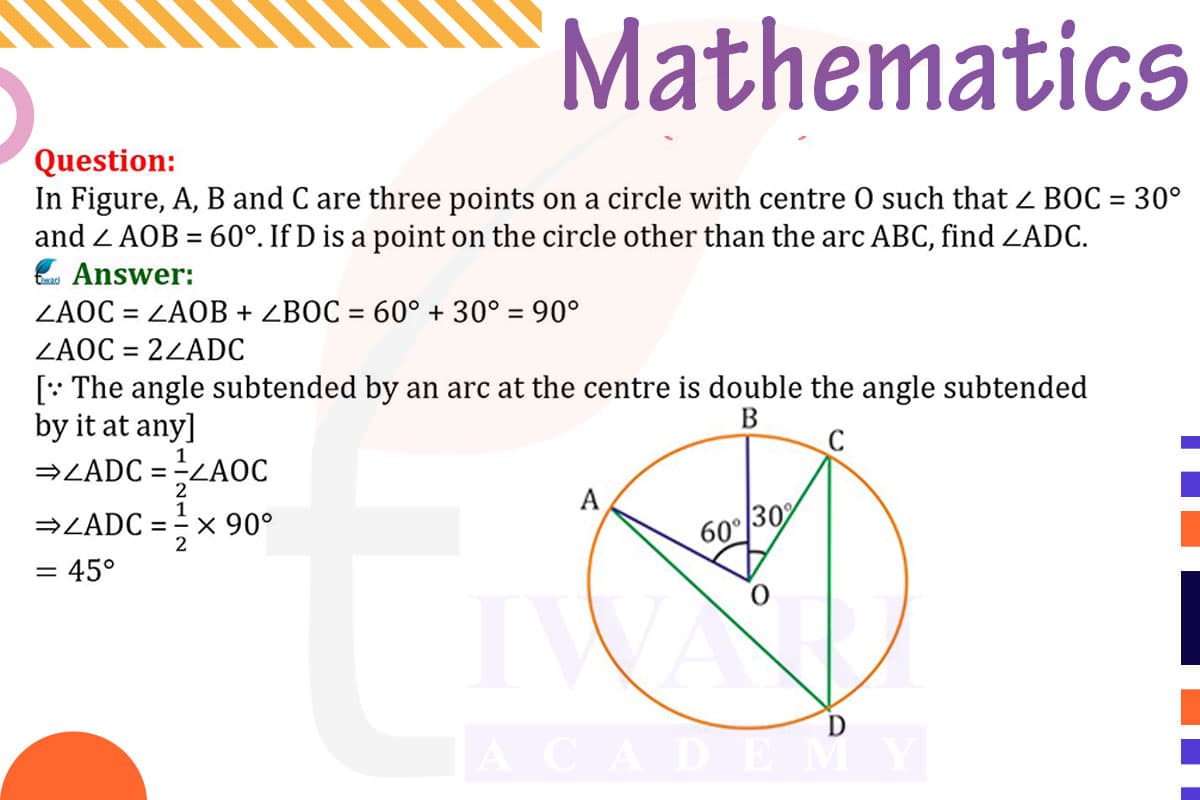
To find ∠ADC when A, B, and C are points on a circle with center O, and ∠BOC = 30° and ∠AOB = 60°, and D is a point on the circle other than the arc ABC, we use the properties of angles in a circle.
∠BOC and ∠AOB are angles at the center of the circle. The angle at the center is twice the angle at the circumference on the same arc. Therefore, ∠BAC, which stands on arc BC, is half of ∠BOC, so ∠BAC = 15°. Similarly, ∠ABC, standing on arc AC, is half of ∠AOB, so ∠ABC = 30°.
In triangle ABC, the sum of angles is 180°. With ∠BAC = 15° and ∠ABC = 30°, ∠ACB = 180° – 15° – 30° = 135°.
Now, consider ∠ADC. D and B are points on the circle in the segment not containing A. The angle in a segment is constant, so ∠ADC = ∠ACB = 135°.
Let’s discuss in detail
Circle Geometry and Angle Relationships
In the realm of circle geometry, the relationships between angles formed by points on a circle and the circle’s center are fundamental. In this scenario, we have a circle with center O and points A, B, C, and D on its circumference. The given angles are ∠BOC = 30° and ∠AOB = 60°. Our objective is to determine the measure of ∠ADC, where D is a point on the circle other than the arc ABC. This problem is a classic example of applying the principles of circle geometry to find unknown angles.
Understanding Central and Circumferential Angles
Central angles in a circle, like ∠BOC and ∠AOB, have a unique property: they are twice the size of the angles at the circumference that stand on the same arc. This principle is key to solving our problem. ∠BOC and ∠AOB are central angles, and their corresponding circumferential angles will be half of their measures. This relationship allows us to calculate the angles at points A, B, and C on the circumference of the circle.
Calculating Angles at the Circumference
Using the property that the angle at the center is twice the angle at the circumference, we find that ∠BAC, which subtends the same arc as ∠BOC, is half of 30°, thus ∠BAC = 15°. Similarly, ∠ABC, which subtends the same arc as ∠AOB, is half of 60°, making ∠ABC = 30°. These calculations are crucial for understanding the angular relationships in the circle and for determining the measure of ∠ADC.
Determining the Angle in Triangle ABC
In triangle ABC, the sum of the internal angles is always 180°. We already know two angles: ∠BAC = 15° and ∠ABC = 30°. Therefore, the third angle, ∠ACB, can be calculated as 180° – 15° – 30° = 135°. This angle is significant because it will help us in determining the measure of ∠ADC.
Applying the Angle in the Same Segment Rule
The angle in the same segment rule states that angles in the same segment of a circle are equal. Since points D and B lie in the same segment of the circle, separated by the chord AC, the angle ∠ADC will be equal to ∠ACB. This is because both angles are subtended by the same arc AC.
Finding the Measure of ∠ADC
By applying the principles of circle geometry, we conclude that ∠ADC, which is in the same segment as ∠ACB and subtended by the same arc, is equal to ∠ACB. Therefore, ∠ADC = 135°. This solution not only demonstrates the application of geometric principles but also highlights the elegance and consistency of mathematical relationships in circle geometry. Understanding these concepts allows for a deeper appreciation of the symmetry and patterns inherent in geometric shapes.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.3 in Detail

