To find ∠BAC when A, B, C, and D are points on a circle, and AC and BD intersect at E with ∠BEC = 130° and ∠ECD = 20°, we use the properties of angles in a circle.
First, consider ∠BEC and ∠ECD. These angles form a linear pair at point E, so they add up to 180°. Since ∠ECD = 20°, ∠BEC = 180° – 20° = 160°.
In a circle, the angle subtended by an arc at the center is twice the angle subtended by the same arc at any point on the circumference. Since ∠BEC is subtended by arc BD, ∠BDC at the center is twice ∠BEC, so ∠BDC = 2 × 160° = 320°.
However, the maximum angle at the center can only be 360°. Since ∠BDC is 320°, the remaining angle ∠BAD is 360° – 320° = 40°.
∠BAC is half of ∠BAD because they are subtended by the same arc BC. Therefore, ∠BAC = 40° / 2 = 20°.
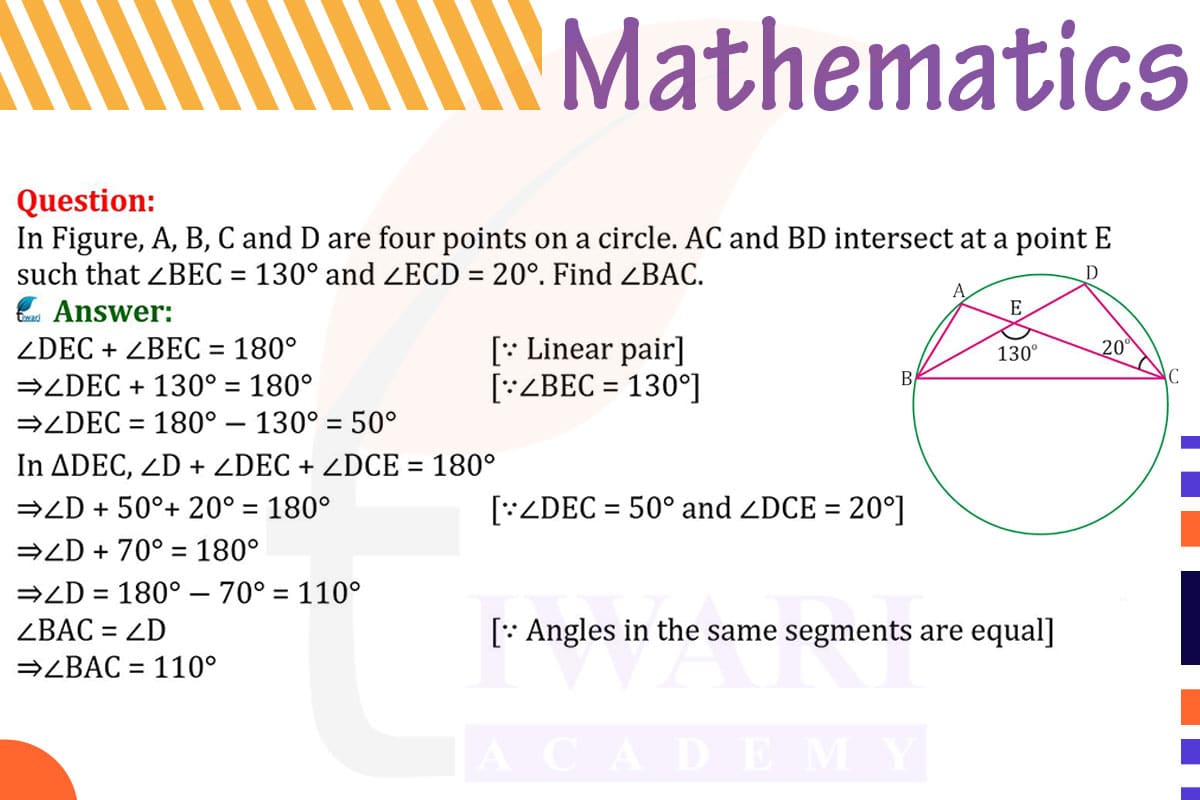
Let’s discuss in detail
Circle Geometry and Intersecting Chords
In the realm of circle geometry, the study of intersecting chords and the angles they create is a fundamental aspect. In this problem, we have a circle with points A, B, C, and D, and chords AC and BD intersecting at point E. Given are the angles ∠BEC = 130° and ∠ECD = 20°. Our goal is to determine the measure of ∠BAC, a task that involves applying principles of circle geometry and the properties of intersecting chords.
Understanding the Linear Pair of Angles at Point E
At the intersection point E of the chords AC and BD, the angles ∠BEC and ∠ECD form a linear pair. This means that their sum is 180°. Given that ∠ECD is 20°, we can calculate ∠BEC as 180° – 20° = 160°. This calculation is crucial as it helps in understanding the relationship between the angles formed by intersecting chords in a circle.
Exploring the Angle Subtended by Arc BD
In circle geometry, the angle subtended by an arc at the center of the circle is twice the angle subtended by the same arc at any point on the circumference. Since ∠BEC (160°) is subtended by arc BD, the angle at the center of the circle over arc BD, denoted as ∠BDC, will be twice ∠BEC. Therefore, ∠BDC = 2 × 160° = 320°.
Analyzing the Total Angle at the Circle’s Center
The total angle around a point, in this case, the center of the circle, is 360°. Having calculated ∠BDC as 320°, we can determine the remaining angle at the center, ∠BAD, by subtracting ∠BDC from 360°. Thus, ∠BAD = 360° – 320° = 40°. This angle is significant as it is subtended by the same arc BC that subtends ∠BAC.
Calculating ∠BAC
Since ∠BAD and ∠BAC are angles subtended by the same arc BC, and ∠BAD is at the center while ∠BAC is at the circumference, ∠BAC is half of ∠BAD. This is due to the property that the angle at the center is twice the angle at the circumference. Therefore, ∠BAC = ∠BAD / 2 = 40° / 2 = 20°.
Application of Circle Geometry Principles
In conclusion, by applying the principles of circle geometry, specifically those related to intersecting chords and angles subtended by arcs, we find that ∠BAC in the given circle is 20°. This problem illustrates the interconnectedness and elegance of geometric principles and their application in solving complex problems. Understanding these relationships enhances our comprehension of spatial relationships and the beauty of geometry in explaining the world around us.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.3 in Detail

