In Figure, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.

Updated on January 6, 2024
by Tiwari Academy

In a cyclic quadrilateral ABCD, where ∠ABC = 69° and ∠ACB = 31°, we can find ∠BDC using the properties of cyclic quadrilaterals.
In a cyclic quadrilateral, the opposite angles are supplementary, meaning they add up to 180°. Since ∠ACB = 31°, this angle is part of triangle ABC which is inscribed in the circle. The angle ∠ACB is subtended by the same arc as angle ∠ADB (the angle at the opposite side of the circle). Therefore, ∠ADB = 31°.
Since ∠ADB and ∠BDC are opposite angles in cyclic quadrilateral ABCD, ∠BDC = 180° – ∠ADB = 180° – 31° = 149°.

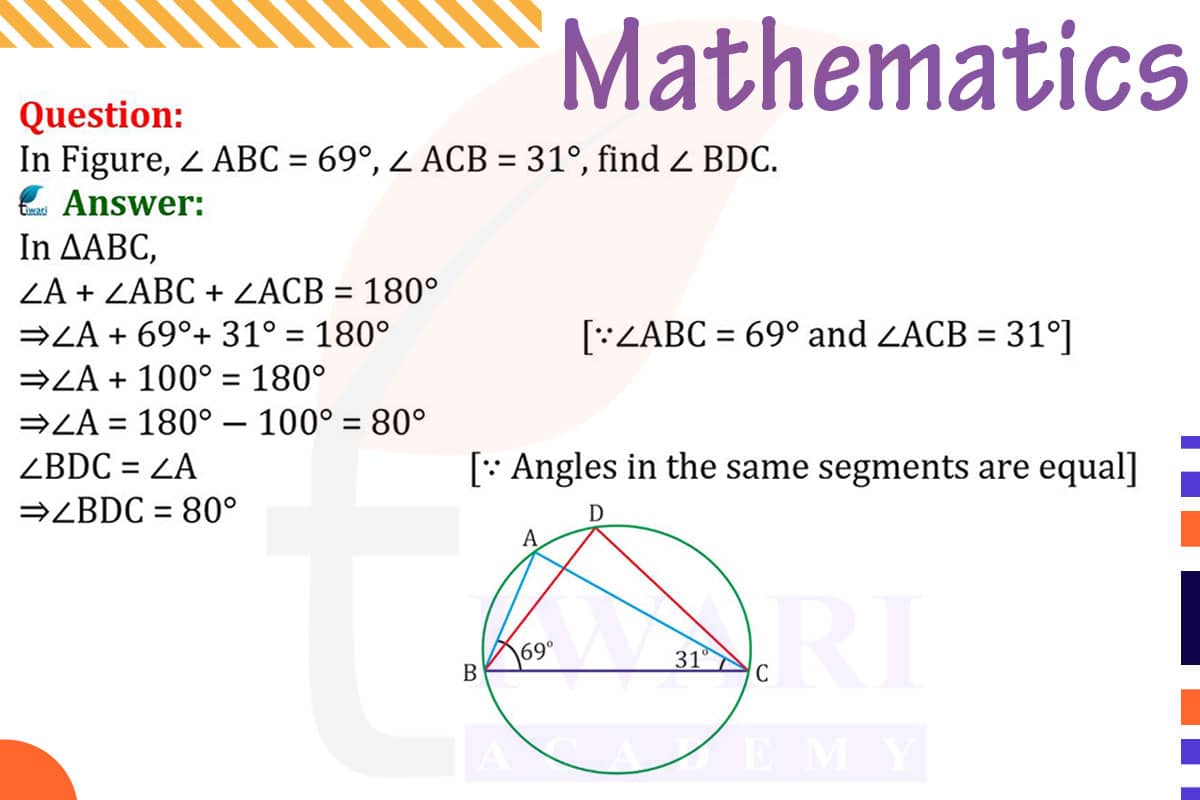
In Figure, ∠ABC = 69°, ∠ACB = 31°, find BDC.

Let’s discuss in detail

Cyclic Quadrilaterals and Angle Relationships

In the study of circle geometry, cyclic quadrilaterals offer fascinating insights into angle relationships. A cyclic quadrilateral is a four-sided figure with all its vertices lying on the circumference of a circle. In such a quadrilateral, certain angle properties hold true, which are crucial for solving problems like the one at hand. We are given a cyclic quadrilateral ABCD with angles ∠ABC = 69° and ∠ACB = 31°, and our task is to find the measure of ∠BDC.

Understanding the Properties of Cyclic Quadrilaterals

One of the key properties of cyclic quadrilaterals is that the sum of each pair of opposite angles is 180°. This supplementary relationship is a direct consequence of the quadrilateral’s vertices lying on a circle. This property will be instrumental in determining the unknown angle ∠BDC in quadrilateral ABCD.

Analyzing Triangle ABC within the Cyclic Quadrilateral

In the cyclic quadrilateral ABCD, triangle ABC is a critical component. The given angles, ∠ABC = 69° and ∠ACB = 31°, belong to this triangle. Since triangle ABC is inscribed in the circle, the angle ∠ACB is subtended by arc AD. This subtended angle relationship is vital for understanding the angles formed at other points on the circle.

Determining Angle ∠ADB
The angle ∠ACB (31°) in triangle ABC subtends the same arc as angle ∠ADB in the cyclic quadrilateral. Since these angles are subtended by the same arc, they are equal. Therefore, ∠ADB, which is the angle at the opposite side of the circle from ∠ACB, is also 31°. This equality is a direct application of the angle subtended by the same arc theorem in circle geometry.

Calculating Angle ∠BDC

With ∠ADB determined as 31°, we can now calculate ∠BDC. Since ∠ADB and ∠BDC are opposite angles in the cyclic quadrilateral ABCD, and opposite angles in a cyclic quadrilateral are supplementary, ∠BDC equals 180° – ∠ADB. Therefore, ∠BDC = 180° – 31° = 149°. This calculation is based on the supplementary nature of opposite angles in a cyclic quadrilateral.

Geometric Principles in Cyclic Quadrilaterals

In conclusion, by applying the properties of cyclic quadrilaterals and the theorem of angles subtended by the same arc, we find that ∠BDC in the cyclic quadrilateral ABCD is 149°. This exercise demonstrates the elegance and interconnectedness of geometric principles, particularly in the context of cyclic quadrilaterals. Understanding these relationships allows for a deeper appreciation of geometry’s role in elucidating spatial relationships and solving complex problems.

Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.3 in Detail

In Figure, A,B and C are three points on a circle with centre O such that ∠ BOC = 30° and ∠ AOB = 60°. If D is a point on the circle other than the arc ABC, find ∠ADC.

A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.

In Figure, ∠PQR = 100°, where P, Q and R are points on a circle with centre O. Find ∠OPR.

In Figure, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.

In Figure, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC = 130° and ∠ECD = 20°. Find ∠BAC.

ABCD is a cyclic quadrilateral whose diagonals intersect at a point E. If ∠DBC = 70°, ∠BAC is 30°, find ∠BCD. Further, if AB = BC, find ∠ECD.

If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.

If the non-parallel sides of a trapezium are equal, prove that it is cyclic.

Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D and P, Q respectively. Prove that ∠ACP = ∠QCD.

If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.

ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠CAD = ∠CBD.