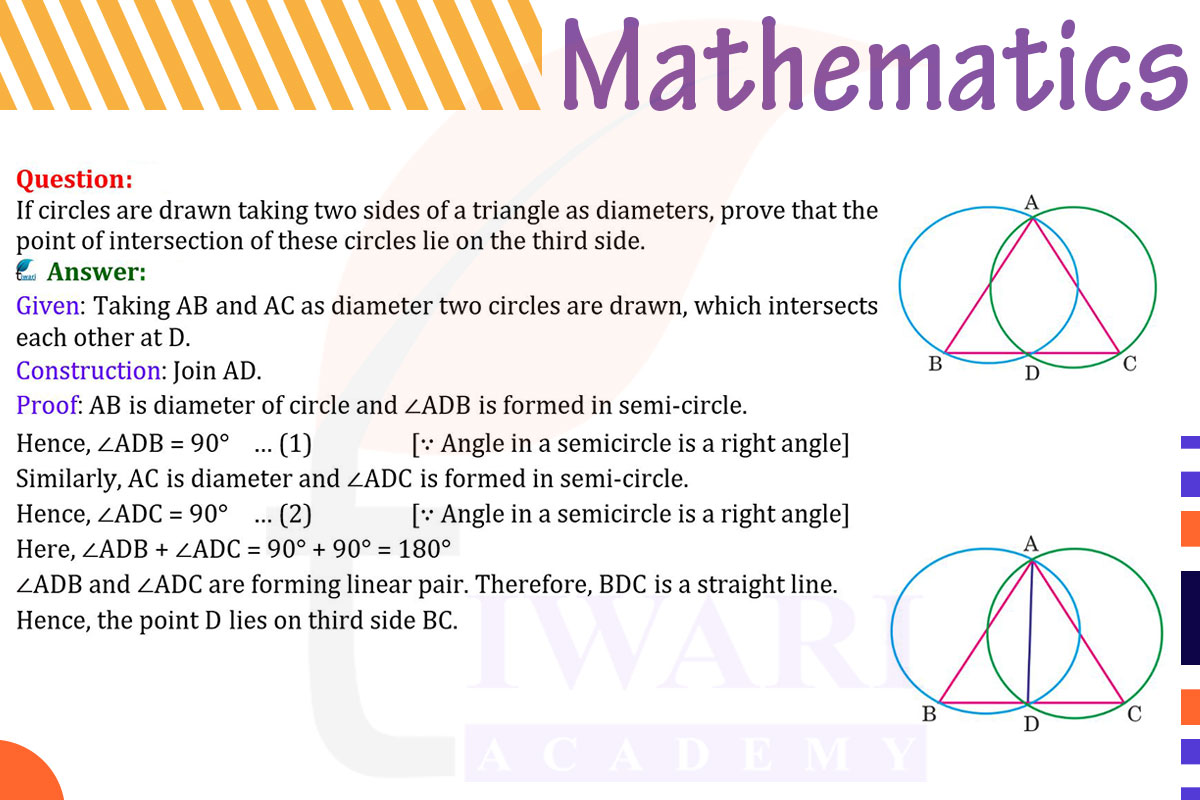
To prove that the point of intersection of two circles, drawn with two sides of a triangle as their diameters, lies on the third side of the triangle, consider a triangle ABC. Draw two circles, one with diameter AB and the other with diameter AC.
By the property of a circle, the angle subtended by a diameter at the circumference is a right angle. So, in the circle with diameter AB, any angle subtended at the circumference (like ∠ACB) is 90°. Similarly, in the circle with diameter AC, ∠ABC is 90°.
The intersection point of these two circles, other than A, must lie on both circles’ circumferences. Therefore, this point must satisfy both conditions of being 90° from AB and AC, which is only possible if it lies on BC, the third side of the triangle. Thus, the intersection point of the circles lies on side BC of triangle ABC.
Let’s discuss in detail
Circle Geometry in Relation to Triangles
In the fascinating world of geometry, the relationship between triangles and circles offers numerous intriguing properties. One such property involves drawing circles with the sides of a triangle as diameters. In this scenario, we are tasked with proving that if circles are drawn taking two sides of a triangle as diameters, the point of intersection of these circles (other than the triangle’s vertex) will lie on the third side of the triangle. This proof hinges on the fundamental properties of circles and triangles.
The Property of Angles Subtended by Diameters
A key principle in circle geometry is that an angle subtended by a diameter at the circumference of a circle is a right angle (90 degrees). This property is crucial for our proof. When a circle is drawn with a diameter along one side of a triangle, any angle formed at the circumference opposite this diameter is a right angle. This principle will be applied to two circles drawn on two sides of a given triangle.
Constructing Circles on Triangle Sides
Consider a triangle ABC. We construct two circles: one with diameter AB and the other with diameter AC. According to the aforementioned property, in the circle with diameter AB, any angle subtended at the circumference opposite AB is a right angle. Therefore, ∠ACB, formed at the circumference of this circle, is 90 degrees. Similarly, in the circle with diameter AC, ∠ABC is 90 degrees.
Intersection Point of the Circles
The intersection point of the two circles, other than the common vertex A, is a point that lies on the circumference of both circles. This point, by the property of the circles, must form a right angle with both diameters AB and AC. The only location where this condition can be satisfied simultaneously for both circles is on the third side of the triangle, BC.
Geometric Justification of the Intersection Point
The intersection point, let’s call it P, forms two right angles: ∠APB and ∠APC, as it lies on the circumferences of both circles. Since these angles are right angles, and they are subtended by the diameters AB and AC, respectively, point P must lie directly opposite these diameters. The only place where this is geometrically possible, given the configuration of the triangle and the circles, is on the line segment BC.
Proving the Intersection Point Lies on the Triangle Side
In conclusion, by utilizing the property of angles subtended by diameters in circles and applying this to circles drawn on two sides of a triangle, we have proven that the intersection point of these circles (other than the vertex) lies on the third side of the triangle. This proof not only demonstrates a beautiful intersection of circle and triangle properties but also highlights the elegance and interconnectedness of geometric principles, providing a deeper understanding of the relationships between different geometric shapes.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.3 in Detail

