NCERT Solutions for Class 9 Maths Chapter 9 Exercise 9.3 Circles updated for CBSE and State board students session 2025-26. Grade 9 Maths Solutions are available in Hindi and English Medium prepared according to new NCERT textbooks for State Boards and CBSE.
Class 9 Maths Exercise 9.3 Questions in Detail
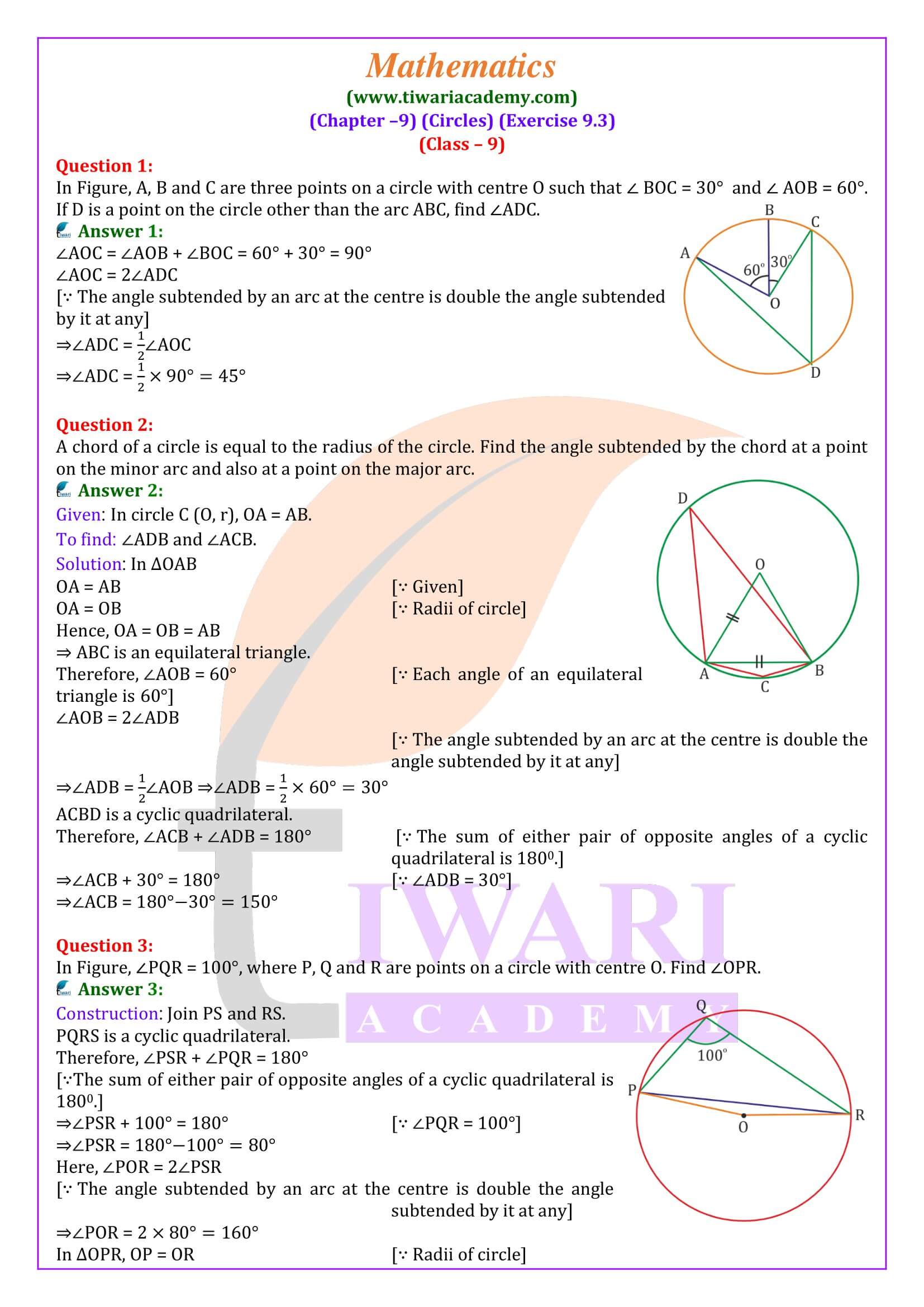
- In Figure, A,B and C are three points on a circle with centre O such that ∠ BOC = 30° and ∠ AOB = 60°. If D is a point on the circle other than the arc ABC, find ∠ADC.
- A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.
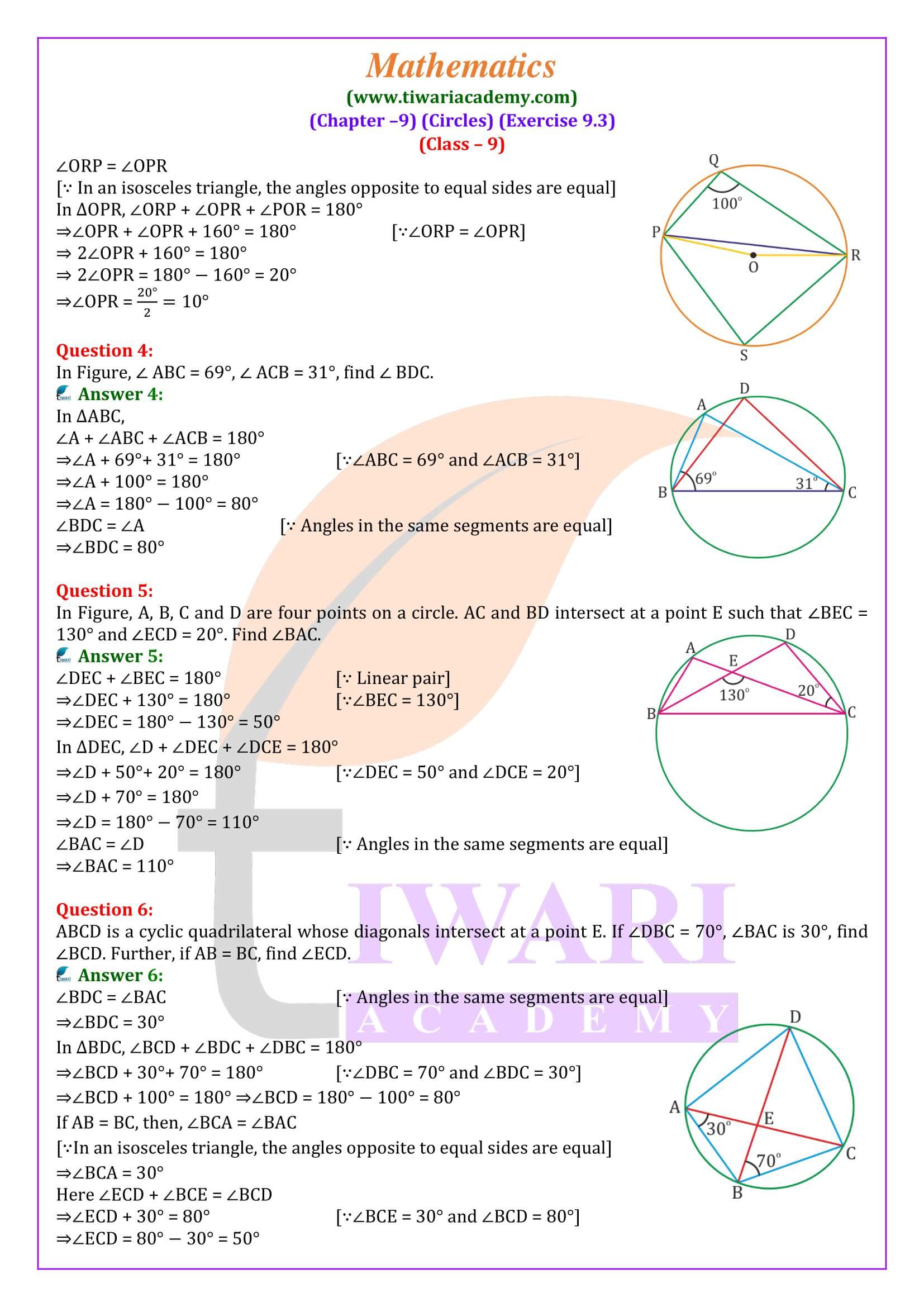
- In Figure, ∠PQR = 100°, where P, Q and R are points on a circle with centre O. Find ∠OPR.
- In Figure, ∠ABC = 69°, ∠ACB = 31°, find ∠BDC.
- In Figure, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠BEC = 130° and ∠ECD = 20°. Find ∠BAC.
- ABCD is a cyclic quadrilateral whose diagonals intersect at a point E. If ∠DBC = 70°, ∠BAC is 30°, find ∠BCD. Further, if AB = BC, find ∠ECD.
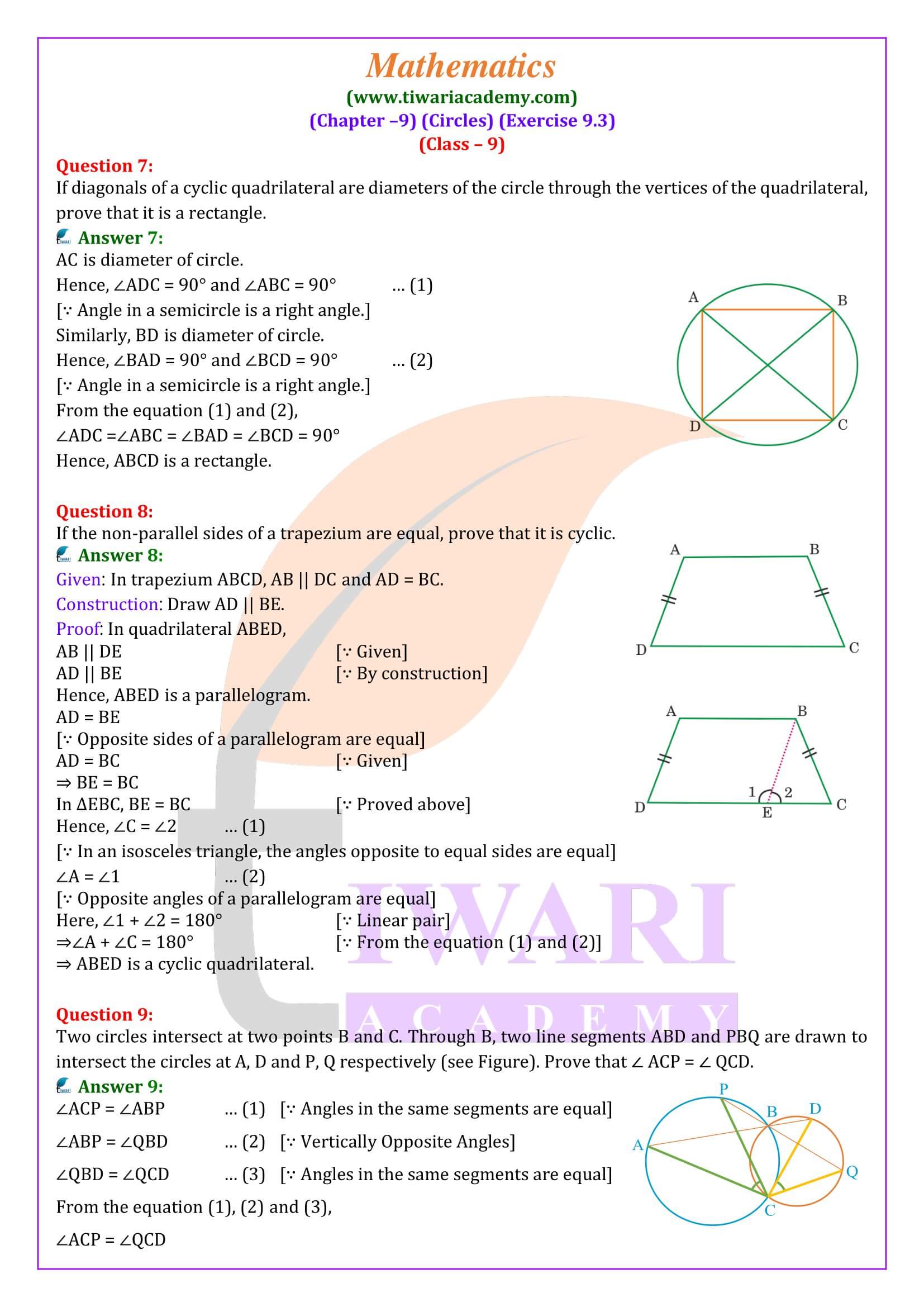
- If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.
- If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
- Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D and P, Q respectively. Prove that ∠ACP = ∠QCD.
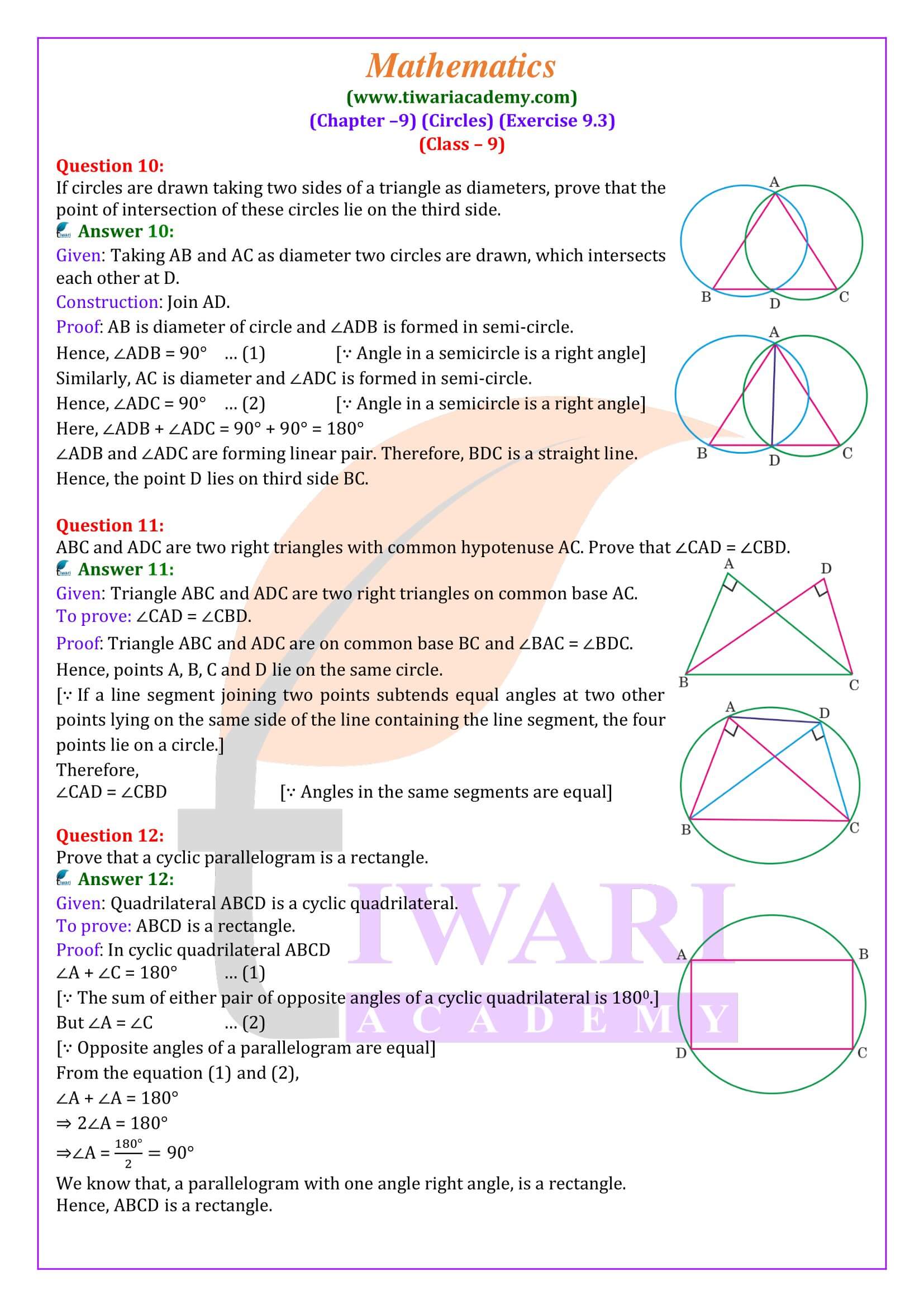
- If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.
- ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠CAD = ∠CBD.
- Prove that a cyclic parallelogram is a rectangle.

| Class: 9 | Mathematics |
| Chapter: 9 | Exercise: 9.3 |
| Topic Name: | Circles |
| Study Material: | Text, PDF and Videos |
| Academic Session: | CBSE 2025-26 |
| Medium: | Hindi and English Medium |
Theorems related to Exercise 9.3 Class 9
This is the most interesting and exciting of the present topic. Few of the interesting things that we are going to study are, if two chords of a circles are equal in length, then there corresponding arcs are congruent.
Conversely if two arcs are “congruent”, then their corresponding chords must be equal. Re –collect the meaning of the word “congruent” and the word “segment”.
Congruence of Circles in Class 9 Exercise 9.3
The term congruent means that if two figures are placed one above the other, they will have similar shape. i.e., the shape will be exactly the same. The term segment means the region between a chord and either of its arcs.
You may also say that a segment is the part of the whole circle. The entire circle can be divided into two segment minor and major. These two may be equal or unequal. They can equal only if both the parts are semicircles.
Important theorems of Exercise 9.3 in 9th Maths
Another interesting feature to be learn in this exercise that theorem 8 this says that the angles subtended by an arc at the center of the circle is double the angle subtended by it at any point on remaining part of the circle.
One of the practical ways to learn this topic or for that matter any topic in geometry is to draw the required figure and see the proof by measuring the angles.
Application of theorem in questions of Exercise 9.3
As they say in English, that the taste of pudding is in the eating. So, if you draw the required figure check the angles.
You will really find that the angles at the centre is double the measure of angle on the remaining part of it. Exercise 9.3 contains 8 application based questions and they should be practiced by drawing proper diagrams.