To find the length of the common chord of two intersecting circles with radii 5 cm and 3 cm, and a center-to-center distance of 4 cm, we use right triangles. Imagine a line segment connecting the centers of the two circles. The common chord is perpendicular to this line and bisects it. This forms two right triangles, each with the common chord as the hypotenuse.
For the larger circle, the radius (5 cm) is the hypotenuse of a right triangle with one leg being half the distance between the centers (2 cm). Using Pythagoras’ theorem, the other leg (half the chord length) is √[5² − 2²] = √21 cm. Since the chord is bisected, its full length is 2√21 cm, approximately 9.17 cm.
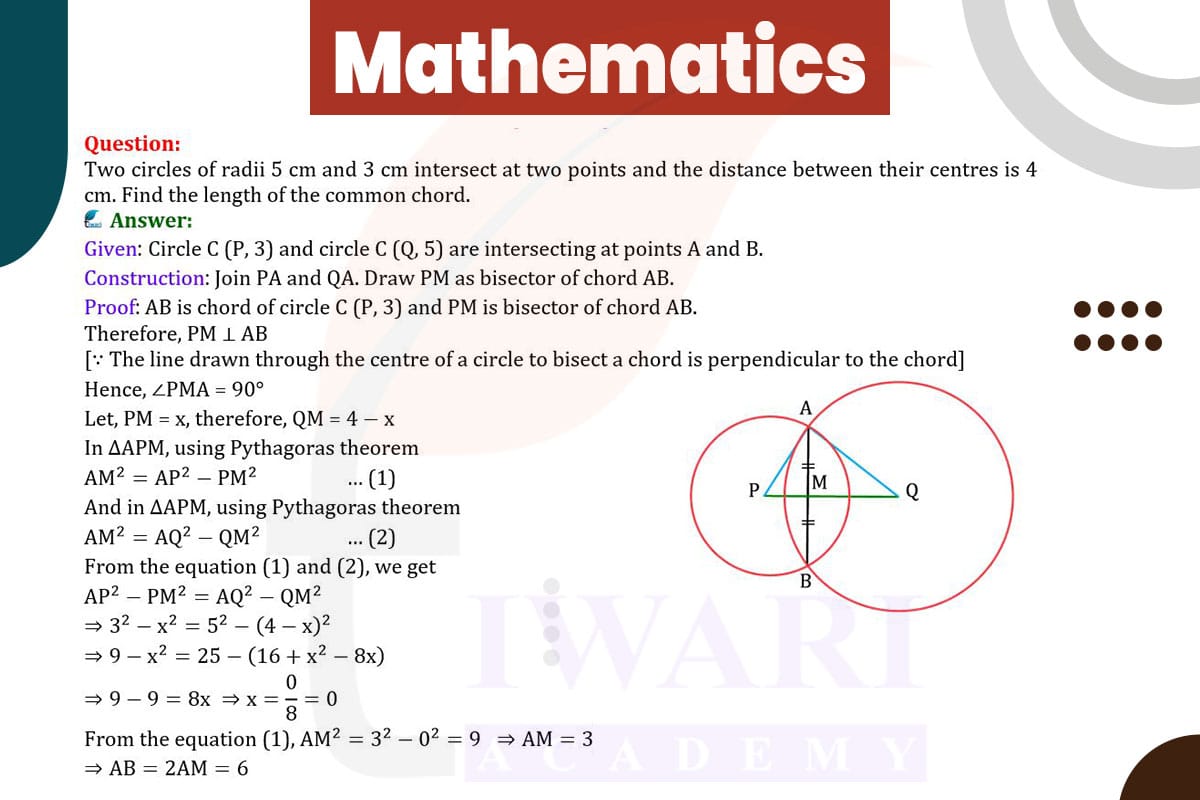
Let’s discuss in detail
Introduction to the Problem
The problem at hand involves two intersecting circles with different radii, specifically 5 cm and 3 cm. These circles intersect at two points, and the distance between their centers is given as 4 cm. The objective is to determine the length of the common chord that these circles share. This problem is a classic example of applying geometric principles, particularly the Pythagorean theorem, to solve real-world problems. Understanding the relationship between the radii of the circles, the distance between their centers, and the properties of the intersecting chord is key to finding the solution.
Understanding the Geometry of Intersecting Circles
When two circles intersect, they form a common chord that is shared by both circles. This chord is crucial in understanding the spatial relationship between the two circles. In our case, the circles have different radii, which adds a layer of complexity to the problem. The distance between the centers of these circles is a critical piece of information, as it helps in constructing right triangles that are instrumental in solving for the length of the chord.
Constructing Right Triangles
To solve the problem, we construct right triangles using the radii of the circles and the distance between their centers. The line segment connecting the centers of the two circles acts as the base for these right triangles. The common chord, which we are trying to find the length of, is perpendicular to this line and bisects it, thus forming two right triangles with the radii as their hypotenuses.
Applying the Pythagorean Theorem
The Pythagorean theorem states that in a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the other two sides. We apply this theorem to the right triangles formed in each circle. For the larger circle (radius 5 cm), the hypotenuse is 5 cm, and one leg is half the distance between the centers, which is 2 cm. We calculate the other leg, which is half the length of the common chord.
Calculating the Length of the Common Chord
Using the Pythagorean theorem, we find that the length of the other leg for the larger circle’s right triangle is √[5² − 2²] = √21 cm. This is half the length of the common chord. Therefore, the full length of the common chord is twice this value, which is 2√21 cm. This calculation gives us the precise length of the chord shared by the two intersecting circles.
Solving Geometric Problems
In conclusion, by applying geometric principles and the Pythagorean theorem, we have successfully determined the length of the common chord of two intersecting circles with given radii and center-to-center distance. This problem exemplifies how geometric concepts can be used to solve complex problems, providing a clear and precise answer. The length of the common chord in this scenario is approximately 9.17 cm, showcasing the elegance and utility of geometry in understanding and solving spatial relationships.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.2 in Detail

