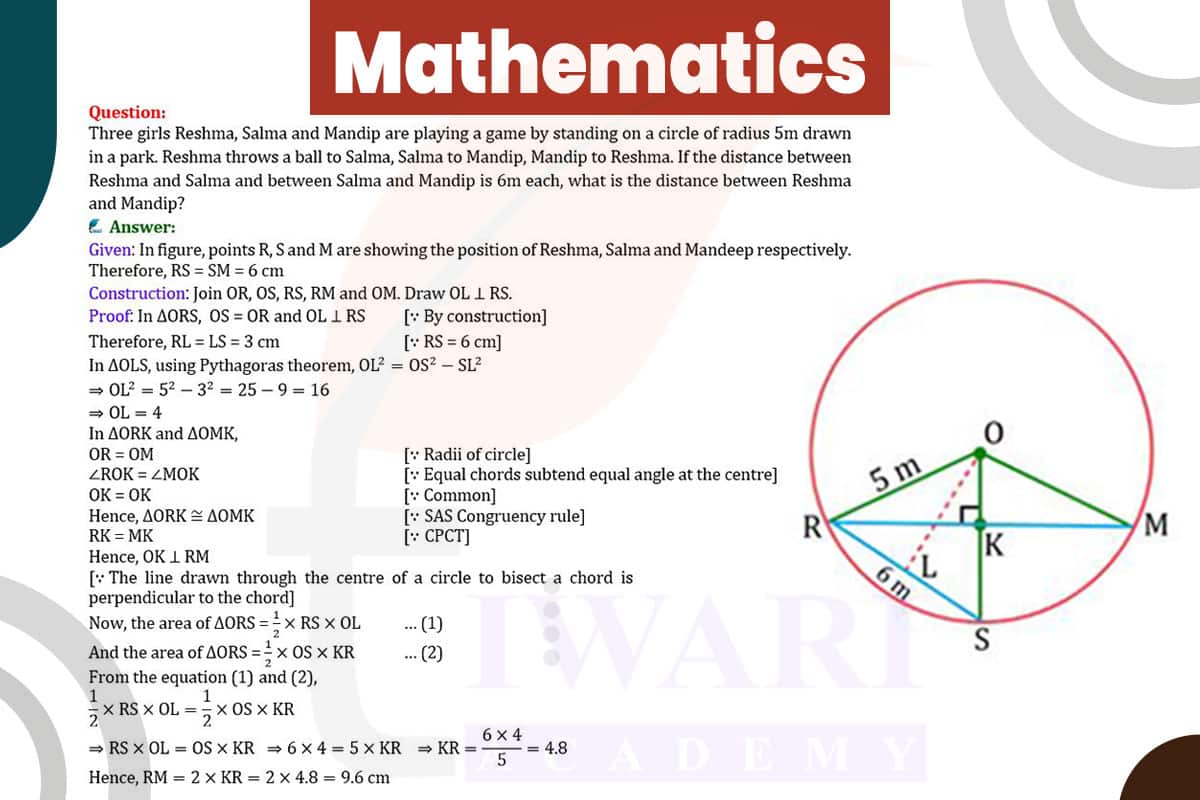
To find the distance between Reshma and Mandip in the scenario where they, along with Salma, are standing on a circle of radius 5m, and the distances between Reshma and Salma and between Salma and Mandip are 6m each, we can use the properties of a circle and triangles.
Reshma, Salma, and Mandip form an isosceles triangle with two sides (Reshma to Salma and Salma to Mandip) measuring 6m each. The base of this triangle is the line between Reshma and Mandip. To find this distance, we drop a perpendicular from Salma to the line joining Reshma and Mandip, bisecting it. This perpendicular is a radius of the circle (5m). We now have a right triangle with one leg as half the distance between Reshma and Mandip and the other leg as 5m, and the hypotenuse as 6m. Using Pythagoras’ theorem, the half distance can be calculated, and then doubled to find the full distance between Reshma and Mandip.
Let’s discuss in detail
Introduction to the Geometric Problem
In a park, three girls, Reshma, Salma, and Mandip, are engaging in a game that involves positioning themselves on a circle with a radius of 5 meters and throwing a ball to each other. Reshma throws to Salma, Salma to Mandip, and Mandip back to Reshma. The distances between Reshma and Salma and between Salma and Mandip are both 6 meters. The intriguing geometric problem here is to determine the distance between Reshma and Mandip. This scenario presents a practical application of circle and triangle geometry.
Understanding the Circle and Positions
The girls are standing on the circumference of a circle with a 5-meter radius. This circle forms the boundary within which they are positioned. The distances between them create a triangular formation within the circle. Since the distances from Reshma to Salma and Salma to Mandip are equal, the triangle formed by the positions of the girls is isosceles. The base of this triangle is the line segment between Reshma and Mandip, which we need to calculate.
Formation of an Isosceles Triangle
The equal distances of 6 meters between Reshma and Salma and between Salma and Mandip indicate that they form an isosceles triangle. In an isosceles triangle, two sides are equal, and the angles opposite these sides are also equal. This property will be crucial in our approach to finding the unknown distance between Reshma and Mandip.
Dropping a Perpendicular from Salma
To solve this problem, we drop a perpendicular from Salma, who is at the apex of the isosceles triangle, to the base, which is the line segment between Reshma and Mandip. This perpendicular bisects the base and also represents the radius of the circle, which is 5 meters. This creates two right-angled triangles within the isosceles triangle.
Applying the Pythagorean Theorem
In one of the right-angled triangles formed, we know the length of the perpendicular (5 meters) and the hypotenuse (6 meters, the distance from Salma to either Reshma or Mandip). We can apply the Pythagorean theorem to find the half-length of the base (distance between Reshma and Mandip). The theorem states that in a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
Calculating and Concluding the Distance
Using the Pythagorean theorem, we calculate the half-length of the base and then double it to find the full distance between Reshma and Mandip. This calculated distance provides the solution to our geometric problem, demonstrating how principles of geometry can be applied to solve real-world scenarios. This approach not only solves the problem at hand but also illustrates the practical application of geometric concepts in everyday situations.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.2 in Detail

