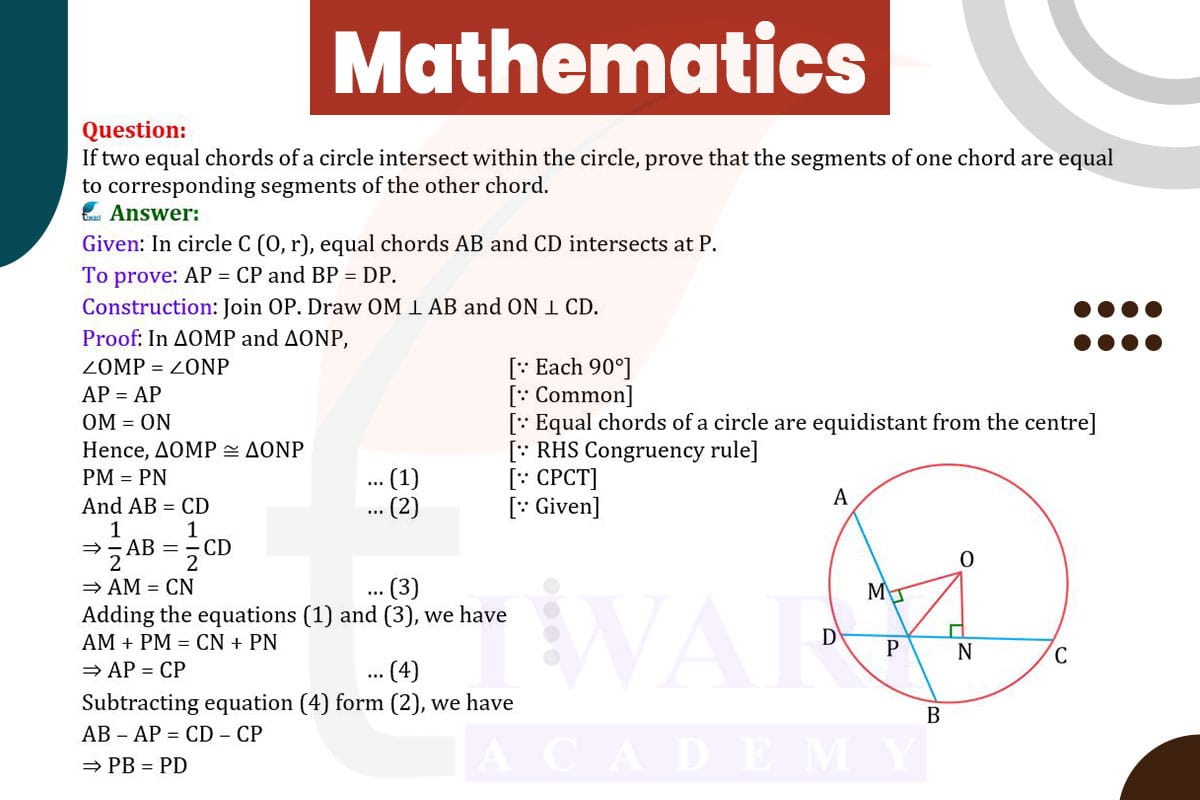
To prove that if two equal chords of a circle intersect within the circle, the segments of one chord are equal to the corresponding segments of the other chord, consider two equal chords AB and CD intersecting at point E. Triangles AEB and CED are formed. Since AB and CD are equal and radii to their endpoints are equal (being radii of the same circle), triangles AEB and CED are congruent by the Side-Side-Side (SSS) criterion. Therefore, corresponding segments AE = CE and BE = DE. Thus, the segments of one chord are equal to the corresponding segments of the other chord.
Let’s discuss in detail
Geometric Relationships in Circles
In the study of circle geometry, the properties of chords play a significant role. A chord is a straight line segment whose endpoints lie on the circumference of the circle. A fascinating scenario arises when two equal chords intersect within a circle. This intersection creates segments of chords, and an intriguing question is whether these segments are equal to each other. The proof of this equality not only demonstrates a fundamental geometric principle but also highlights the symmetry and harmony inherent in circular shapes.
Understanding Chords and Their Segments
When two chords intersect within a circle, they form four segments. In our case, we consider two equal chords, which means their lengths are identical. The point of intersection divides each chord into two segments. The geometric relationship between these segments is not immediately apparent but can be deduced through a series of logical geometric steps. The key lies in understanding the properties of the triangles formed by these intersecting chords.
Constructing Triangles from Intersecting Chords
Consider two equal chords AB and CD intersecting at point E within the circle. This intersection forms two triangles: AEB and CED. These triangles are crucial to our proof as they contain the segments of the intersecting chords. The equality of the chords AB and CD suggests a potential congruence between these triangles, which we will explore to understand the relationship between the segments.
Applying the Side-Side-Side (SSS) Criterion
To prove the triangles AEB and CED are congruent, we use the Side-Side-Side (SSS) criterion. This criterion states that if three sides of one triangle are equal to three sides of another triangle, the triangles are congruent. In our case, AB equals CD (as given), and the radii of the circle to the endpoints of these chords are equal (since all radii in a circle are equal). This sets the stage for proving the congruence of the triangles.
Proving the Equality of Chord Segments
With the congruence of triangles AEB and CED established, it follows that corresponding parts of congruent triangles are equal. This means that the segments AE and CE (formed by the intersection of chords AB and CD) are equal, as are the segments BE and DE. Therefore, the segments of one chord are equal to the corresponding segments of the other chord. This equality is a direct result of the congruence of the triangles formed by the intersecting chords.
Symmetry in Circle Geometry
In conclusion, the proof that equal chords intersecting within a circle create equal corresponding segments illustrates the beautiful symmetry and consistency in circle geometry. This property is a testament to the elegance of geometric principles and their ability to reveal underlying patterns and relationships in seemingly complex shapes. Understanding such relationships is crucial in the broader context of geometric studies and their practical applications in various scientific and engineering fields.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.2 in Detail

