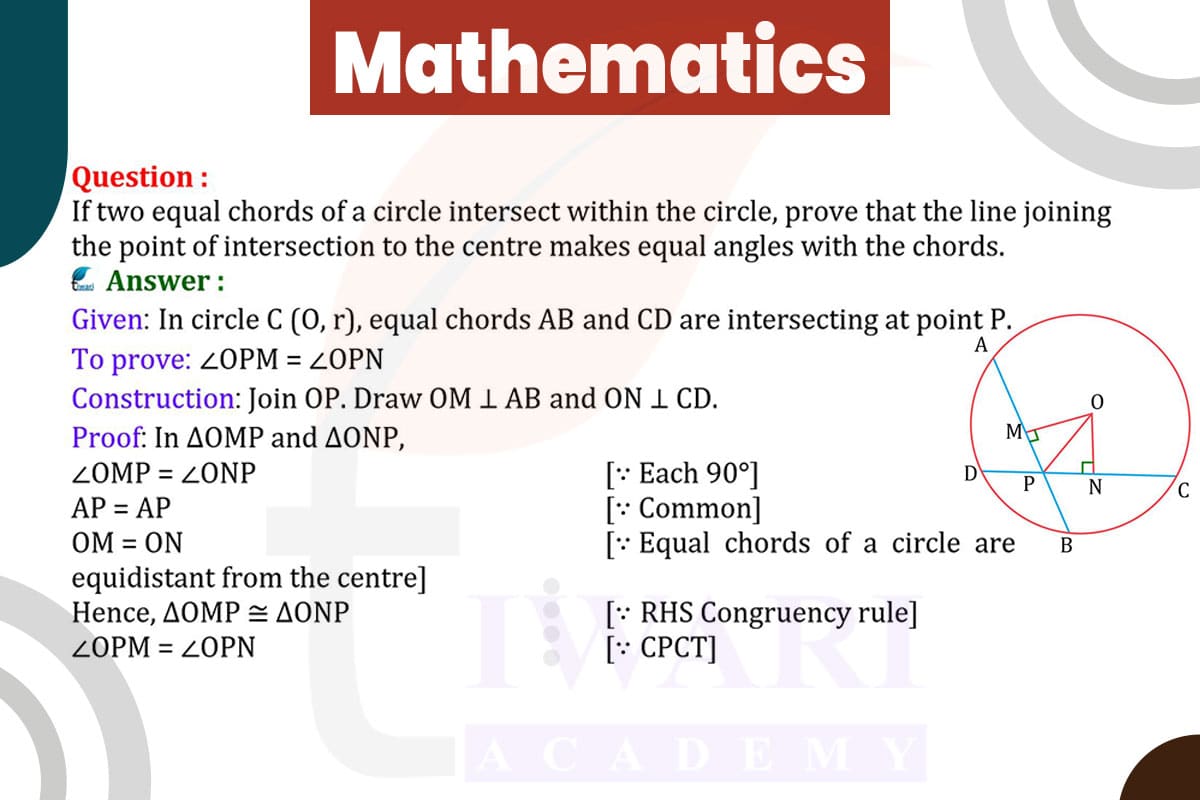
To prove that if two equal chords of a circle intersect within the circle, the line joining the point of intersection to the center makes equal angles with the chords, consider two equal chords AB and CD intersecting at point E. Draw a line from E to the center O of the circle. Triangles OEA and OEC are formed. Since AB and CD are equal, triangles OEA and OEC are congruent by the Side-Side-Side (SSS) criterion (OE is common, and radii OA and OC are equal). Therefore, angles OEA and OEC are equal, as they are corresponding angles of congruent triangles.
Let’s discuss in detail
Geometric Properties of Chords
In circle geometry, the study of chords and their properties reveals fascinating insights. A chord is a line segment with both endpoints on the circle’s circumference. A unique geometric scenario arises when two equal chords intersect within a circle. This intersection creates an opportunity to explore the angles formed by a line drawn from the intersection point to the circle’s center. The question we address is whether this line makes equal angles with the chords, a query that delves into the symmetry and properties inherent in circular shapes.
Concept of Equal Chords in a Circle
When we talk about equal chords in a circle, we refer to chords that have the same length. The intersection of two such chords within a circle forms a point that is equidistant from the endpoints of each chord. This point of intersection is crucial in our exploration, as it forms the vertex of the angles we are interested in examining. The symmetry suggested by the equality of the chords hints at potential equal angles formed with a line drawn to the circle’s center.
Formation of Triangles by Intersecting Chords
Consider two equal chords, AB and CD, intersecting at point E within the circle. Drawing a line from E to the center of the circle, O, forms two triangles: OEA and OEC. These triangles are key to our proof, as they contain the angles formed by the line OE with each chord. The properties of these triangles, given the equality of the chords, lead us to the heart of the proof.
Applying the Side-Side-Side (SSS) Criterion for Congruence
To establish the congruence of triangles OEA and OEC, we use the Side-Side-Side (SSS) criterion. This criterion states that if three sides of one triangle are equal to three sides of another triangle, the triangles are congruent. In our case, sides OA and OC are equal (being radii of the circle), side OE is common to both triangles, and chords AB and CD are equal. This congruence is the foundation for proving the equality of the angles in question.
Proving the Equality of Angles
With the congruence of triangles OEA and OEC established, it follows that corresponding parts of congruent triangles are equal. This includes the angles ∠OEA and ∠OEC. These angles are formed by the line OE with the chords AB and CD, respectively. Therefore, the line joining the point of intersection to the center of the circle makes equal angles with the chords. This conclusion is a direct result of the congruence of the triangles formed by the intersecting chords and the center of the circle.
Symmetry in Circle Geometry
In conclusion, this proof demonstrates the elegant symmetry inherent in circle geometry. The fact that a line from the intersection point of two equal chords to the center forms equal angles with the chords highlights the balanced and harmonious properties of circles. Such geometric principles not only provide aesthetic satisfaction but also have practical implications in various fields, from engineering to design. Understanding these relationships deepens our appreciation of geometry’s role in explaining the world around us.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.2 in Detail

