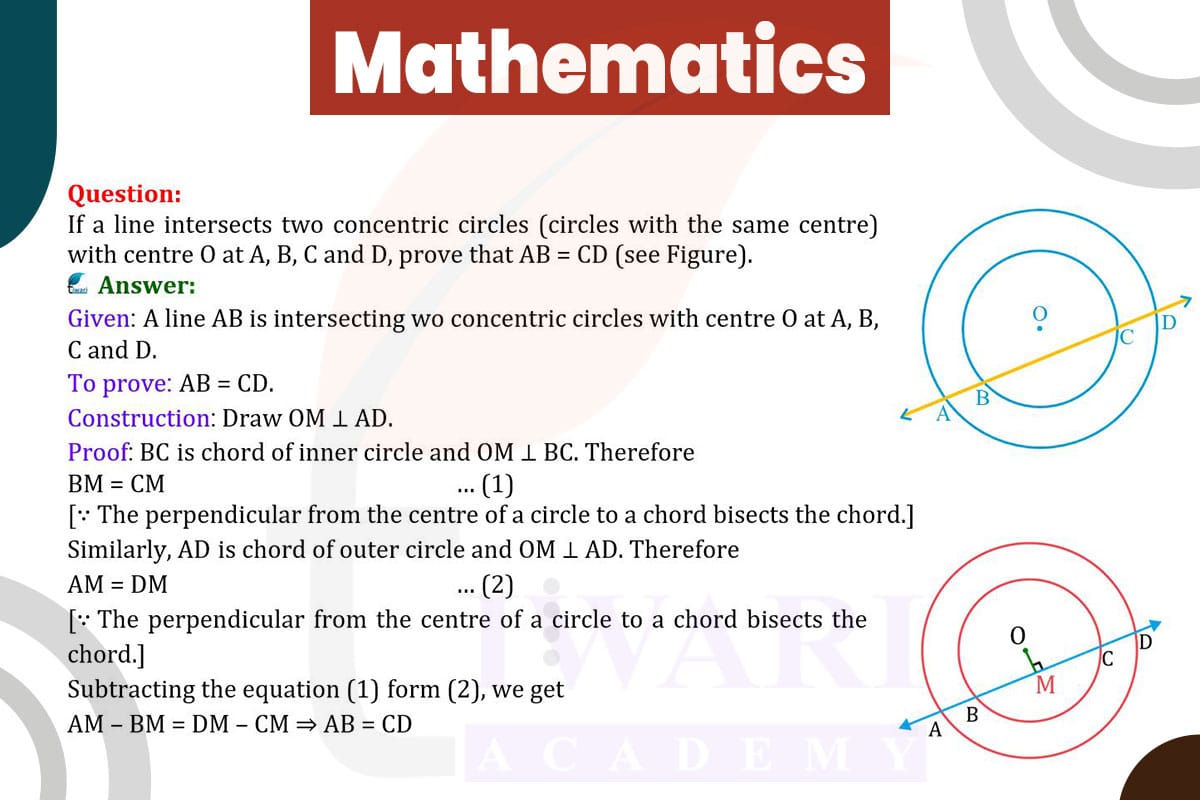
To prove that AB = CD when a line intersects two concentric circles (circles with the same center O) at points A, B, C, and D, consider the line segment OC. Since O is the center of both circles, OC is a radius of the outer circle and OD is a radius of the inner circle. The line intersects the circles at A and B on the outer circle, and at C and D on the inner circle, with A and D being the farthest points from O. Since OA = OC (radii of the outer circle) and OB = OD (radii of the inner circle), and OC = OD (both are the same line segment), triangles OAB and OCD are congruent by the Side-Side-Side (SSS) criterion. Therefore, AB = CD as they are corresponding parts of congruent triangles.
Let’s discuss in detail
Concentric Circles and Line Intersections
In the study of circle geometry, concentric circles, which are circles with a common center, present unique properties. A line intersecting two concentric circles creates specific points of intersection that are of interest in geometric proofs. When such a line intersects two concentric circles, it typically touches each circle at two points. If we label these points as A, B on the outer circle and C, D on the inner circle, a question arises: are the segments AB and CD equal? This scenario provides a fascinating glimpse into the symmetrical properties of concentric circles.
Understanding the Geometry of Concentric Circles
Concentric circles share the same center, denoted as point O in our case. This shared center is a pivotal element in understanding the relationship between the line segments formed by the line’s intersection with the circles. The radii of these circles, although different in length, emanate from the same central point, creating a basis for exploring geometric relationships between the points of intersection A, B, C, and D.
Formation of Line Segments by Intersection
When a line intersects the two concentric circles, it forms two distinct line segments: AB on the outer circle and CD on the inner circle. The positioning of these segments is crucial to our proof. Points A and D are the farthest from the center O, while B and C are closer to O. This arrangement forms the basis for comparing the lengths of AB and CD.
Constructing Triangles and Applying the SSS Criterion
To prove that AB equals CD, we consider triangles OAB and OCD. In these triangles, OA and OC are radii of the outer circle, and OB and OD are radii of the inner circle. Since OA = OC and OB = OD (as they are radii of concentric circles), and OC and OD are the same line segment, triangles OAB and OCD are congruent by the Side-Side-Side (SSS) criterion. This congruence is key to establishing the equality of AB and CD.
Proving the Equality of AB and CD
With the congruence of triangles OAB and OCD established, it follows that corresponding parts of congruent triangles are equal. This includes the line segments AB and CD, which are corresponding parts of these triangles. Therefore, AB is equal to CD. This equality is a direct result of the congruence of the triangles formed by the line intersecting the concentric circles.
Symmetry in Concentric Circle Geometry
In conclusion, this proof illustrates a beautiful aspect of symmetry in concentric circle geometry. The fact that a line intersecting two concentric circles at four points results in two line segments of equal length highlights the inherent geometric harmony. Such properties are not only aesthetically pleasing but also fundamental to understanding complex geometric relationships. This proof exemplifies how basic geometric principles can lead to profound insights into the nature of shapes and their interrelations.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.2 in Detail

