To find the length of the string of each toy telephone when three boys, Ankur, Syed, and David, are sitting at equal distances on the boundary of a circular park with a radius of 20 meters, we can consider the circle and the positions of the boys. Since they are at equal distances from each other, they form an equilateral triangle inscribed in the circle.
The distance between any two boys is a side of this equilateral triangle. The radius of the circle is the segment from the center to a vertex of the triangle. Using the properties of an equilateral triangle and a circle, we can calculate the side length of the triangle.
For an equilateral triangle inscribed in a circle, the relationship between the side of the triangle (s) and the radius of the circle (R) is given by s = √3 × R. Therefore, for a radius of 20 meters, the length of each side of the triangle (the length of the string of each phone) is √3 × 20 meters, which is approximately 34.64 meters.
Let’s discuss in detail
Introduction to the Geometric Scenario
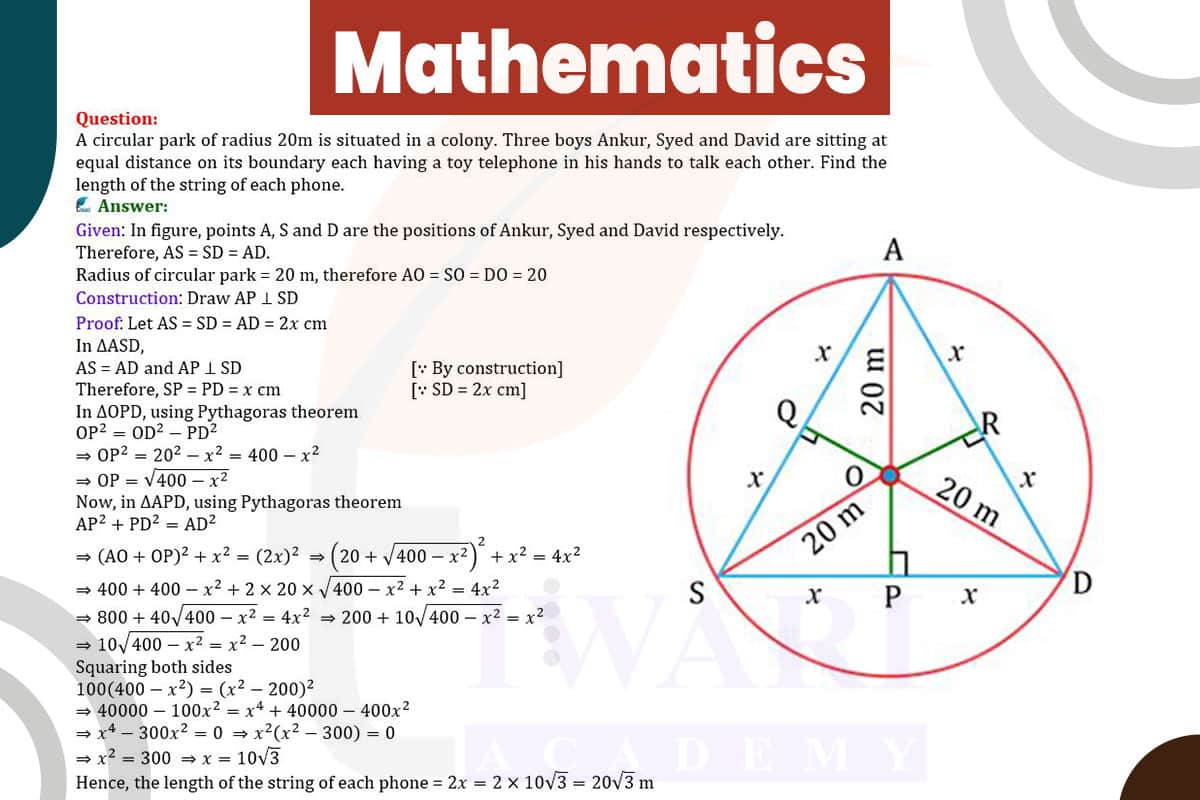
In a circular park with a radius of 20 meters, a unique geometric scenario is presented with three boys, Ankur, Syed, and David, sitting at equal distances on its boundary. Each boy is equipped with a toy telephone connected by a string, and they are positioned in such a way that they can communicate with each other. The task is to determine the length of the string of each toy telephone. This problem is not just a practical application of geometry but also an interesting exploration of how geometric principles apply to everyday situations.
The Circular Park and Equidistant Points
The park is circular, with a radius of 20 meters. When three points (where the boys are sitting) are equidistant on the boundary of a circle, they form the vertices of an equilateral triangle. This triangle is inscribed within the circle, meaning all its vertices touch the circle’s circumference. The significance of this arrangement is that it creates a perfect geometric shape, allowing for precise calculations and symmetry.
Formation of an Equilateral Triangle
The positions of Ankur, Syed, and David form an equilateral triangle, where each side represents the distance between two boys. In an equilateral triangle, all sides are of equal length, and all angles are equal, each measuring 60 degrees. This uniformity is key to determining the length of the string connecting the toy telephones, as it simplifies the calculation by providing a consistent measure across all sides of the triangle.
Relationship Between the Circle and the Triangle
The relationship between the radius of the circle and the side of the equilateral triangle inscribed within it is governed by specific geometric principles. For an equilateral triangle inscribed in a circle, the length of each side of the triangle can be calculated using the radius of the circle. This relationship is crucial in solving our problem, as it directly links the known radius of the park to the unknown length of the string.
Calculating the Length of the String
To calculate the length of the string, we use the formula that relates the side of an equilateral triangle to the radius of its circumscribed circle: s = √3 × R, where s is the side of the triangle and R is the radius of the circle. Substituting 20 meters for R, we find that the length of each side of the triangle, and thus the length of the string of each toy telephone, is √3 × 20 meters, approximately 34.64 meters.
Applying Geometry to Real-World Problems
In conclusion, this problem demonstrates the practical application of geometric principles to real-world scenarios. By understanding the relationship between the elements of a circle and an equilateral triangle, we can solve problems that, at first glance, might seem complex. The ability to apply geometry in such contexts not only provides solutions to specific problems but also enriches our understanding of the spatial relationships in the world around us.
Discuss this question in detail or visit to Class 9 Maths Chapter 9 for all questions.
Questions of 9th Maths Exercise 9.2 in Detail

