ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the

Updated on November 25, 2023
by Tiwari Academy

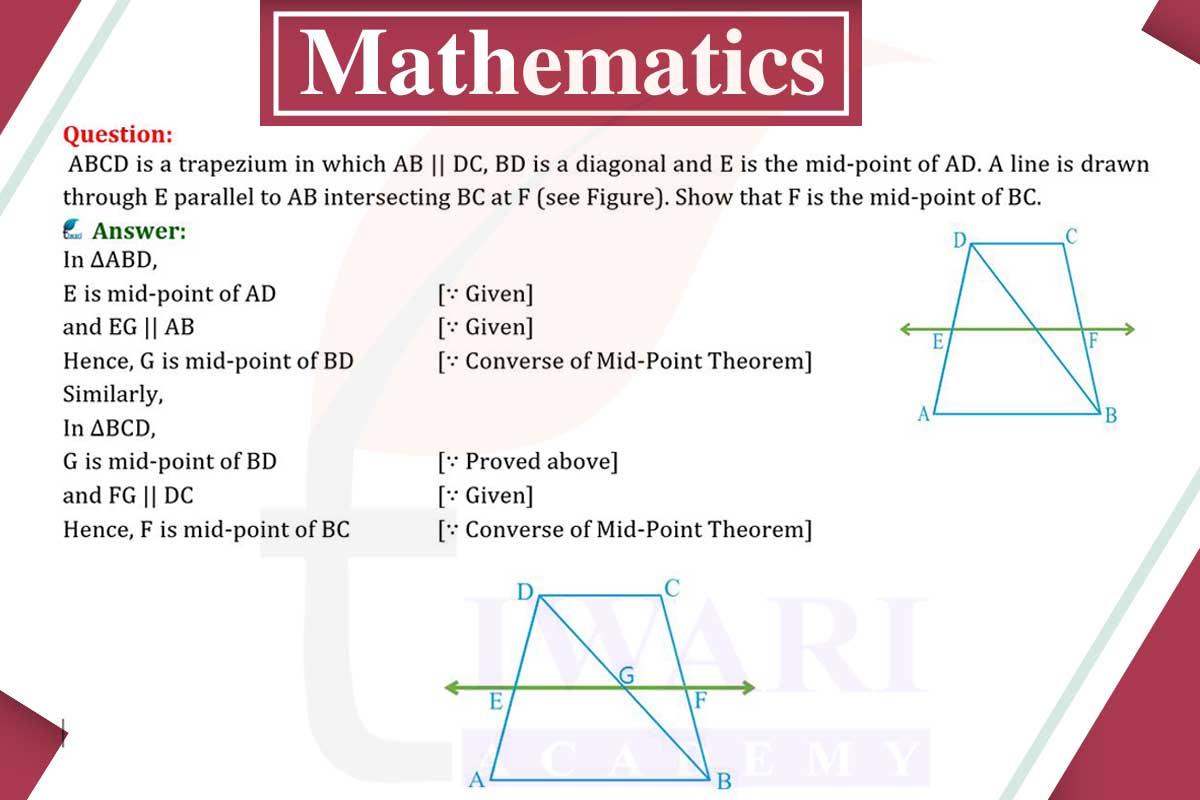
In trapezium ABCD, where AB is parallel to DC, BD is a diagonal, and E is the midpoint of AD:
Triangles AED and CFD are Similar: Since E is the midpoint of AD, AE = ED. The line through E parallel to AB (and DC) means ∠AED = ∠CFD (alternate interior angles) and ∠DAE = ∠DCF (corresponding angles). Thus, ΔAED ∼ ΔCFD (by AA similarity).
Proportionality in Similar Triangles: In similar triangles, corresponding sides are proportional. Since AE = ED, the corresponding sides AF and FC in ΔAED and ΔCFD are equal.
Therefore, F is the midpoint of BC, as AF = FC.

ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F. Show that F is mid point of BC.

Let’s discuss in detail

Trapezium ABCD and Its Geometric Configuration

In the trapezium ABCD, where AB is parallel to DC, and BD serves as a diagonal, a unique geometric scenario is presented. The addition of point E as the midpoint of AD introduces an interesting aspect to this configuration. When a line is drawn through E parallel to AB (and consequently DC, due to the properties of a trapezium), intersecting BC at point F, it creates a set of parallel lines and triangles within the trapezium. This setup is ripe for the application of geometric theorems and properties, particularly those related to parallel lines, similar triangles, and proportions.

Establishing the Similarity of Triangles AED and CFD

The key to solving this problem lies in proving the similarity of triangles AED and CFD. Since E is the midpoint of AD, AE and ED are equal in length. The line through E parallel to AB and DC creates alternate interior angles ∠AED and ∠CFD, and corresponding angles ∠DAE and ∠DCF, which are equal. According to the Angle-Angle (AA) criterion for similarity of triangles, ΔAED is similar to ΔCFD. This similarity is crucial for establishing the proportional relationships between the sides of these triangles.

Proportional Relationships in Similar Triangles

In similar triangles, corresponding sides are in proportion. This means that the ratios of corresponding sides of similar triangles are equal. For triangles AED and CFD, which have been established as similar, this implies that the ratio of AE to ED is equal to the ratio of AF to FC. Since AE equals ED (as E is the midpoint of AD), the proportionality principle dictates that AF must equal FC. This proportional relationship is the cornerstone of proving that F is the midpoint of BC.

The Role of Parallel Lines in Establishing Midpoints
The parallel lines AB and DC, and the line through E parallel to these lines, play a significant role in this geometric configuration. Parallel lines have the property of creating equal alternate interior and corresponding angles when intersected by a transversal. In this case, the line through E acts as a transversal, creating equal angles in triangles AED and CFD. This equality of angles leads to the similarity of these triangles, which in turn leads to the proportional relationships between their sides.

Concluding F as the Midpoint of BC

Given the similarity of triangles AED and CFD and the proportional relationship of their sides, it can be concluded that F, the point of intersection on BC, is indeed its midpoint. The equality of AF and FC is a direct result of the equal lengths of AE and ED and the properties of similar triangles. This conclusion not only solves the problem but also illustrates the elegance and interconnectedness of geometric principles, particularly in the context of trapeziums and similar triangles.

Geometric Principles in Trapezium ABCD

In summary, the trapezium ABCD, with its specific geometric properties and the addition of points E and F, demonstrates the beauty of geometry. The problem showcases how the concepts of similar triangles, proportional sides, and parallel lines come together to reveal significant properties within a shape. The conclusion that F is the midpoint of BC is a testament to the power of geometric reasoning and the depth of relationships that can exist within seemingly simple geometric figures.

Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.2 in Detail

ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA. AC is a diagonal. Show that: (i) SR || AC and SR = 1/2AC (ii) PQ = SR (iii) PQRS is a parallelogram.

ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.

ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.

ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F. Show that F is the mid-point of BC.

In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively. Show that the line segments AF and EC trisect the diagonal BD.

ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that (i) D is the mid-point of AC (ii) MD⊥AC (iii) CM = MA = 1/2AB.