ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB?

Updated on November 25, 2023
by Tiwari Academy

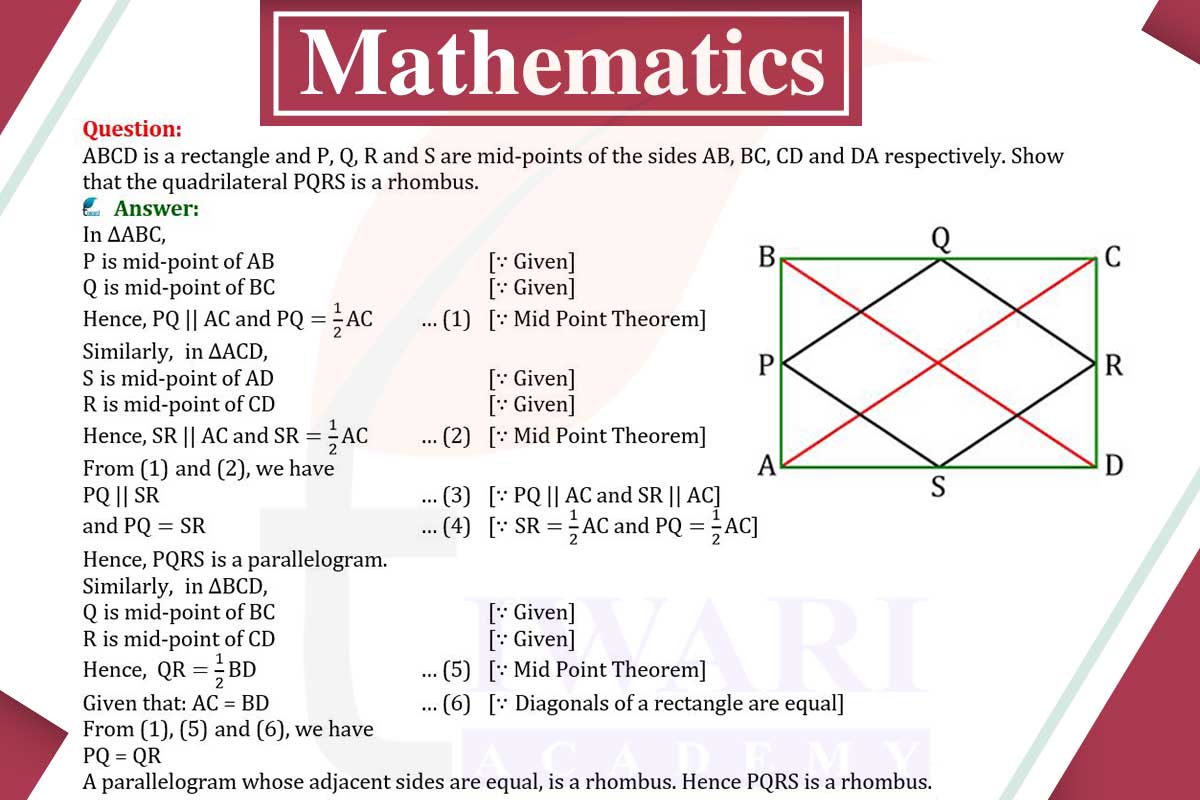
In rectangle ABCD, with P, Q, R, and S as mid-points of sides AB, BC, CD, and DA respectively:
PQRS is a Parallelogram: By the Midpoint Theorem, PQ and SR are parallel and equal as they join midpoints of consecutive sides of the rectangle. Similarly, PS and QR are parallel and equal. Thus, PQRS is a parallelogram.
Sides of PQRS are Equal: In a rectangle, opposite sides are equal. Since P, Q, R, and S are midpoints, each side of PQRS is half the length of a side of ABCD, making all sides of PQRS equal.
Therefore, PQRS is a parallelogram with all sides equal, making it a rhombus.

ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that quadrilateral PQRS is a rhombus.

Let’s discuss in detail

Introduction to Rectangle ABCD and Midpoints

In the rectangle ABCD, points P, Q, R, and S are designated as the midpoints of the sides AB, BC, CD, and DA, respectively. A rectangle is characterized by opposite sides being equal and angles being right angles. The selection of midpoints on the sides of a rectangle creates a specific geometric configuration that leads to intriguing properties in the quadrilateral formed by connecting these midpoints. This setup in rectangle ABCD allows for an exploration of the properties of the quadrilateral PQRS, revealing insights into its nature based on the characteristics of the rectangle.

Establishing PQRS as a Parallelogram

The first step is to establish that PQRS is a parallelogram. According to the Midpoint Theorem, a line segment joining the midpoints of two sides of a triangle is parallel to the third side and half its length. In the context of rectangle ABCD, this theorem implies that PQ is parallel to SR and PS is parallel to QR, as they join the midpoints of consecutive sides. Since opposite sides of PQRS are parallel, it satisfies the definition of a parallelogram.

Equal Lengths of Opposite Sides in PQRS

In addition to being parallel, the opposite sides of PQRS are also equal in length. This equality arises because, in a rectangle, opposite sides are equal, and midpoints divide these sides into segments of equal length. Therefore, the lengths of PQ and SR, and PS and QR, are equal, as each is half the length of the sides of rectangle ABCD. This characteristic further confirms that PQRS is a parallelogram.

All Sides of PQRS are Equal
A defining feature of a rhombus is that all its sides are equal. In PQRS, each side is half the length of a side of rectangle ABCD. Since the rectangle has opposite sides equal, all sides of PQRS are equal in length. This equality of all sides is a crucial step in identifying the nature of quadrilateral PQRS.

PQRS as a Rhombus

Given that PQRS is a parallelogram with all sides equal, it meets the definition of a rhombus. A rhombus is essentially a parallelogram with equal sides, and since PQRS has been shown to have both these properties, it can be conclusively stated that PQRS is a rhombus. This conclusion is a direct result of the properties of the rectangle ABCD and the specific positioning of the midpoints on its sides.

Geometric Nature of PQRS

In conclusion, the quadrilateral PQRS, formed by connecting the midpoints of the sides of rectangle ABCD, is a rhombus. This is established through the application of the Midpoint Theorem and the inherent properties of a rectangle, specifically the equality of opposite sides. The exercise demonstrates how specific configurations within a geometric figure can lead to the formation of another figure with its own distinct properties, showcasing the interconnectedness and elegance of geometric principles.

Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.2 in Detail

ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA. AC is a diagonal. Show that: (i) SR || AC and SR = 1/2AC (ii) PQ = SR (iii) PQRS is a parallelogram.

ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.

ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.

ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F. Show that F is the mid-point of BC.

In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively. Show that the line segments AF and EC trisect the diagonal BD.

ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that (i) D is the mid-point of AC (ii) MD⊥AC (iii) CM = MA = 1/2AB.