ABCD is a quadrilateral in which P, Q, R and S are mid-points of the

Updated on November 25, 2023
by Tiwari Academy

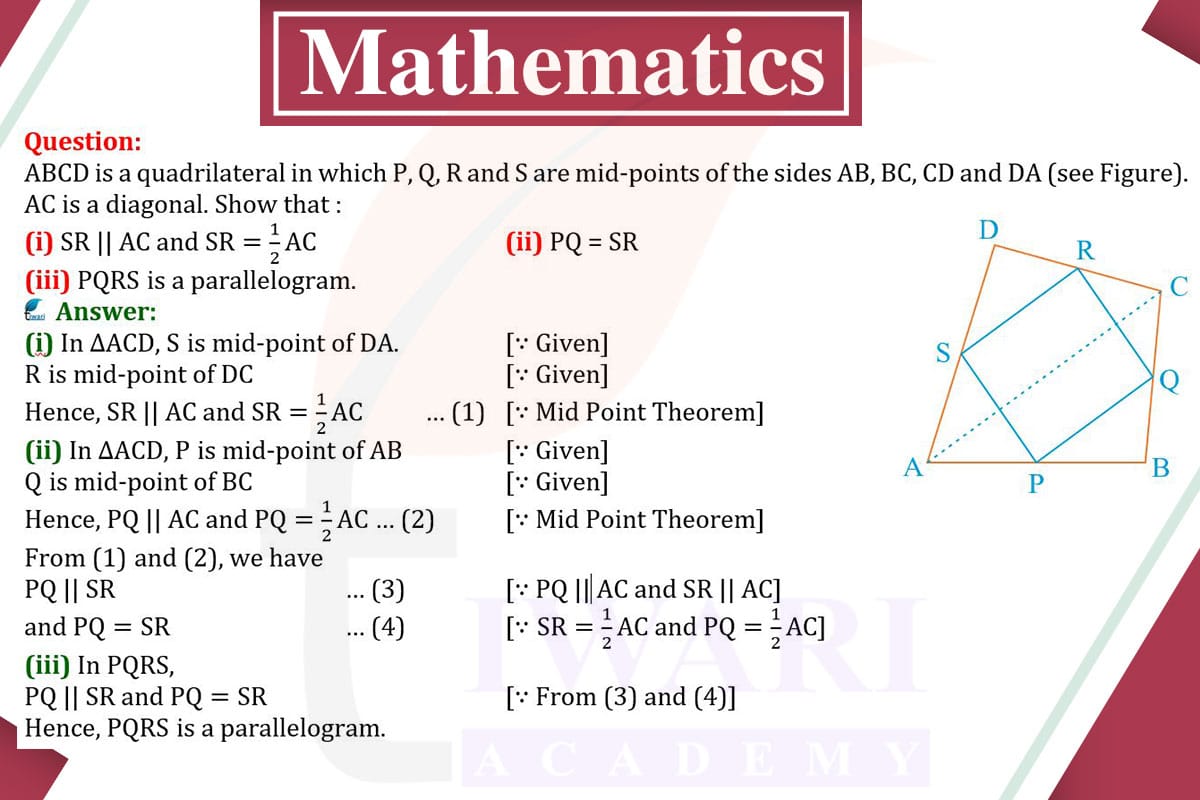
In quadrilateral ABCD, with P, Q, R, and S as mid-points of sides AB, BC, CD, and DA respectively, and AC as a diagonal:
(i) SR || AC and SR = 1/2 AC: By the Midpoint Theorem, a line segment joining the midpoints of two sides of a triangle is parallel to the third side and half its length. In ΔACD, S and R are midpoints of AD and CD, so SR || AC and SR = 1/2 AC.
(ii) PQ = SR: Similarly, in ΔABC, P and Q are midpoints of AB and BC, so PQ || AC and PQ = 1/2 AC. Since SR = 1/2 AC, PQ = SR.
(iii) PQRS is a Parallelogram: PQ and SR are both parallel and equal in length. Also, PQ || SR (both parallel to AC). Therefore, PQRS has both pairs of opposite sides parallel and equal, making it a parallelogram.

ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA. AC is a diagonal. Show that: (i) SR || AC and SR = 1/2AC (ii) PQ = SR

Let’s discuss in detail

Quadrilateral ABCD and Midpoints

In the quadrilateral ABCD, consider points P, Q, R, and S, which are the midpoints of sides AB, BC, CD, and DA, respectively. The diagonal AC divides the quadrilateral into two triangles, ΔABC and ΔACD. The positioning of these midpoints and the diagonal creates a unique geometric scenario, allowing for the application of the Midpoint Theorem. This theorem plays a crucial role in understanding the relationships between the line segments formed by these midpoints and the sides of the quadrilateral, leading to significant conclusions about the nature of the figure formed by connecting these midpoints.

Properties of Line Segment SR in ΔACD

In triangle ΔACD, S and R are the midpoints of sides AD and CD, respectively. According to the Midpoint Theorem, a line segment joining the midpoints of two sides of a triangle is parallel to the third side and half its length. Applying this theorem to ΔACD, it can be deduced that line segment SR is parallel to AC and that its length is half that of AC. This property of SR being parallel and proportionally shorter than AC is fundamental to establishing the geometric nature of the figure formed by P, Q, R, and S.

Properties of Line Segment PQ in ΔABC

Similarly, in triangle ΔABC, where P and Q are the midpoints of AB and BC, respectively, the Midpoint Theorem can be applied. This theorem dictates that PQ will be parallel to the third side AC and that its length will be half of AC. This parallelism and proportional relationship between PQ and AC mirror the properties of SR in ΔACD, suggesting a symmetry in the quadrilateral ABCD that is not immediately apparent but is revealed through the analysis of these midpoints.

Equality of PQ and SR
Since both PQ and SR are parallel to AC and each is half the length of AC, it follows that PQ is equal in length to SR. This equality is a direct consequence of the proportional relationships established by the Midpoint Theorem in both ΔABC and ΔACD. The fact that these two line segments are not only parallel to the same diagonal but also equal in length is a significant geometric finding, indicating a level of symmetry and congruence within the quadrilateral ABCD.

PQRS as a Parallelogram

Given that PQ and SR are both parallel and equal in length, and considering that PQ is parallel to SR (as both are parallel to AC), it can be concluded that PQRS is a parallelogram. In a quadrilateral, if both pairs of opposite sides are equal and parallel, the figure is a parallelogram. Therefore, the properties of PQ and SR, derived from their positions as midpoints and the application of the Midpoint Theorem, are sufficient to establish that PQRS is a parallelogram.

Geometric Nature of PQRS

In conclusion, the quadrilateral formed by connecting the midpoints P, Q, R, and S of the sides of ABCD exhibits the properties of a parallelogram. This conclusion is reached through the application of the Midpoint Theorem to the triangles formed by the diagonal AC, leading to the findings that SR is parallel and equal to half of AC, PQ is equal to SR, and both are parallel. These properties demonstrate that PQRS is a parallelogram, showcasing the fascinating ways in which geometric principles can reveal hidden structures and symmetries in a seemingly complex figure like a quadrilateral.

Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.2 in Detail

ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA. AC is a diagonal. Show that: (i) SR || AC and SR = 1/2AC (ii) PQ = SR (iii) PQRS is a parallelogram.

ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.

ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.

ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F. Show that F is the mid-point of BC.

In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively. Show that the line segments AF and EC trisect the diagonal BD.

ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that (i) D is the mid-point of AC (ii) MD⊥AC (iii) CM = MA = 1/2AB.