ABC is a triangle right angled at C. A line through the mid-point M

Updated on November 25, 2023
by Tiwari Academy

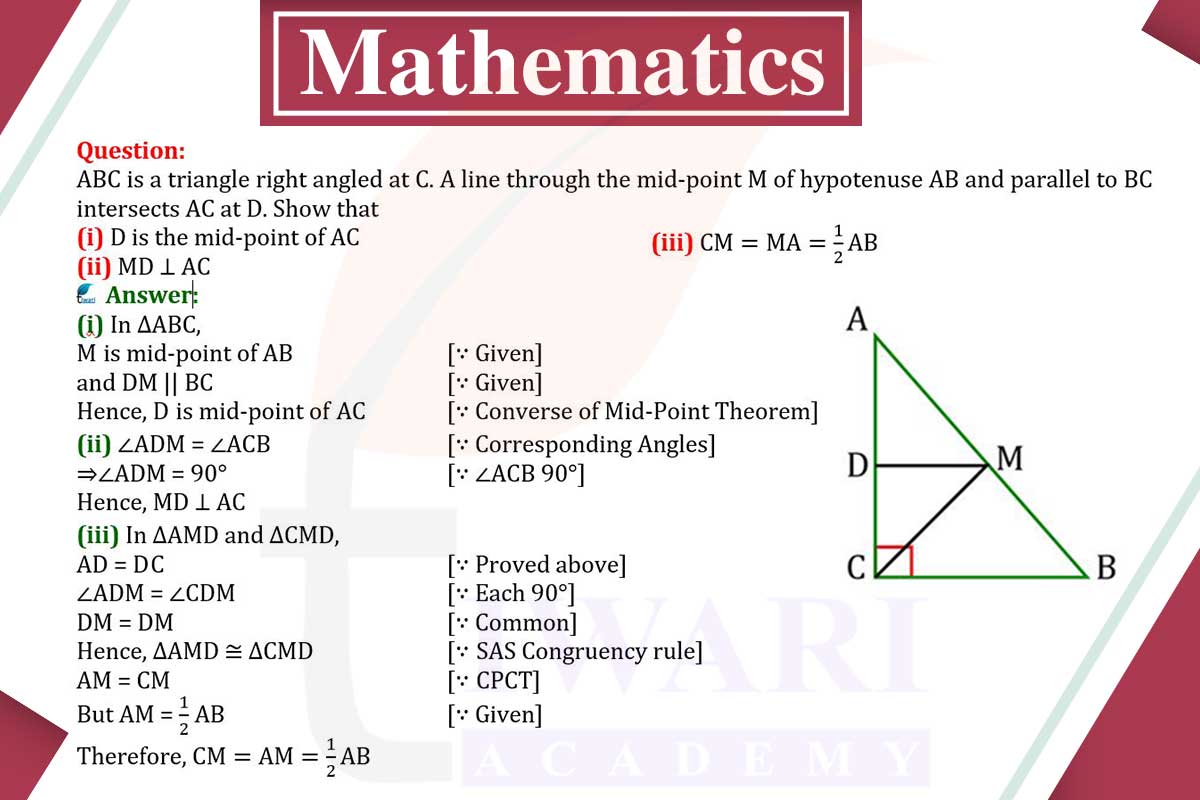
In right-angled triangle ABC, with the right angle at C and M as the midpoint of the hypotenuse AB:
D is the Mid-point of AC: Since M is the midpoint of AB, MD is parallel to BC and intersects AC. By the Converse of the Midpoint Theorem, D must be the midpoint of AC.
MD ⊥ AC: In a right-angled triangle, the median from the right angle to the hypotenuse is half the hypotenuse and perpendicular to it. Since M is the midpoint of AB, MD is this median, so MD is perpendicular to AC.
CM = MA = 1/2AB: In a right-angled triangle, the median to the hypotenuse (MD) is half the hypotenuse. Since M is the midpoint of AB, CM and MA are each half of AB, i.e., CM = MA = 1/2AB.

ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that (i) D is the mid-point of AC (ii) MD ⊥ AC

Let’s discuss in detail

Right-Angled Triangle ABC and Midpoint M

In the right-angled triangle ABC, where the right angle is at C, and M is the midpoint of the hypotenuse AB, a unique geometric scenario unfolds. The properties of right-angled triangles, combined with the concept of midpoints and parallel lines, create a foundation for exploring several interesting geometric relationships. The line drawn through M parallel to BC, intersecting AC at D, sets the stage for demonstrating specific properties related to midpoints, perpendicularity, and lengths within the triangle.

Establishing D as the Midpoint of AC

The first property to establish is that D is the midpoint of AC. This is derived from the Converse of the Midpoint Theorem, which states that if a line is drawn through the midpoint of one side of a triangle and is parallel to another side, it will intersect the third side at its midpoint. Since M is the midpoint of AB and MD is parallel to BC, D must be the midpoint of AC. This relationship is a fundamental aspect of the geometry of triangles and is crucial for understanding the subsequent properties of the triangle.

Perpendicularity of MD to AC

The second property to demonstrate is that MD is perpendicular to AC. In a right-angled triangle, the median from the right angle to the hypotenuse has a special property: it is half the length of the hypotenuse and is perpendicular to it. Since M is the midpoint of the hypotenuse AB, MD is this median. Therefore, MD is perpendicular to AC, a property that is characteristic of right-angled triangles and plays a significant role in their geometric properties.

Equality of CM, MA, and Half of AB
The third property involves the lengths of CM, MA, and their relationship to the hypotenuse AB. In a right-angled triangle, the length of the median to the hypotenuse (MD in this case) is equal to half the length of the hypotenuse. Since M is the midpoint of AB, the segments CM and MA are each half of the length of AB. Therefore, CM equals MA, and both are equal to half the length of AB. This equality is a direct consequence of the properties of medians in right-angled triangles.

Implications of These Geometric Properties

The implications of these geometric properties in triangle ABC are significant. They demonstrate the harmony and balance inherent in right-angled triangles. The perpendicularity of the median to the hypotenuse and the equal division of the sides and hypotenuse highlight the symmetry and precise geometric relationships within right-angled triangles. These properties are not just specific to triangle ABC but are general characteristics of all right-angled triangles, showcasing the elegance and consistency of geometric principles.

Geometric Harmony in Right-Angled Triangle ABC

In conclusion, the right-angled triangle ABC, with its specific construction involving the midpoint M of the hypotenuse AB and a line MD parallel to BC, exhibits fascinating geometric properties. D being the midpoint of AC, MD being perpendicular to AC, and the equal lengths of CM, MA, and half of AB, all illustrate the interconnectedness and elegance of geometric principles. This example underscores the depth and beauty of geometry, where simple constructions can lead to profound and elegant results, demonstrating the intricate relationships and symmetries within geometric figures.

Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.2 in Detail

ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA. AC is a diagonal. Show that: (i) SR || AC and SR = 1/2AC (ii) PQ = SR (iii) PQRS is a parallelogram.

ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.

ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.

ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F. Show that F is the mid-point of BC.

In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively. Show that the line segments AF and EC trisect the diagonal BD.

ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that (i) D is the mid-point of AC (ii) MD⊥AC (iii) CM = MA = 1/2AB.