CBSE NCERT Solutions for Class 9 Maths Chapter 8 Exercise 8.2 Quadrilaterals in Hindi and English Medium for new session 2025-26. Get here revised solutions of ex. 8.2 class 9th math updated according the revised syllabus and NCERT books for CBSE 2025-26.
NCERT Solutions for Class 9 Maths Exercise 8.2 in Hindi and English Medium
| Class: 9 | Mathematics |
| Chapter: 8 | Exercise: 8.2 |
| Topic Name: | Quadrilaterals |
| Content: | Textbook solution and Extra Questions |
| Content Mode: | Text, Videos and PDF |
| Academic Session: | CBSE 2025-26 |
| Medium: | English and Hindi Medium |
Class 9 Maths Exercise 8.2 Questions in Detail
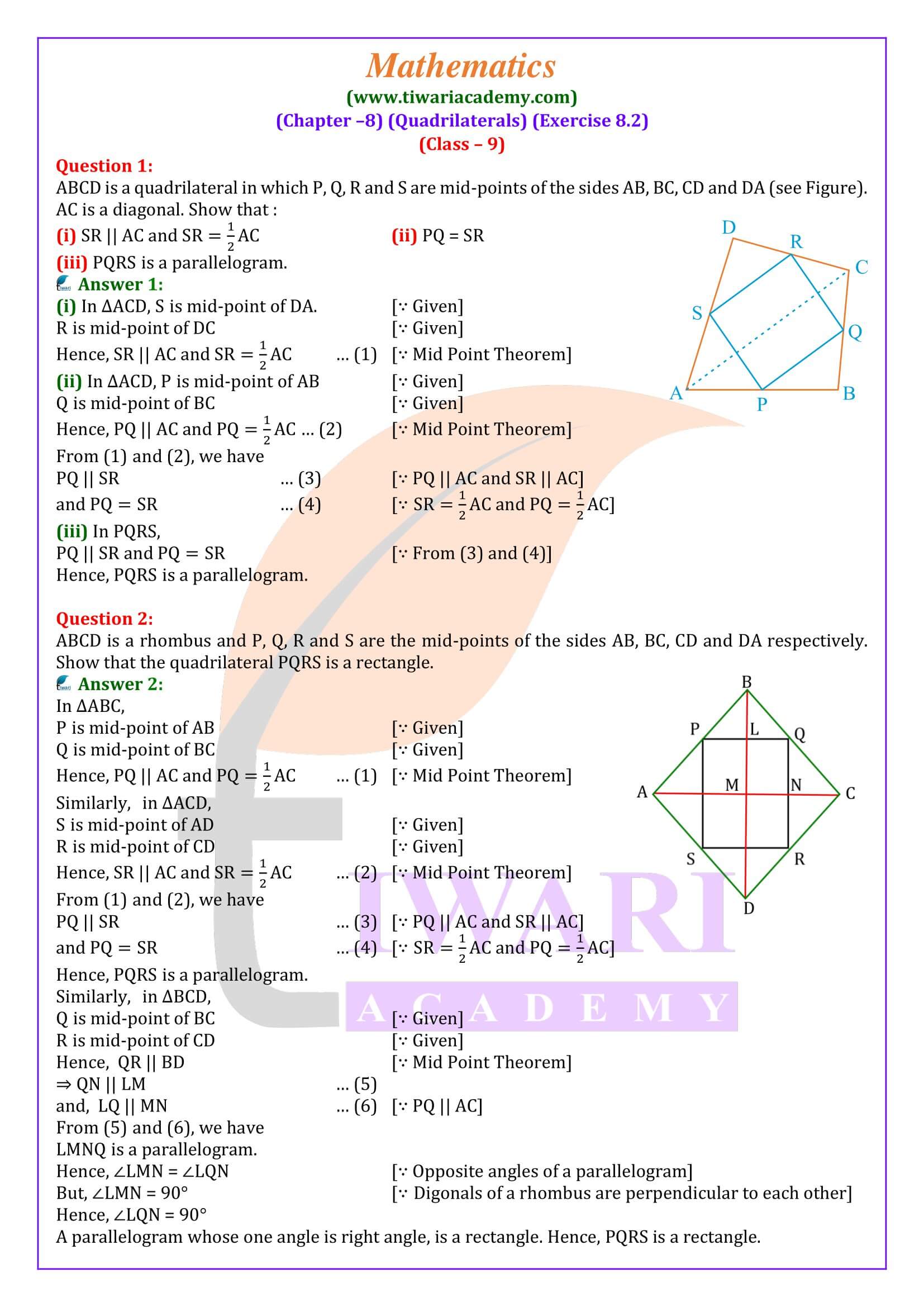
- ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA. AC is a diagonal. Show that: (i) SR || AC and SR = 1/2AC (ii) PQ = SR (iii) PQRS is a parallelogram.
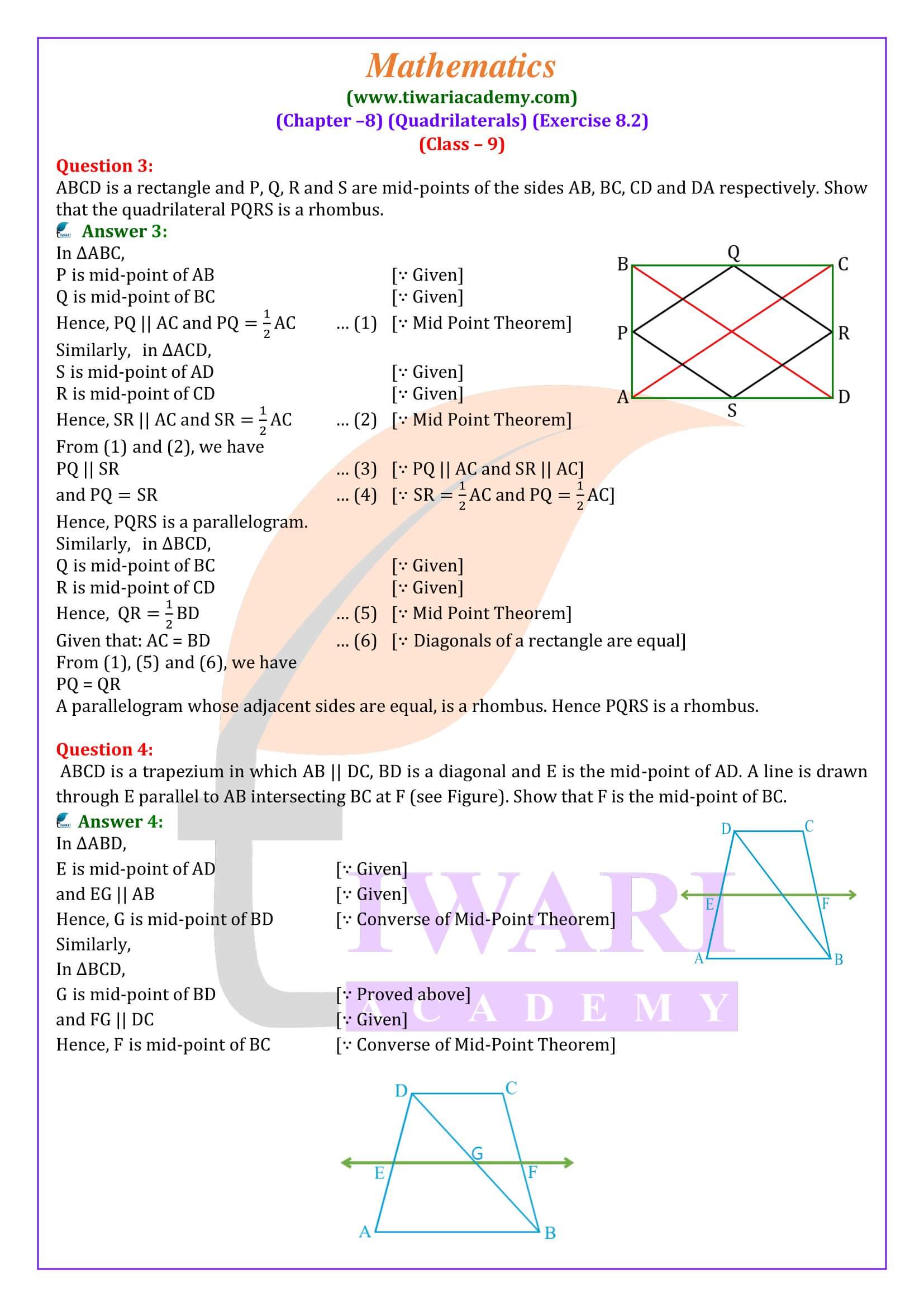
- ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
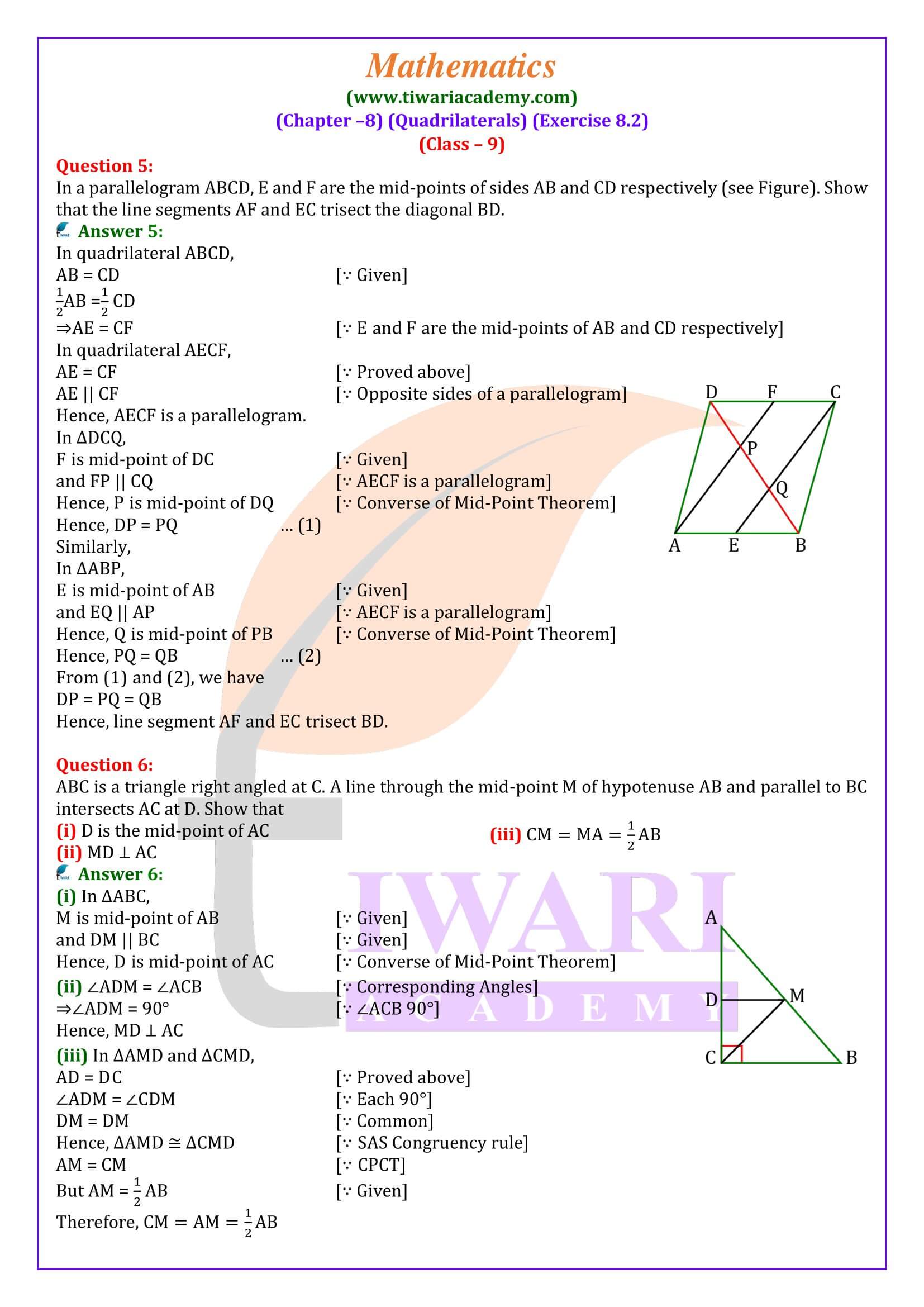
- ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
- ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F. Show that F is the mid-point of BC.
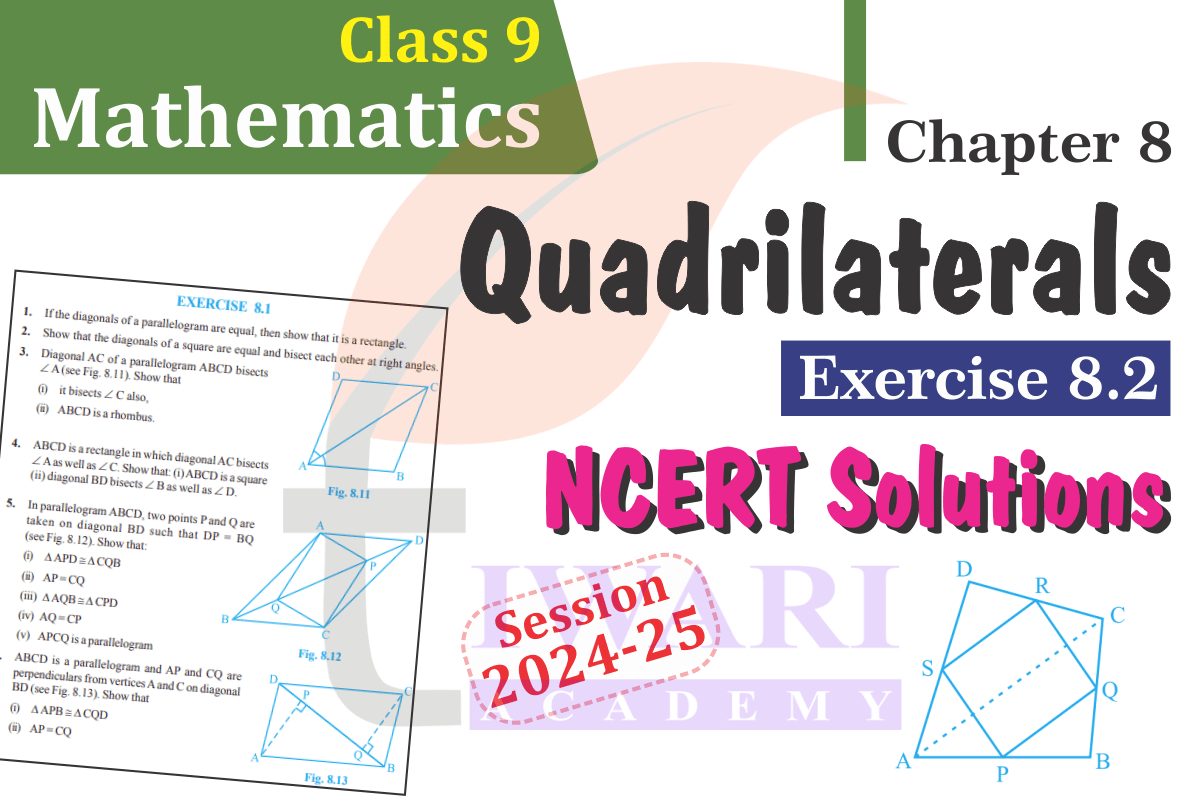
- In a parallelogram ABCD, E and F are the mid-points of sides AB and CD respectively. Show that the line segments AF and EC trisect the diagonal BD.
- ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that (i) D is the mid-point of AC (ii) MD⊥AC (iii) CM = MA = 1/2AB.
Class 9 Maths Exercise 8.2 Solution in Hindi and English Medium
Uttar Pradesh board high school students can also use these solutions as UP Board Solutions for Class 9 Maths Chapter 8. All the contents are updated for the new academic session 2025-26 in PDF as well as Videos format. Videos related to ex. 8.2 in Hindi and English language are given here free to use in simplified language.
Class 9 Maths Exercise 8.2 Solution in Hindi Medium Video
About Class 9 Maths Chapter 8 Quadrilaterals
We have seen that in a parallel quadrilateral, each pair of opposite sides is equal. Further, we also know that if each pair of opposite sides of the quadrilateral is equal, then it is a parallel quadrilateral. We can conclude the same result for couples of opposite angles that in the parallelogram, the opposite angles are the same.
We have studied many properties of a parallel quadrilateral in Grade 9 Math Chapter 8. Here, we can say that a quadrilateral is a parallelogram if a pair of opposite sides are equal and parallel.
Properties of Quadrilaterals
We have studied many properties of a triangle and a quadrilateral. Now let’s examine another result that is related to the midpoint of the sides of a triangle. Do the following activity.
Draw a triangle and mark the midpoints E and F of the two sides of the triangle. Join the points E and F. We will see that the line segment joining the midpoint of two sides of the triangle is parallel to the third side.
Significant result based on Mathematics chapter 8 from class 9
- The sum of the angles of a quadrilateral is 360°.
- The diagonal of a parallelogram divides it into two congruent triangles.
- The diagonals of a rectangle intersect and are equal and vice versa.
- The diagonals of an equilateral intersect at each other and vice versa.
- The diagonals of a square cut each other at right angles and are equal, and vice versa.
- The line segment joining the midpoints of any two sides of a triangle is parallel to the third side and half.
- A line that passes parallel to one side of a triangle through the middle divides the third arm.
- A quadrilateral formed by connecting the midpoints of a quadrilateral’s sides, in order, is a parallelogram.
What will students learn in exercise 8.2 of class 9th Maths?
In exercise 8.2 of grade 9th Maths, students will learn two theorems (theorems 8.9 and 8.10) and solve questions based on these theorems. Theorem 8.9 and 8.10 are:
- Theorem 8.9: The line segment joining the mid-points of two sides of a triangle is parallel to the third side.
- Theorem 8.10: The line drawn through the mid-point of one side of a triangle, parallel to another side bisects the third side.
Which theorem is important for exams from exercise 8.2 of CBSE Class 9 Maths?
Theorem 8.9, also known as the Mid-point theorem, is the essential theorem from chapter 8. It is the most frequent question in all exams.
Is exercise 8.2 of class 9th math tough?
Exercise 8.2 of class 9th Maths is not easy and not tough. It lies in the middle of easy and tough because some problems of this exercise are easy, and some are complicated. However, the difficulty level of any topic varies from student to student. So, exercise 8.2 of class 9th Maths is easy, or tough depends on students also. Some students find it difficult, some find it easy, and some find it in the middle of easy and difficult.
Which example from class 9 Maths exercise 8.2 is important for exams?
Only two examples are covering exercise 8.2, that is, for example, 7 and 8. Example 8 is considered as a good question for exams.
Is exercise 8.2 of class 9th Maths lengthy?
No, exercise 8.2 of class 9th Maths is not at all lengthy. This exercise has only two examples and 7 questions. Students need a maximum of 2 days to complete exercise 8.2 of class 9th Maths if they give at least 2 hours per day to this exercise. This time also depends on student’s working speed, efficiency, capability and many other factors.
Which is the most important question from exercise 8.2 of 9th Maths?
Class 9 Maths exercise 8.2 is consists of 7 questions. Question 1 is easy but important for exams. Question number 5 is tricky and frequently comes in exams.
Which problems of exercise 8.2 of grade 9th Maths can come in the final exams?
Exercise 8.2 of class 9th Maths has 2 examples and 7 questions. All the problems of exercise 8.2 are equally important from the exam point of view. Students should practice all examples and questions of this exercise for the exams because any example and any question can come from this exercise in the final exams. Three or more than three marks questions can come from this exercise.