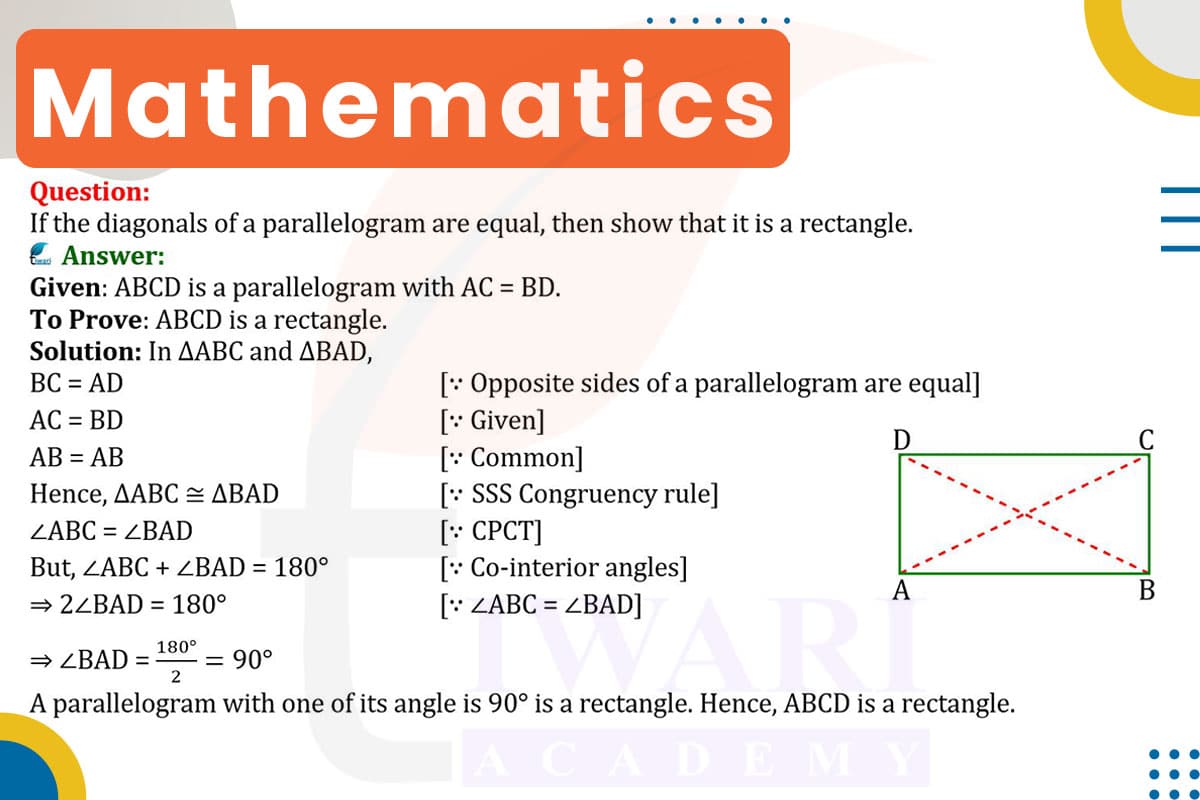
To show that a parallelogram with equal diagonals is a rectangle, we start by noting the properties of a parallelogram: opposite sides are equal and parallel, and its diagonals bisect each other. In a parallelogram, if the diagonals are equal, it implies that each diagonal divides the parallelogram into two congruent triangles. Since the diagonals are equal and bisect each other, the triangles formed are not only congruent but also isosceles. In an isosceles triangle, the angles adjacent to the equal sides are equal. Therefore, each angle of the parallelogram must be a right angle, making it a rectangle.
Let’s discuss in detail
Parallelograms and Rectangles
A parallelogram is a four-sided figure with opposite sides that are parallel and equal in length. Rectangles are a special type of parallelogram where each interior angle is a right angle (90 degrees). Understanding the relationship between parallelograms and rectangles is crucial in geometry, particularly in proving certain properties about these shapes. The key to linking parallelograms to rectangles lies in their diagonals. Diagonals are straight lines connecting opposite corners of a quadrilateral. In parallelograms, these diagonals have unique properties that can lead to significant conclusions about the nature of the shape.
Properties of Parallelograms
Parallelograms have several defining properties. Firstly, opposite sides are equal in length and parallel. Secondly, opposite angles are equal. Thirdly, the diagonals of a parallelogram bisect each other, meaning they cut each other exactly in half. This bisecting property is crucial as it implies that each diagonal divides the parallelogram into two congruent triangles. These properties set the stage for understanding more complex characteristics of parallelograms, such as the conditions under which they become rectangles.
Diagonals in Parallelograms
In a parallelogram, the diagonals have a unique relationship. While they bisect each other, they are not necessarily equal in length. When the diagonals are equal, it leads to a special scenario. The equality of diagonals means that each diagonal divides the parallelogram into two congruent triangles. These triangles are not only congruent but also isosceles, given the equal lengths of the diagonals. This isosceles nature leads to equal angles at the base of these triangles, which has implications for the angles of the parallelogram itself.
Transition to Rectangles
When a parallelogram has equal diagonals, it suggests a symmetry that is not present in all parallelograms. This symmetry implies that the angles adjacent to each side of the parallelogram are equal. Since the diagonals bisect each other and are equal, the angles formed at the intersection are also equal. This equality of angles is a step towards proving that the parallelogram is a rectangle. In a rectangle, not only are opposite sides equal and parallel, but each of its angles is a right angle.
Proving the Parallelogram is a Rectangle
To prove that a parallelogram with equal diagonals is a rectangle, we focus on its angles. The congruent isosceles triangles formed by the diagonals imply that the angles adjacent to each side of the parallelogram are equal. In geometry, if a quadrilateral has one right angle, then the presence of equal opposite angles and parallel sides ensures that all angles are right angles. Therefore, in our parallelogram, if we can prove that at least one angle is a right angle, then the parallelogram is a rectangle. The symmetry provided by the equal diagonals suggests that this is indeed the case.
The Rectangle Identity
In conclusion, a parallelogram with equal diagonals possesses a symmetry that transforms it into a rectangle. The equal diagonals create congruent isosceles triangles, leading to equal angles adjacent to each side. This symmetry, combined with the properties of parallelograms, ensures that all interior angles are right angles, fulfilling the criteria for a rectangle. Thus, in the realm of geometry, a parallelogram with equal diagonals is not just a simple quadrilateral but elevates to the more specific and symmetric form of a rectangle.
Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.1 in Detail

