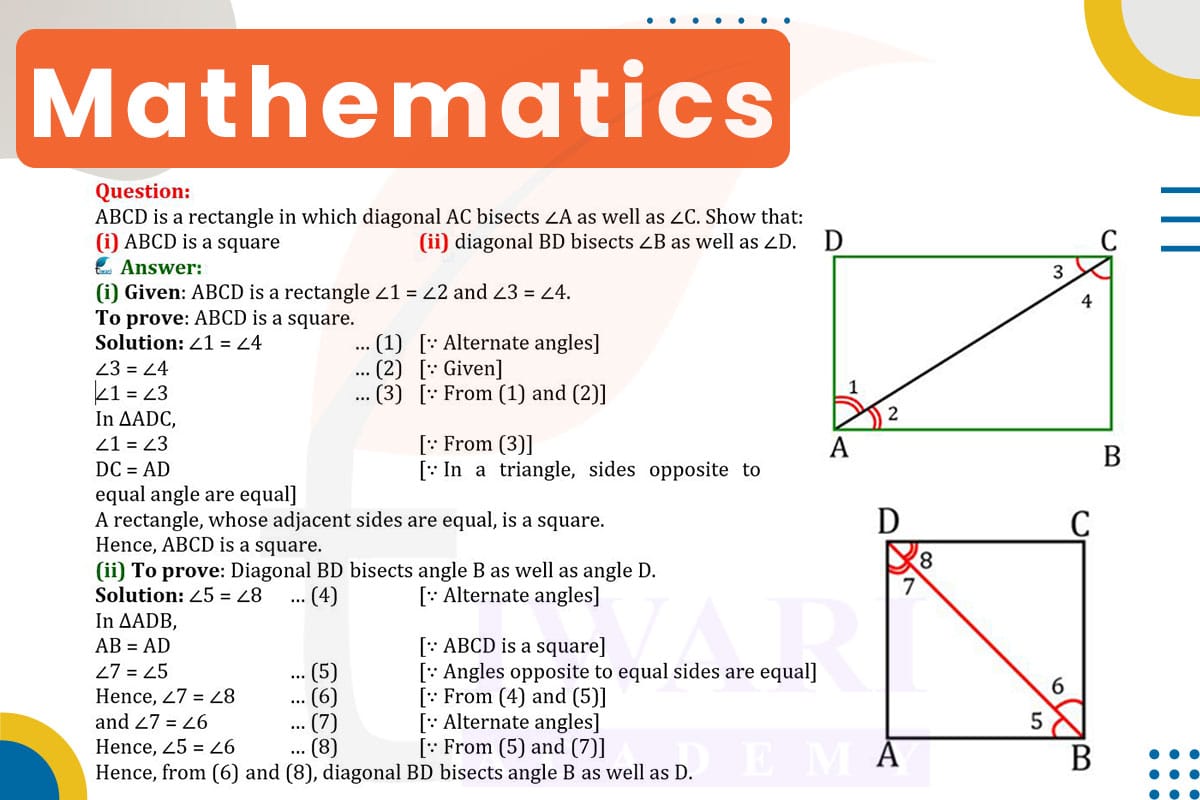
ABCD is a rectangle in which diagonal AC bisects ∠A as well as ∠C. Show that: (i) ABCD is a square (ii) diagonal BD bisects ∠B as well as ∠D.

Let’s discuss in detail

Consider rectangle ABCD, a fundamental shape in geometry characterized by right angles and opposite sides of equal length. A unique feature is introduced when its diagonal AC bisects ∠A and ∠C. This specific condition in a rectangle is not typical and leads to intriguing geometric properties. Understanding how the diagonals interact with the angles and sides of the rectangle can reveal much about the nature of the shape, potentially transforming it into another geometric figure with distinct characteristics.

In rectangle ABCD, diagonal AC bisecting ∠A and ∠C means that it divides these angles into two equal parts. Since the angles in a rectangle are 90 degrees, the bisected angles at ∠A and ∠C are each 45 degrees. This bisecting property is significant because it introduces an element of symmetry in the rectangle that is not inherently present in all rectangles. This symmetry suggests that ABCD might possess additional properties that elevate it from a mere rectangle to another geometric figure.

The bisecting of angles ∠A and ∠C by diagonal AC creates two isosceles right triangles, ABC and ADC, within the rectangle. In these triangles, the legs (sides of the rectangle) are congruent, and the hypotenuses (diagonal AC) are equal. This configuration is critical because it implies that all sides of these triangles are equal, given the 45-45-90 degree triangle rule. This equality of sides within the triangles suggests that the sides of the rectangle might also be equal, leading to a significant geometric conclusion about the nature of ABCD.

ABCD as a Square
Given that the sides of triangles ABC and ADC are equal, it follows that AB = BC and AD = CD. In a rectangle, opposite sides are equal by definition, so AB = CD and BC = AD. This equality of all four sides of ABCD means that it is not just a rectangle but also a square. A square is a special type of rectangle where all sides are equal, and the bisecting property of diagonal AC in ABCD fulfills this criterion, thus proving that ABCD is indeed a square.

Now, considering diagonal BD in square ABCD, the properties of a square come into play. In a square, both diagonals have similar properties. Since diagonal AC bisects ∠A and ∠C, creating 45-degree angles, it is logical to conclude that diagonal BD will also bisect the angles at its endpoints, ∠B and ∠D. This is due to the symmetry and equal properties of a square, where the diagonals are congruent and bisect the angles at the vertices.

In conclusion, the bisecting property of diagonal AC in rectangle ABCD leads to the revelation that ABCD is, in fact, a square. This transformation from a rectangle to a square is a result of the equal bisected angles at ∠A and ∠C, which implies equal sides. Furthermore, the properties of diagonal BD, bisecting ∠B and ∠D, reinforce the square’s symmetry and congruence. This example illustrates the fascinating interplay of geometric properties and theorems, showcasing the depth and interconnectedness of geometric principles in defining and transforming shapes.

Discuss this question in detail or visit to Class 9 Maths Chapter 8 for all questions.
Questions of 9th Maths Exercise 8.1 in Detail