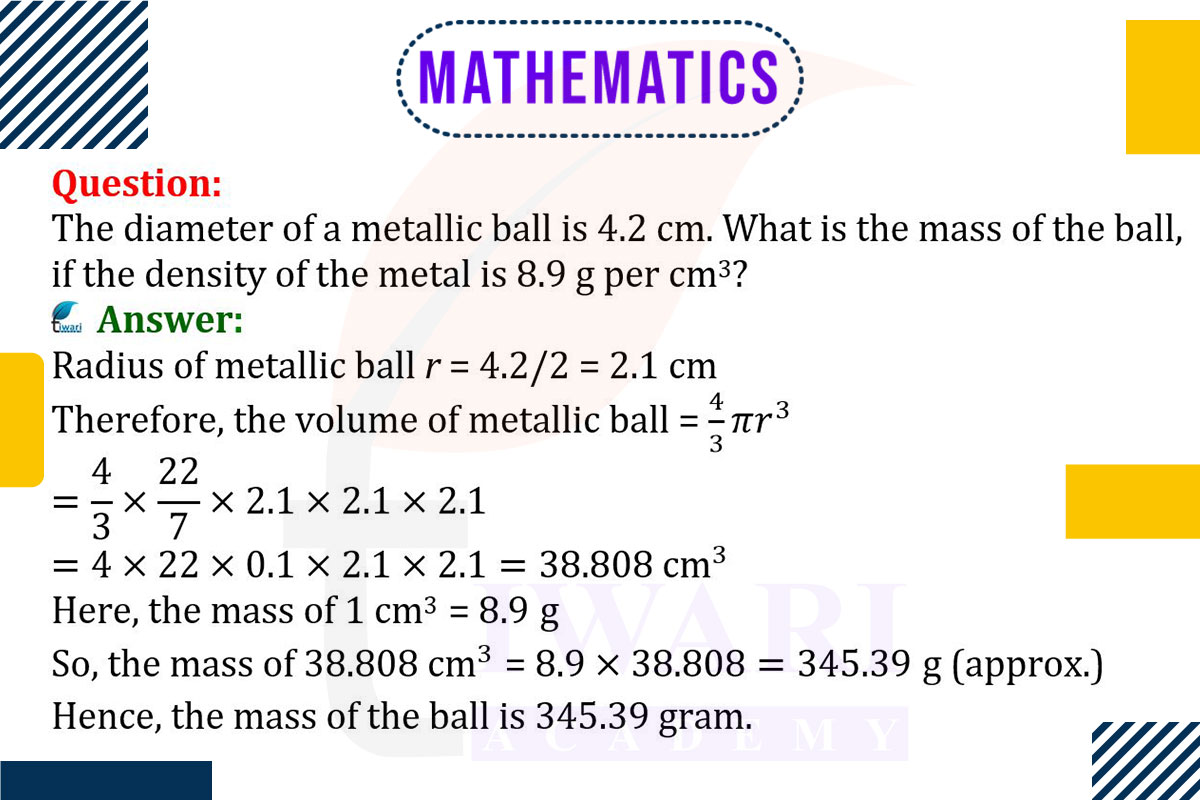
To find the mass of a metallic ball, first calculate its volume and then use the density of the metal. The volume of a sphere is given by V = (4/3)πr³, where r is the radius. For a ball with a diameter of 4.2 cm, the radius r is half of this, so r = 2.1 cm. The volume V is V = (4/3)π × 2.1³ cm³.
The mass of the ball can be found using the formula Mass = Density × Volume. With a density of 8.9 g/cm³, the mass is
Mass = 8.9 × (4/3)π × 21³ g. This calculation yields the mass of the metallic ball based on its volume and the given density of the metal.
Let’s discuss in detail
Relationship Between Mass, Volume, and Density
Understanding the relationship between mass, volume, and density is crucial in various scientific and engineering fields. Mass is a measure of the amount of matter in an object, volume quantifies the space that object occupies, and density is the mass per unit volume. This relationship is particularly important in material science, where the properties of a substance are determined by these factors. In the context of a metallic ball, determining its mass involves calculating its volume and then applying the known density of the material from which it is made.
The Concept of Volume in Spherical Objects
The volume of a sphere is a key factor in determining its physical properties, including its mass when the density is known. The volume of a sphere is calculated using the formula V = (4/3)πr³, where V is the volume and r is the radius of the sphere. This formula is derived from mathematical principles of geometry and calculus. For a spherical object like a metallic ball, knowing its volume is the first step in calculating its mass.
Calculating the Volume of the Metallic Ball
For a metallic ball with a diameter of 4.2 cm, the radius is half of the diameter, which is 2.1 cm. Substituting this radius into the volume formula, the calculation becomes V = (4/3)π × 2.1³ cm³. This computation yields the volume of the ball in cubic centimeters. The accuracy of this volume calculation is crucial as it directly influences the accuracy of the subsequent mass calculation.
Understanding Density and Its Role in Mass Calculation
Density is defined as the mass per unit volume of a substance and is a critical property in material science. For the given metallic ball, the density of the metal is provided as 8.9 g/cm³. This means that each cubic centimeter of the metal has a mass of 8.9 grams. Density plays a pivotal role in determining the mass of an object when its volume is known, making it a fundamental concept in physics and engineering.
Determining the Mass of the Metallic Ball
To find the mass of the metallic ball, multiply its volume by its density. The formula used is Mass = Density × Volume. With the calculated volume of the ball and the given density of 8.9 g/cm³, the mass is Mass = 8.9 × (4/3)π × 2.1³ grams. This calculation provides the mass of the ball, reflecting the amount of metal used to make it and its physical heft.
The Interplay of Geometry and Material Science
In conclusion, calculating the mass of a metallic ball by using its diameter and the density of the metal exemplifies the interplay between geometry and material science. This process highlights the importance of understanding geometric principles and material properties in practical applications. Such calculations are not only fundamental in academic settings but also have significant implications in industries like manufacturing, where they are used for designing and creating objects with specific properties. The ability to calculate mass from volume and density is a valuable skill in various scientific and engineering disciplines.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.4 in Detail

