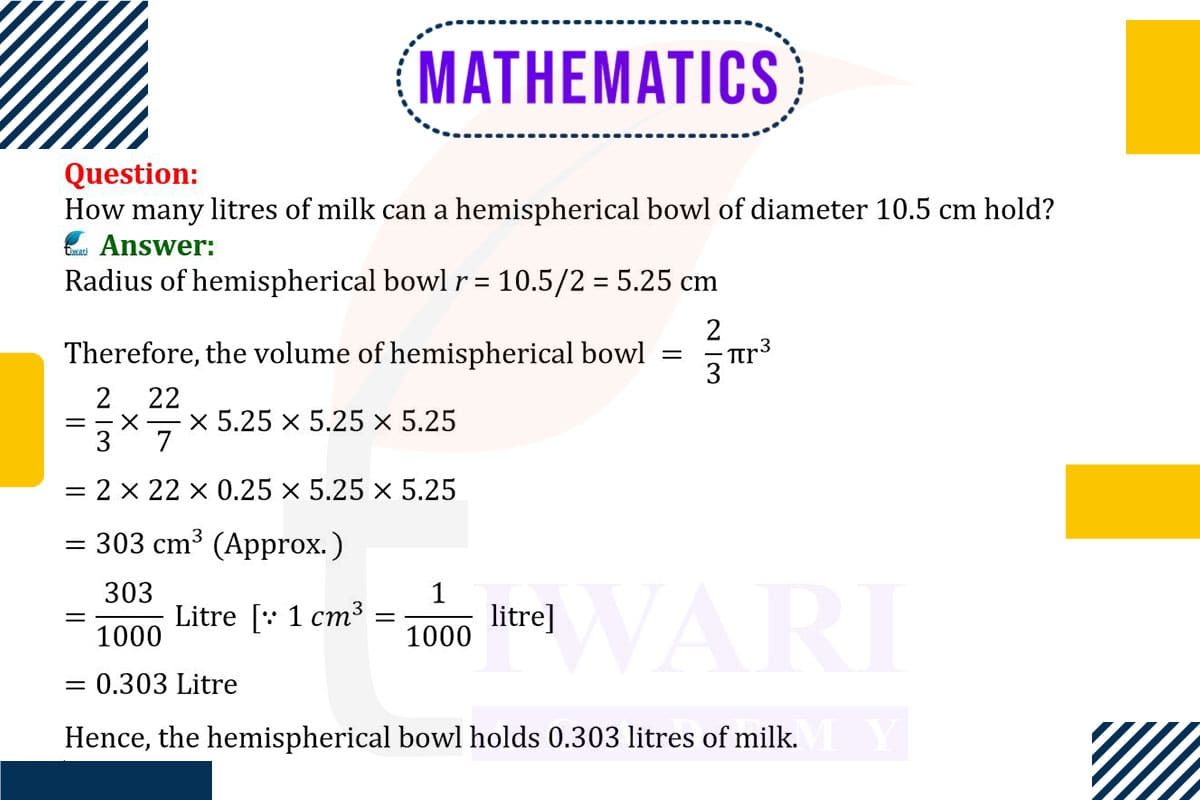
To determine the capacity in liters of a hemispherical bowl, first calculate its volume and then convert that volume to liters. The volume of a hemisphere is half the volume of a full sphere. The formula for the volume of a sphere is V = (4/3)πr³, where r is the radius.
For a bowl with a diameter of 10.5 cm, the radius r is half of this, so r = 5.25 cm. The volume of the hemisphere is half the sphere’s volume, so V = 1/2 × (4/3)π × 5.25³ cm³.
To convert cubic centimeters to liters, use the fact that 1 liter equals 1000 cubic centimeters. Therefore, divide the volume in cubic centimeters by 1000 to get the capacity in liters. This calculation will give the maximum amount of milk the bowl can hold.
Let’s discuss in detail
Volume Calculation of Hemispherical Objects
Calculating the volume of hemispherical objects is a common problem in geometry that has practical applications in everyday life. A hemisphere is half of a sphere, and understanding how to calculate its volume is essential in various fields, from culinary arts to industrial design. In this context, we are interested in determining the capacity of a hemispherical bowl, which is a practical problem that can be encountered in settings like cooking or storage.
Understanding the Geometry of a Hemisphere
A hemisphere is a three-dimensional shape representing half of a sphere. The key to calculating its volume lies in understanding the properties of a sphere. The volume of a sphere is given by the formula V = (4/3)πr³, where r is the radius. Since a hemisphere is half of a sphere, its volume is exactly half of this value. This relationship between the volume of a sphere and a hemisphere is fundamental to solving problems involving hemispherical objects.
Determining the Radius of the Hemisphere
The first step in calculating the volume of a hemisphere is to determine its radius. The radius is half the diameter of the sphere. For a hemispherical bowl with a diameter of 10.5 cm, the radius r is half of this, which is 5.25 cm. This radius is a crucial measurement as it directly influences the volume calculation.
Calculating the Volume of the Hemisphere
With the radius determined, the volume of the hemisphere can be calculated. Since the volume of a full sphere is V = (4/3)πr³, the volume of the hemisphere is half of this. Therefore, the volume V of the hemisphere is V = 1/2 × (4/3)π × 5.25³ cubic centimeters. This formula gives the volume of the hemisphere, which represents the space it occupies.
Converting Volume to Liters
To find out how many liters of milk the bowl can hold, the volume in cubic centimeters needs to be converted to liters. Since 1 liter is equivalent to 1000 cubic centimeters, the volume of the hemisphere in cubic centimeters is divided by 1000 to convert it to liters. This conversion is essential for practical applications where measurements are typically made in liters or milliliters.
Practical Application in Everyday Life
In conclusion, calculating the volume of a hemispherical bowl and converting it to liters is a practical application of geometric principles. This calculation is not only relevant in academic settings but also has real-world applications, such as in cooking or manufacturing. Understanding how to perform these calculations allows for accurate measurement and efficient use of resources, highlighting the importance of geometry in everyday life.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.4 in Detail

