To find the volume of a sphere when given its surface area, we first use the surface area to find the radius and then calculate the volume. The surface area A of a sphere is given by A = 4πr², where r is the radius. Given a surface area of 154 cm², we can rearrange the formula to solve for r: r = √(A/4π). Substituting A = 154 cm², we find the radius.
Once the radius is known, the volume V of the sphere can be calculated using V = 4πr³. This process demonstrates the relationship between the surface area and volume of a sphere, a fundamental concept in geometry.
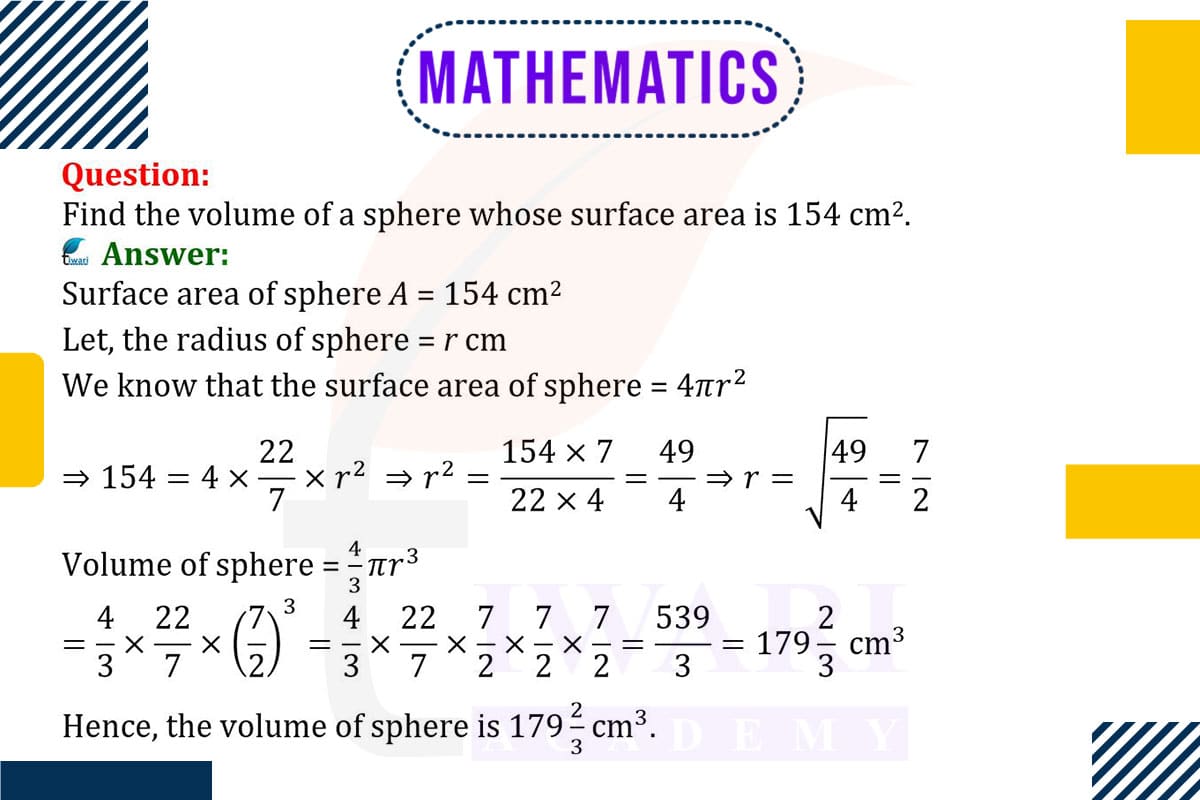
Let’s discuss in detail
Sphere Volume Calculation from Surface Area
Determining the volume of a sphere from its surface area is a classic problem in geometry, illustrating the interrelation between different geometric properties of a sphere. The surface area provides information about the sphere’s exterior, while the volume describes the space it occupies. This calculation is not only a fundamental exercise in geometry but also has practical applications in fields like material science, architecture, and engineering, where understanding the dimensions and capacity of spherical objects is crucial.
Understanding the Surface Area of a Sphere
The surface area of a sphere is a measure of the total area that the surface of the sphere occupies. It is calculated using the formula A = 4πr, where A is the surface area and r is the radius of the sphere. This formula is derived from the principles of calculus and provides a direct relationship between the radius of a sphere and its surface area. In practical terms, knowing the surface area of a sphere can provide insights into its size and scale.
Calculating the Radius from the Surface Area
To find the volume of a sphere, the radius must first be determined from its surface area. Given the surface area A, the radius r can be calculated by rearranging the surface area formula: r = √(A/4π). For a sphere with a surface area of 154 cm², this calculation involves substituting 154 cm² into the formula and solving for r. This step is crucial as the radius is a key component in calculating the volume.
The Formula for Sphere Volume
Once the radius is known, the volume of the sphere can be calculated. The volume V of a sphere is given by the formula V = (4/3)πr³. This formula indicates that the volume of a sphere is directly proportional to the cube of its radius, highlighting a cubic relationship between the radius and the volume. This relationship is fundamental in understanding how changes in the size of a sphere affect its volume.
Applying the Volume Formula
With the radius calculated from the surface area, it can be substituted into the volume formula to find the sphere’s volume. This involves cubing the radius and multiplying it by
(4/3)π. This calculation will yield the volume of the sphere in cubic centimeters, providing a measure of how much space the sphere occupies. This step is a direct application of geometric principles to solve a practical problem.
The Significance of Geometric Calculations
In conclusion, calculating the volume of a sphere from its surface area is a clear demonstration of the practical application of geometric principles. This process is not only important in academic settings but also has real-world implications in various fields where understanding the dimensions and capacities of spherical objects is necessary. The ability to transition from surface area to volume exemplifies the interconnected nature of geometric properties and underscores the importance of geometry in understanding and interpreting the physical world.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.4 in Detail

