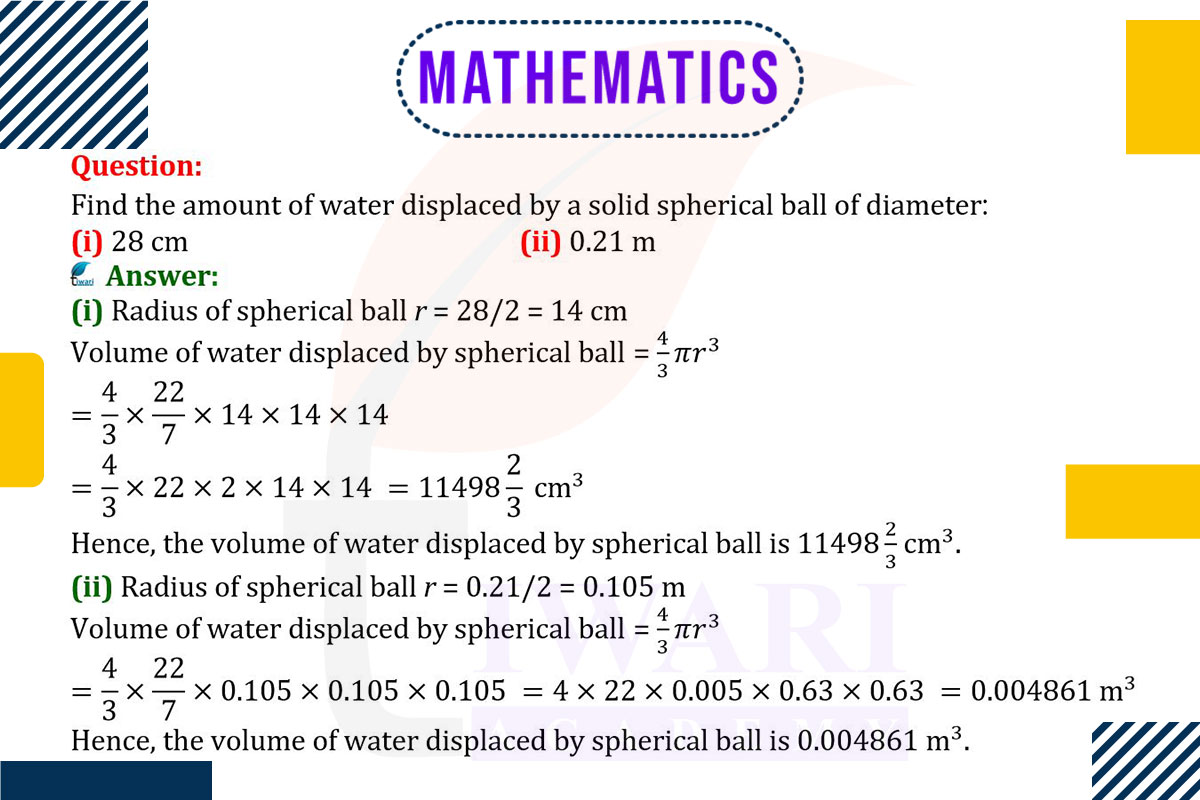
To find the amount of water displaced by a solid spherical ball, calculate the volume of the sphere, as the volume of water displaced will be equal to the volume of the sphere. The volume of a sphere is given by V = (4/3)πr³, where r is the radius.
(i) For a sphere with a diameter of 28 cm, the radius is half the diameter, so r = 14 cm. The volume V is V = (4/3)π × 14³ cm³.
(ii) For a sphere with a diameter of 0.21 m, the radius is r = 0.105 m. The volume V = (4/3)π × 0.105³ m³.
These calculations give the volume of water displaced by each spherical ball, reflecting the principle of displacement in fluid mechanics.
Let’s discuss in detail
Concept of Displacement in Fluid Mechanics
The principle of displacement in fluid mechanics is a fundamental concept, famously illustrated by Archimedes’ principle. It states that the volume of fluid displaced by an object submerged in a fluid is equal to the volume of the object. This principle is widely used in various fields, including engineering, physics, and maritime studies. In the context of a solid spherical ball being submerged in water, the amount of water displaced by the ball can be determined by calculating the volume of the sphere.
Understanding the Volume of a Sphere
The volume of a sphere is a key factor in determining the amount of fluid it will displace. This volume is calculated using the formula V = (4/3)πr³, where V is the volume and r is the radius of the sphere. The radius is half the diameter of the sphere. This formula is derived from the principles of calculus and is essential in geometric calculations involving spheres.
Calculating Water Displacement for a Sphere with 28 cm Diameter
For a spherical ball with a diameter of 28 cm, the radius is half of this, which is 14 cm. By substituting r = 14 cm into the volume formula, the calculation becomes V = (4/3)π × 14³ cm³. This volume represents the amount of water that would be displaced when the spherical ball is fully submerged in water. The calculation is straightforward and provides a clear understanding of the displacement caused by the ball.
Calculating Water Displacement for a Sphere with 0.21 m diameter.
Similarly, for a spherical ball with a diameter of 0.21 meters, the radius is half of this, which is 0.105 meters. Substituting r = 0.105 m into the volume formula, the calculation is V = (4/3)π × 0.105³ m³. This volume is the amount of water displaced by the sphere when it is completely submerged. The calculation in meters cubed is particularly relevant for larger objects and helps in understanding the displacement in a larger body of water.
Practical Applications of Displacement Calculations
The calculation of water displacement by spherical objects has practical applications in various fields. In naval architecture, it helps in determining the buoyancy and stability of vessels. In environmental studies, it aids in understanding the impact of submerged objects on aquatic ecosystems. In industries, these calculations are crucial for designing containers and storage systems. The principle of displacement is not just a theoretical concept but has real-world implications that are essential for scientific and industrial applications.
The Significance of Displacement in Fluid Dynamics
In conclusion, calculating the amount of water displaced by solid spherical balls of different diameters illustrates the practical application of the principles of fluid dynamics and geometry. These calculations are essential for understanding the interactions between solid objects and fluids, highlighting the importance of displacement in various scientific and engineering contexts. Whether for educational purposes or practical applications, the ability to calculate fluid displacement is a valuable skill, underscoring the relevance of fluid mechanics in our understanding of the physical world.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.4 in Detail

