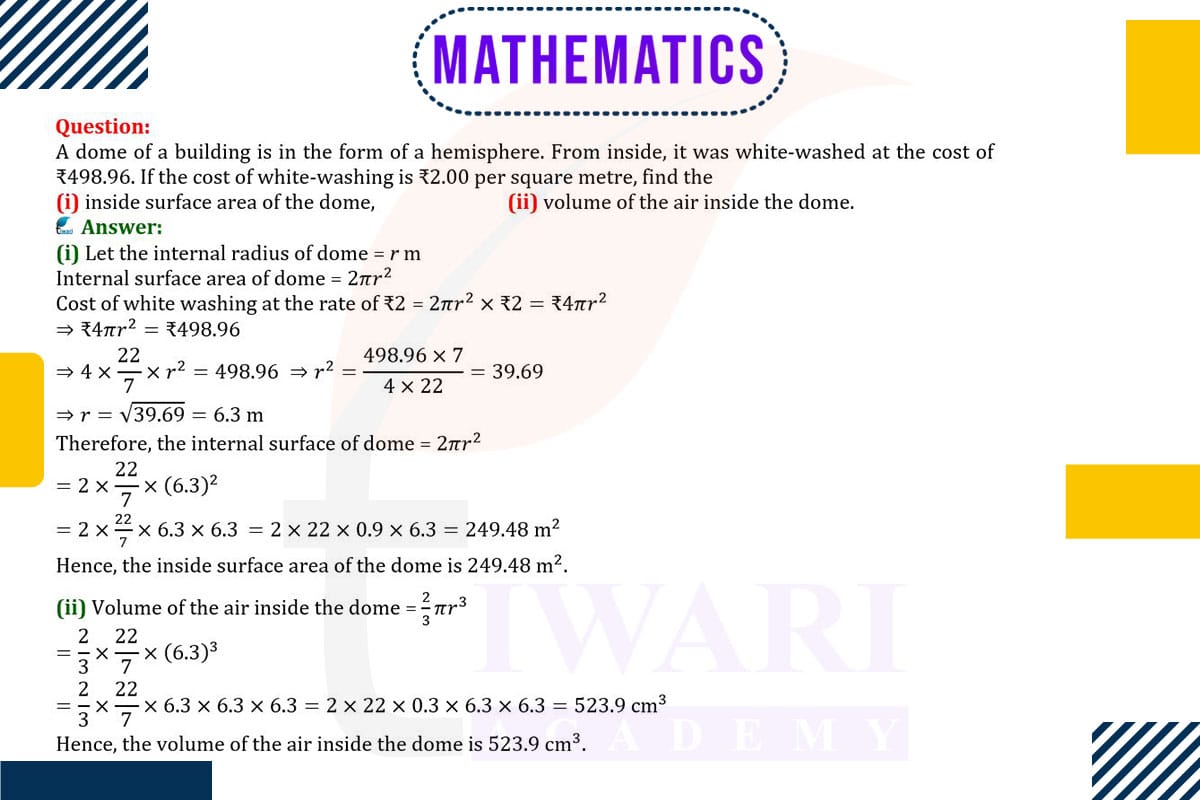
To find the inside surface area and the volume of air inside a dome shaped like a hemisphere, we can use the given cost of white-washing and the rate per square meter.
(i) The cost of white-washing is ₹4989.60 at ₹20 per square meter. The surface area A can be found by dividing the total cost by the cost per square meter: A = 4989.60/20 m².
(ii) The volume V of a hemisphere is given by V = (2/3)πr³. To find the volume, we first need the radius, which can be derived from the surface area of a hemisphere A = 2πr². Solving for r and then substituting it into the volume formula will give the volume of air inside the dome. This approach demonstrates how geometric formulas can be applied to practical problems.
Let’s discuss in detail
Geometric Problem Solving in Architecture
The application of geometry in architecture is evident in various structures, including domes. A dome, often hemispherical in shape, presents an interesting geometric problem when it comes to determining its surface area and internal volume. This is particularly relevant in tasks such as white-washing the interior of a dome, where understanding the surface area is crucial for cost estimation. Similarly, calculating the volume of air inside the dome can be important for ventilation and acoustics. Such problems demonstrate the practical application of geometric principles in architectural design and maintenance.
Calculating the Surface Area for White-Washing
The cost of white-washing the interior of a dome provides a direct way to calculate its surface area. Given that the cost of white-washing is ₹4989.60 and the rate is ₹20 per square meter, the surface area can be determined by dividing the total cost by the rate per square meter. This calculation is based on the formula A = (Total Cost)/(Rate per Square Meter). It yields the total area that needs to be white-washed, a crucial piece of information for planning and budgeting the maintenance work.
Deriving the Radius from the Surface Area
The surface area of a hemisphere is given by A = 2πr², where r is the radius. By rearranging this formula, the radius can be calculated from the known surface area. This step is essential because the radius is a key variable needed to calculate the volume of the dome. Once the surface area is known from the cost calculation, the radius can be derived, providing a basis for further calculations related to the dome’s geometry.
Volume Calculation of the Dome
The volume of a hemisphere, representing the air inside the dome, is calculated using the formula V = (2/3)πr³. This formula highlights the relationship between the radius of the hemisphere and its volume. After determining the radius from the surface area calculation, it can be substituted into this volume formula. The resulting volume represents the total space inside the dome, which is an important factor in architectural design, especially for considerations like air circulation and acoustics.
Practical Implications in Architecture
Calculating the surface area and volume of a dome has practical implications in architecture and construction. The surface area is directly related to the cost of materials and labor for tasks like white-washing, while the volume is important for environmental control within the structure. These calculations are not just theoretical exercises but are essential for the practical aspects of building design, maintenance, and renovation. They enable architects and engineers to make informed decisions about material usage, cost estimation, and structural design.
The Intersection of Geometry and Architecture
In conclusion, the task of calculating the surface area for white-washing and the volume of air inside a dome exemplifies the intersection of geometry and architecture. These calculations are crucial for efficient planning, cost estimation, and ensuring the functionality of architectural structures. The application of geometric formulas to real-world problems in architecture demonstrates the importance of mathematics in practical scenarios. Understanding these concepts allows architects, engineers, and maintenance teams to effectively plan, design, and maintain architectural structures, highlighting the indispensable role of geometry in the built environment.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.4 in Detail

