To find the diameter of a cone’s base given its volume and height, we use the formula for the volume of a cone, V = (1/3)πr²h, where V is the volume, r is the radius, and h is the height.
Rearranging the formula to solve for r, we get r = √(3v/πh).
Given V = 48π cm³ and h = 9 cm, substitute these values to find r.
The diameter is twice the radius, so multiply the found radius by 2 to get the diameter of the base of the cone.
This calculation will yield the diameter, providing the full dimension of the cone’s base.
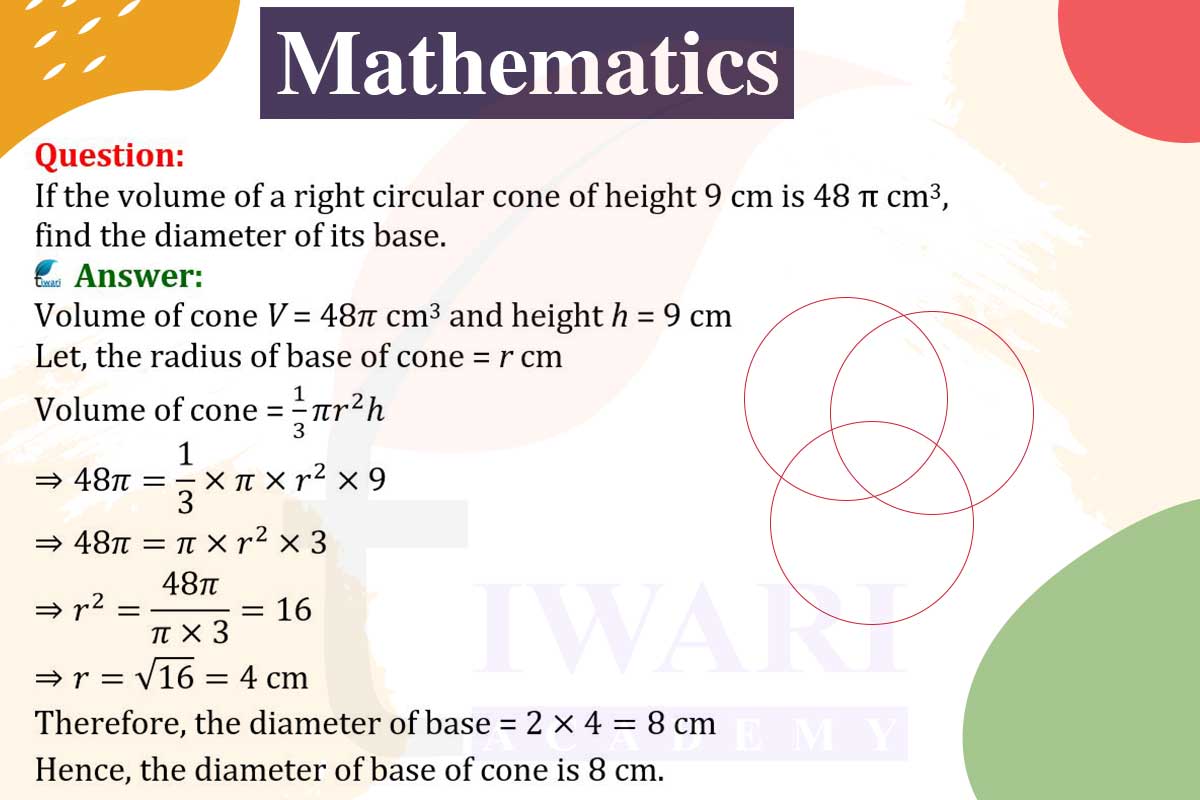
Let’s discuss in detail
Determining Cone Dimensions
The process of determining the dimensions of a cone, particularly the diameter of its base when given its volume and height, is a fundamental exercise in geometry. A right circular cone is a three-dimensional geometric figure with a circular base and a pointed top, known as the apex. The volume of a cone is a key attribute that represents the amount of space it occupies. This volume is intrinsically linked to the cone’s height and the radius of its base. Understanding how to manipulate the volume formula to find other dimensions like the diameter is crucial in various fields, from engineering to design.
The Volume Formula of a Right Circular Cone
The volume of a right circular cone is calculated using the formula V = (1/3)πr²h, where V is the volume, r is the radius of the base, and h is the height of the cone. This formula is derived from the fact that the volume of a cone is one-third that of a cylinder with the same base and height. The presence of π (pi), a constant approximately equal to 3.14, is due to the circular nature of the cone’s base. This formula is essential for solving problems related to cone dimensions.
Rearranging the Volume Formula to Find the Radius
To find the radius of the cone’s base when the volume and height are known, the volume formula needs to be rearranged. By isolating the radius, the formula becomes r = √(3v/πh). This rearrangement allows for the calculation of the radius using the known values of volume and height. It’s a straightforward algebraic manipulation that provides a method to determine one dimension of the cone from the others.
Applying the Formula to a Specific Problem
In the given problem, the volume of the cone is 48π cm³, and the height is 9 cm. Substituting these values into the rearranged formula gives r = √[(3 × 48π)/(π × 9)]. This calculation will yield the radius of the cone’s base. It’s important to note that the π in the numerator and denominator will cancel each other out, simplifying the calculation.
Calculating the Diameter of the Cone’s Base
Once the radius is found, the diameter can be easily calculated as it is simply twice the radius. Therefore, the diameter is given by D = 2r. This step is crucial as it translates the radius into a more comprehensive dimension, the diameter, which gives a complete idea of the size of the cone’s base. This conversion is straightforward but essential for fully understanding the dimensions of the cone.
The Importance of Cone Dimension Calculations
In conclusion, the ability to calculate the diameter of a cone’s base from its volume and height is a valuable skill in geometry. This process demonstrates the practical application of mathematical formulas and principles in solving real-world problems. Whether in academic settings, engineering, architecture, or design, understanding these geometric calculations is crucial. It allows for the accurate creation and interpretation of three-dimensional shapes, underscoring the significance of geometry in various professional and everyday contexts.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.3 in Detail

