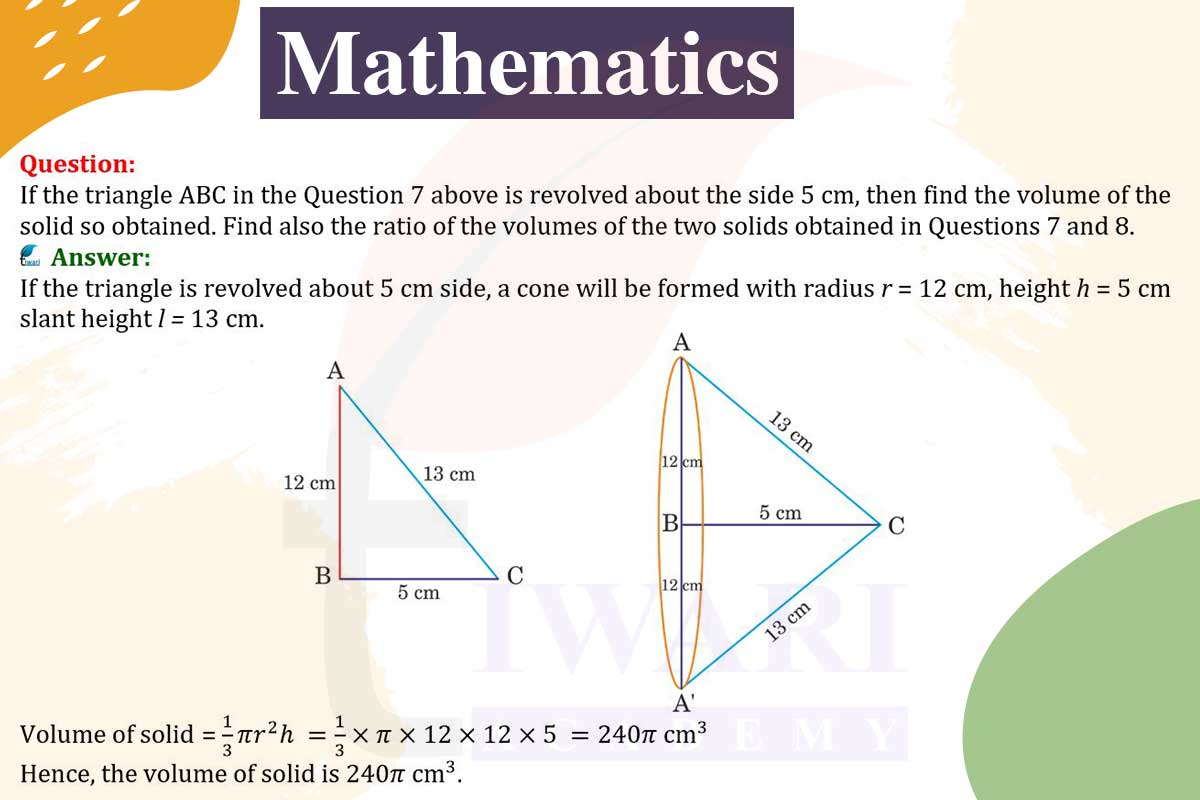
When the right triangle ABC with sides 5 cm, 12 cm, and 13 cm is revolved around its 5 cm side, it forms a cylindrical solid. The 5 cm side becomes the height (h) of the cylinder, and the 12 cm side, being perpendicular to the axis of rotation, forms the radius (r) of the cylinder’s base. The volume of a cylinder is given by the formula V = (1/3)πr²h. Substituting r = 12 cm and h = 5 cm, the volume V is calculated as V = (1/3)π × 12² × 5 cm³. This calculation yields the volume of the solid formed by the revolution of the right triangle around its 5 cm side.
Let’s discuss in detail
Geometric Solids of Revolution
The concept of solids of revolution is a fascinating area in geometry, where a two-dimensional shape, such as a triangle, is rotated around an axis to form a three-dimensional object. This process is not just a theoretical construct in mathematics but has practical applications in fields like engineering, architecture, and design. In this specific case, we consider a right triangle with sides 5 cm, 12 cm, and 13 cm, and explore the solid formed when this triangle is revolved around one of its sides.
The Right Triangle ABC and Its Dimensions
The right triangle ABC in question is defined by its sides measuring 5 cm, 12 cm, and 13 cm. This set of measurements confirms that it is a Pythagorean triplet, indicating a right-angled triangle. The sides of the triangle represent different dimensions: the base, the perpendicular, and the hypotenuse. When this triangle is rotated around one of these sides, the resulting solid’s shape and volume are determined by which side is chosen as the axis of rotation.
Forming a Cylinder by Revolving Around the 5 cm Side
Revolving the triangle around its 5 cm side results in the formation of a cylindrical solid. In this scenario, the 5 cm side of the triangle becomes the height of the cylinder, and the 12 cm side, being perpendicular to the axis of rotation, forms the radius of the cylinder’s base. The 13 cm side, the hypotenuse, does not directly contribute to the dimensions of the cylinder but is essential in establishing the right-angled nature of the triangle.
Calculating the Volume of the Cylinder
The volume of a cylinder is calculated using the formula V = (1/3)πr²h, where V is the volume, r is the radius of the base, and h is the height. In this case, the radius r is 12 cm, and the height h is 5 cm. Substituting these values into the formula, the volume of the cylinder is computed as V = (1/3)π × 12² × 5 cm³. This formula is a fundamental principle in geometry and is widely used to determine the volume of cylindrical objects.
The Significance of the Calculation
This calculation is not merely an academic exercise but has practical implications. For example, in industrial design and manufacturing, understanding how to calculate the volume of solids of revolution is crucial for material estimation, cost analysis, and design specifications. In academic settings, it helps students grasp the concepts of volume and the transformation of shapes, enhancing their spatial reasoning and understanding of geometry.
The Importance of Geometric Transformations
In conclusion, calculating the volume of a solid formed by revolving a right triangle around one of its sides illustrates the interplay between two-dimensional shapes and three-dimensional objects. It highlights the importance of understanding geometric principles in both theoretical and practical contexts. This knowledge is not only academically significant but also essential in various practical fields, from engineering to design, showcasing the real-world applications of geometric transformations.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.3 in Detail

