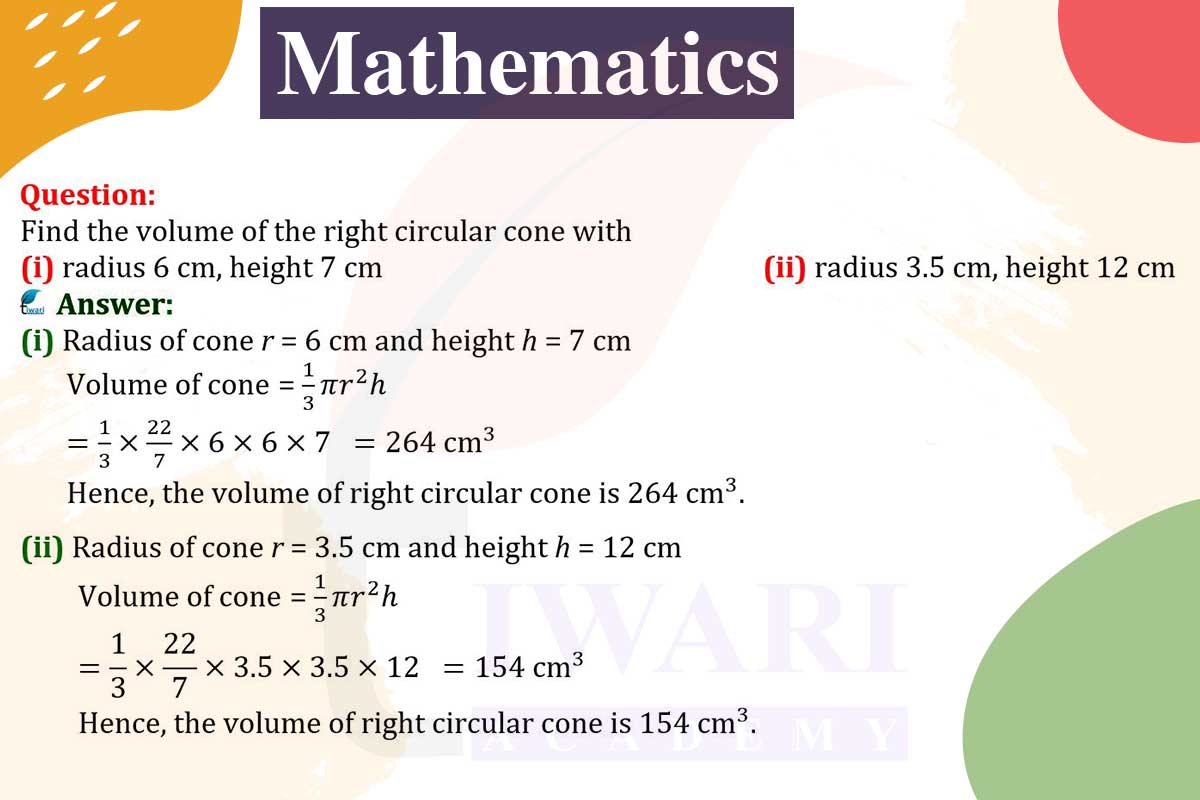
To find the volume of a right circular cone, use the formula
Volume = (1/3)πr²h, where r is the radius and h is the height.
For a cone with radius 6 cm and height 7 cm:
Volume = (1/3)π × 6² × 7 ≈ 264 cubic cm.
For a cone with radius 3.5 cm and height 12 cm:
Volume = (1/3)π × 3.5² × 12 ≈ 173.4 cubic cm.
These calculations provide the volumes for the respective cones.
Let’s discuss in detail
Volume of a Right Circular Cone
The concept of volume in geometry is a fundamental aspect that helps in determining the capacity of three-dimensional objects. In the case of a right circular cone, which is a common geometric shape, the volume is a measure of how much space it occupies. A right circular cone is characterized by a circular base and a pointed top, known as the apex or vertex, which is perpendicular to the base. The volume of a cone is a function of two key measurements: the radius of its base and its height. The radius is the distance from the center of the base to any point on its circumference, while the height is the perpendicular distance from the base to the apex. Understanding these dimensions is crucial for calculating the volume of the cone.
Formula for the Volume of a Right Circular Cone
The volume of a right circular cone can be calculated using the formula V = (1/3)πr²h, where V represents the volume, r is the radius of the base, h is the height of the cone, and π is a constant approximately equal to 3.14159. This formula is derived from the principle that the volume of a cone is one-third of the volume of a cylinder with the same base and height. This relationship is vital in understanding the spatial properties of conical shapes and is widely used in various fields, including engineering, architecture, and mathematics.
Calculating the Volume of a Cone with Specific Dimensions
To illustrate the application of the volume formula, consider a right circular cone with a radius of 6 cm and a height of 7 cm. By substituting these values into the formula, the volume can be calculated as follows: V = (1/3)π × 6² × 7. This calculation involves squaring the radius, multiplying it by the height, and then multiplying the result by π and dividing by 3. The computed volume provides the amount of space enclosed within the cone, which is an essential measure in practical applications.
Example with Different Dimensions
Another example involves a cone with a radius of 3.5 cm and a height of 12 cm. Applying the same formula, the volume is computed as V = (1/3)π × 3.5² × 12. This process highlights the versatility of the formula, as it can be applied to cones of various sizes. The result of this calculation gives the volume of this particular cone, demonstrating how changing the dimensions significantly alters the volume. This adaptability of the formula makes it a powerful tool in geometric calculations.
Practical Applications of Cone Volume Calculation
The calculation of the volume of a right circular cone has numerous practical applications. In architecture and construction, it is used to determine the material requirements for conical structures. In manufacturing, it helps in designing objects with conical parts, such as funnels and containers. In environmental science, cone volume calculations can be used to estimate soil erosion in conical depressions. Understanding the volume of a cone is also crucial in academic fields, particularly in teaching geometry and spatial reasoning.
The Significance of Cone Volume in Various Fields
In conclusion, the volume of a right circular cone is a fundamental geometric concept with wide-ranging applications. The formula V = (1/3)πr²h is a simple yet powerful tool that provides essential information about the space a cone occupies. From academic to practical real-world applications, the ability to calculate the volume of a cone is invaluable. It not only enhances our understanding of geometric shapes but also aids in the practical design and analysis of objects and structures in various fields.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.3 in Detail

