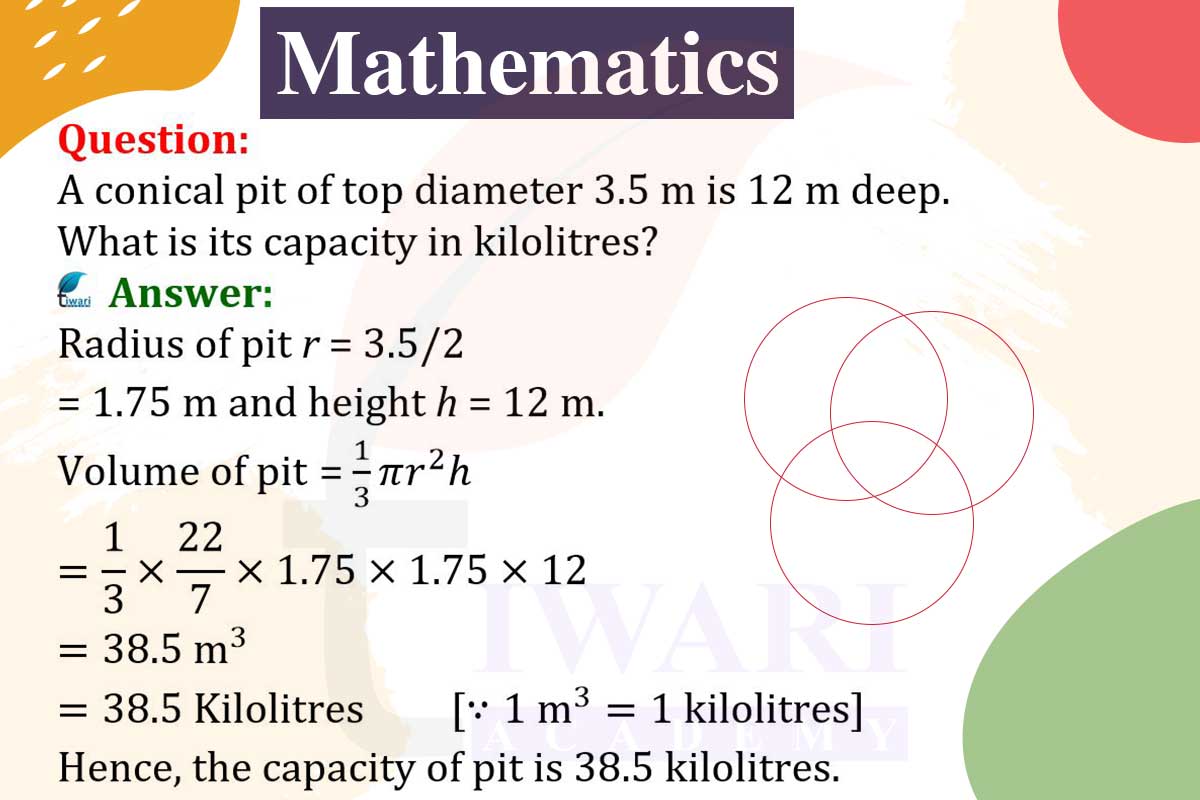
To find the capacity of a conical pit in kilolitres, we first calculate its volume in cubic meters and then convert it to kilolitres (1 kilolitre = 1 cubic meter). The volume of a cone is given by V = (1/3)πr²h, where r is the radius and h is the height. For a pit with a top diameter of 3.5 m, the radius r is half of that, i.e., 1.75 m. The height h is 12 m. Substituting these values and π = 3.14 into the formula, we calculate the volume in cubic meters. This volume is equivalent to the capacity of the pit in kilolitres.
Let’s discuss in detail
Calculating the Capacity of a Conical Pit
The calculation of the capacity of a conical pit is a practical application of geometric principles. A conical pit, often used in construction, agriculture, or landscaping, is a three-dimensional shape with a circular opening that tapers smoothly to a point at a certain depth. The capacity of such a pit is essentially its volume, which indicates how much material it can hold. This is particularly important in scenarios like storage, waste disposal, or when estimating the amount of material needed to fill the pit.
Understanding the Geometry of a Cone
A cone is defined by its circular base and its height. The base’s radius and the height are crucial dimensions for calculating the volume. In the case of a conical pit, the top diameter is often given, from which the radius can be derived (radius is half the diameter). The height of the cone, in this context, is the depth of the pit. These dimensions are used in a specific formula to calculate the volume of the cone.
The Volume Formula for a Cone
The volume of a cone is calculated using the formula V = (1/3)πr²h, where V is the volume, r is the radius of the base, h is the height, and π is approximately 3.14. This formula is derived from the fact that the volume of a cone is a third of the volume of a cylinder with the same base and height. The formula is a fundamental aspect of geometry and is widely used in various fields.
Calculating the Radius and Applying the Formula
For a conical pit with a top diameter of 3.5 meters, the radius r is half of this, which is 1.75 meters. The depth of the pit, which is the height h of the cone, is given as 12 meters. Substituting these values into the volume formula, along with π = 3.14, allows us to calculate the volume of the pit. This calculation will provide the volume in cubic meters, which directly translates to the capacity of the pit.
Converting Volume to Capacity in Kilolitres
After calculating the volume in cubic meters, the next step is to convert this volume into kilolitres for practical understanding. Since 1 cubic meter is equivalent to 1 kilolitre, the volume in cubic meters directly gives us the capacity in kilolitres. This conversion is straightforward but essential for applications where measurements are more commonly understood or required in kilolitres, such as in water storage or large-scale construction projects.
Practical Implications of the Calculation
In conclusion, calculating the capacity of a conical pit in kilolitres is a clear demonstration of applying geometric principles to solve real-world problems. This calculation is not only crucial in academic settings but also has significant practical applications in various industries. Understanding how to compute the volume and convert it into a usable unit like kilolitres is essential for effective planning, resource management, and execution of projects involving conical structures or spaces.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.3 in Detail

