NCERT Solutions for Class 9 Maths Chapter 11 Exercise 11.3 Surface Areas and Volumes in Hindi and English Medium for session 2025-26. Questions of ex. 11.3 class 9th Maths are revised on the basis of revised syllabus and new NCERT books for CBSE 2025-26.
NCERT Solutions for Class 9 Maths Exercise 11.3
Class IX Maths Ex. 11.3 solutions updated for new academic session 2025-26. All the questions are solved in Hindi and English Medium. Videos and PDF files are also given to download for offline use. Solutions are simplified in such a way every student can use it comfortably.
Class 9 Maths Exercise 11.3 Questions in Detail
- Find the volume of the right circular cone with (i) radius 6 cm, height 7 cm (ii) radius 3.5 cm, height 12 cm.
- Find the capacity in litres of a conical vessel with (i) radius 7 cm, slant height 25 cm (ii) height 12 cm, slant height 13 cm.
- The height of a cone is 15 cm. If its volume is 1570 cm³, find the radius of the base.
- If the volume of a right circular cone of height 9 cm is 48 π cm³, find the diameter of its base.
- A conical pit of top diameter 3.5 m is 12 m deep. What is its capacity in kilolitres?
- The volume of a right circular cone is 9856 cm³. If the diameter of the base is 28 cm, find (i) height of the cone (ii) slant height of the cone (iii) curved surface area of the cone.
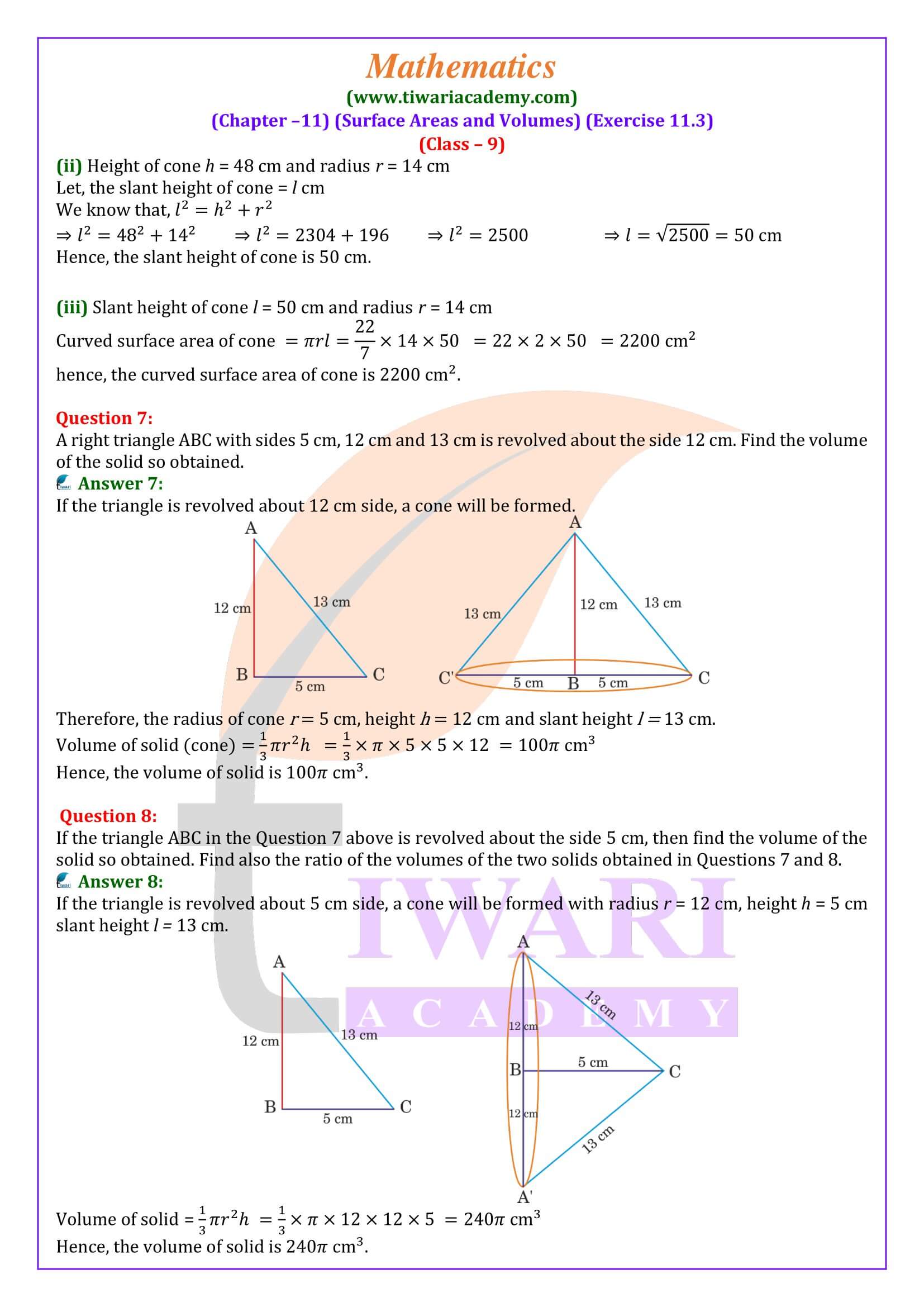
- A right triangle ABC with sides 5 cm, 12 cm and 13 cm is revolved about the side 12 cm. Find the volume of the solid so obtained.
- If the triangle ABC in the Question 7 above is revolved about the side 5 cm, then find the volume of the solid so obtained. Find also the ratio of the volumes?
- A heap of wheat is in the form of a cone whose diameter is 10.5 m and height is 3 m. Find its volume. The heap is to be covered by canvas to protect it from rain. Find the area of the canvas required.
| Class: 9 | Mathematics |
| Chapter: 11 | Exercise 11.3 |
| Chapter Name: | Surface Areas and Volumes |
| Mode: | Online Text and Video Format |
| Session: | CBSE 2025-26 |
| Medium: | English and Hindi Medium |
A right circular Cone and its Properties
As we have already mentioned above about the right circular cone. In Topic 11.3 you will study the volume of the cone. There is a massive similarity between the right circular cone and the pyramid. If we can be a little more liberal we say that a cone is another form of a pyramid.
The basic and only difference is that pyramid has a polygon (a square, pentagon or other) base, whereas, a right circular cone has a circular base. The tip of the cone is called the vertex or apex and the curved side is known as the lateral surface. Before diving deep into details, you must be comfortable with the concept of the volume of a cylinder.
Formula for the Volume of Cone
If you are not well versed with the concept of cylinder, you might find it a little difficult to understand. Though, you have already studied about the surface area of a right circular cone. Here is the formula of the cone V = 1/3 πr²h and surface area formula is πr² + πrl. The thing to notice here, r, h, and l, shows different a measurement.
The r shows the radius of the base of the cone, h shows the height and l is for slant height of the cone. More specifically, the height determined by that line which goes from the middle of the base to the vertex. For the slant height, you can imagine the line that goes from the vertex of the cone to the edge of the base.
Application of Formula of Volume of Cone
If all the dimensions are given for any particular question, just put the value in the formula to get the answer. In most of the questions you have to solve the volume and surface area equation by replacing the measurement in formulae variables.
Questions based on volumes are easier than that of surface areas. To understand the concept completely you have to compare it with cylinder-like objects. It is done in the figure of explanation 9th Mathematics Ex. 11.3 also. This will show you a clear picture of how there is a difference between height and slant height.
Finding Volume of Cone in Exercise 11.3
To understand the derivation of these formulae one must see the activity. If you fill the cone with liquid and emptied in the cylinder, it will take three times to fill the cylinder with the liquid.
That is why the volume of cone is just one-third of the cylinder. The cylinder has a parallel side and congruent base that makes it bigger than the cone. However, the slant height is only used in the pyramid and cone. There are certain facts to be remembered – there are two distinct types of cones, the right circular conical and the irregular one. Get here 9th Maths Exercise 11.3 NCERT (https://ncert.nic.in/) Solutions in simplified format.
It is based on the placement of the apex in combination with the base. The entire space that is covered by this type of 3D object is determined by the volume of the right circular cone.
Is exercise 11.3 of class 9 Maths difficult?
The questions in chapter 11 exercise 11.3 of class 9 Maths tries to combine different concepts to receive an adequate answer for the given shapes. Questions are easy to solve and understand. The solutions of ex 11.3 would help students to enhance their skill and knowledge to combine various concepts. The entire exercise is based on measure the volume and space with given measurements.
How can we prepare exercise 11.3 of 9th Maths NCERT?
There is well explained solution of class 9 Maths exercise 11.3 on the Tiwari Academy website. Here you can receive detailed step-by-step directions to solve the questions. Make sure you understand the concepts and formulas that are relevant to the exercise 11.3. Before solving the questions, write down the given information and try to organize it in a way that makes sense to you. Later on implement the values in formula and solve the equation.
What are the basic concepts to practice in exercise 11.3 of chapter 11 class 9 Maths?
Exercise 11.3 of Chapter 11 in class 9 Maths NCERT solutions would likely involve concepts from the topic of mensuration, which is the study of the measure of geometric shapes and figures. Some concepts that may be covered in exercise 11.3 are Area, perimeter, Volume, and surface area of Right-angled or right circular cones. You will also be asked to implement the Pythagorean Theorem here.