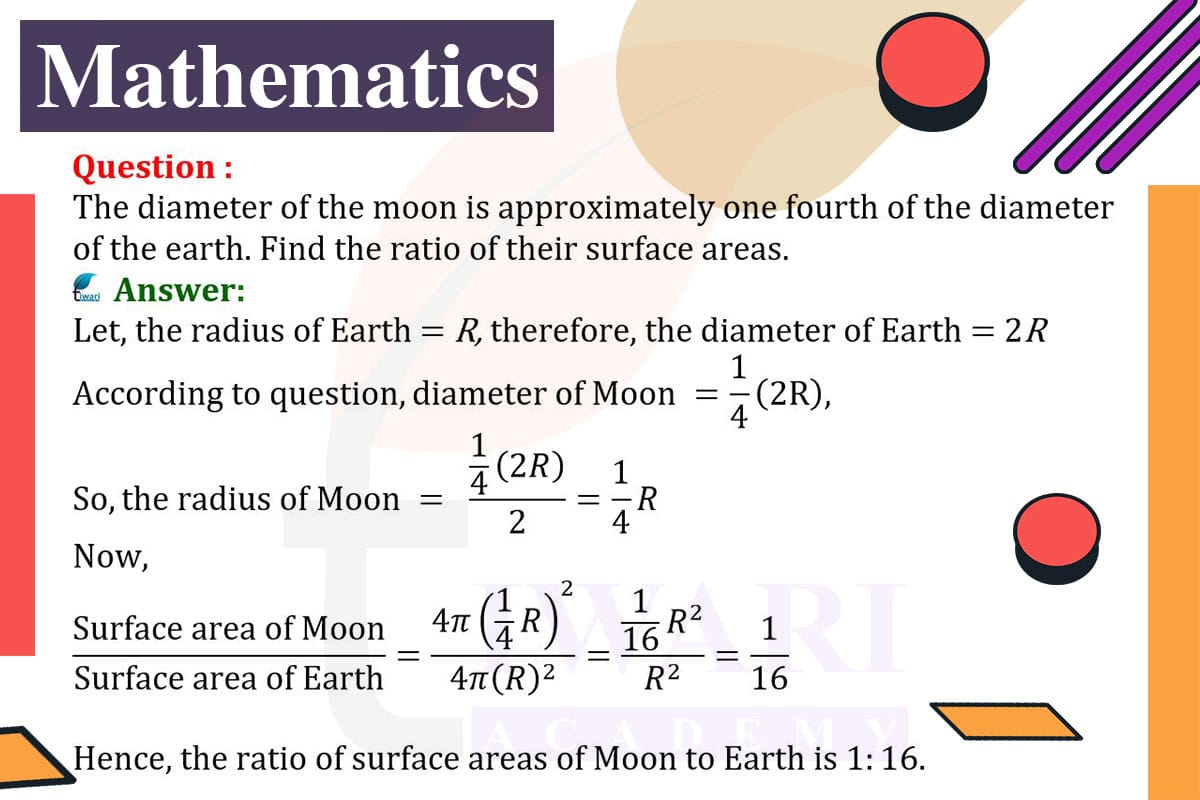
To find the ratio of the surface areas of the Moon and the Earth, given that the diameter of the Moon is approximately one fourth of the Earth’s diameter, we use the formula for the surface area of a sphere: 4πr². The surface area is directly proportional to the square of the radius (or diameter).
If the Earth’s diameter is D, the Moon’s diameter is (1/4)D.
The ratio of their radii is also 1/4.
Squaring this ratio for the surface areas, we get (1/4)² = 1/16.
Therefore, the ratio of the surface areas of the Moon to the Earth is 1:16.
This means the Earth’s surface area is 16 times larger than the Moon’s. This calculation illustrates how a smaller diameter significantly reduces the surface area, a concept important in understanding celestial bodies’ sizes and comparisons.
Let’s discuss in detail
Comparative Surface Areas of Celestial Bodies
The task at hand involves comparing the surface areas of the Moon and the Earth, based on the known ratio of their diameters. This comparison is not just a fascinating exercise in astronomy but also a practical application of geometric principles. The Moon’s diameter is approximately one fourth that of the Earth. Understanding the relationship between the diameters of these celestial bodies and their surface areas involves applying the formula for the surface area of a sphere, which is crucial in the field of astronomy and space science.
Understanding the Diameter Ratio
The diameter of the Moon is stated to be about one fourth of the Earth’s diameter. This ratio is significant because it sets the stage for understanding the relative sizes of these two celestial bodies. In terms of their diameters, if the Earth’s diameter is represented as D, then the Moon’s diameter is (1/4)D. This simple ratio has profound implications when we consider the surface areas of these spherical bodies.
The Formula for Surface Area of Spheres
The surface area of a sphere is calculated using the formula 4πr², where r is the radius of the sphere. Since the radius is half the diameter, the surface area is directly proportional to the square of the radius (or diameter). This relationship means that any change in the diameter of a sphere has a squared effect on its surface area, a key concept in understanding how the surface areas of the Earth and Moon compare.
Calculating the Ratio of Surface Areas
To find the ratio of the surface areas of the Moon and the Earth, we square the ratio of their diameters. Since the diameter ratio is 1/4, the surface area ratio becomes (1/4)², which equals 1/16. This calculation reveals that the surface area of the Earth is 16 times larger than that of the Moon. This significant difference in surface area is a direct result of the squared relationship between diameter and surface area.
Implications of the Surface Area Ratio
The 1:16 ratio of the Moon’s surface area to the Earth’s highlights how a relatively small difference in diameter can lead to a substantial difference in surface area. This concept is crucial in astronomy and planetary science, where understanding the relative sizes of celestial bodies is important for studying their physical properties, climates, and potential for supporting life. The ratio also has implications in understanding how solar radiation and heat are absorbed and reflected by these bodies.
This exercise in comparing the surface areas of the Moon and the Earth underscores the importance of mathematical principles in understanding our universe. The ability to calculate and compare the surface areas of celestial bodies based on their diameters is a fundamental aspect of astronomy and space exploration. It also illustrates the broader application of geometry in real-world contexts, demonstrating how mathematical concepts can be used to gain insights into the natural world, from the smallest particles to the vastness of space.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.2 in Detail

