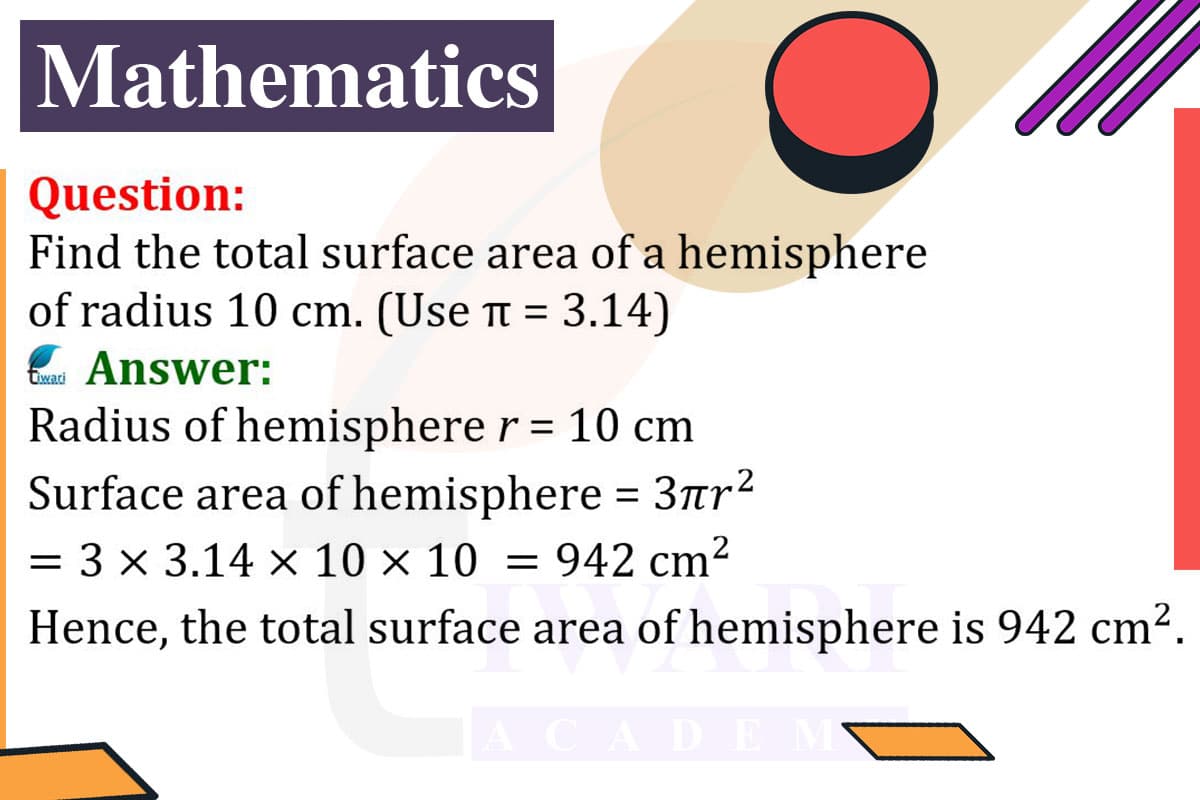
To find the total surface area of a hemisphere with a radius of 10 cm, we need to consider both the curved surface area and the base area. The curved surface area of a hemisphere is half of the surface area of a full sphere, which is 2πr².
For a radius (r) of 10 cm, this is 2 × 3.14 × 10² = 628 cm².
The base area, a circle, is πr² = 3.14 × 10² = 314 cm².
Adding these together, the total surface area is 628 + 314 = 942 cm².
This calculation is crucial for applications requiring material estimation or surface coverage of hemispherical objects.
Let’s discuss in detail
Introduction to Hemisphere Surface Area Calculation
The calculation of the total surface area of a hemisphere is a fundamental concept in geometry, essential for various practical applications. A hemisphere is half of a sphere, and its surface consists of a curved part and a flat circular base. In this task, we calculate the total surface area of a hemisphere with a radius of 10 cm. This involves determining the area of both the curved surface and the base. The formula used for these calculations is a combination of the formulas for the surface area of a sphere and the area of a circle.
Understanding the Geometry of a Hemisphere
A hemisphere is a three-dimensional shape resulting from slicing a sphere into two equal halves. It has a curved surface identical to half of the sphere’s surface and a flat circular base at the cut. The radius of the hemisphere is the same as the radius of the original sphere. In our case, the radius is 10 cm. The total surface area of the hemisphere is the sum of the curved surface area and the area of the base.
Calculating the Curved Surface Area
The curved surface area of a hemisphere is half of the surface area of a full sphere. The formula for the surface area of a sphere is 4πr². Therefore, the curved surface area of the hemisphere is 2πr². For a radius of 10 cm, this calculation becomes 2 × 3.14 × 10², which equals 628 cm². This area represents the outer part of the hemisphere that curves from the base to the top.
Determining the Base Area
The base of the hemisphere is a circle with the same radius as the hemisphere. The area of a circle is given by the formula πr². For our hemisphere with a radius of 10 cm, the base area is 3.14 × 10², which equals 314 cm². This area is the flat circular part of the hemisphere that forms the base when the sphere is cut in half.
Calculating the Total Surface Area
To find the total surface area of the hemisphere, we add the curved surface area and the base area. The curved surface area is 628 cm², and the base area is 314 cm². Therefore, the total surface area of the hemisphere is 628 + 314 = 942 cm². This total surface area is crucial for applications that require material estimation for covering or decorating hemispherical objects.
Understanding the calculation
Understanding the calculation of the total surface area of a hemisphere is essential in various practical scenarios, such as in architecture, design, and manufacturing. This calculation is particularly important when determining the amount of material needed for covering or painting hemispherical surfaces. The ability to accurately calculate surface areas based on radius is a valuable skill in many fields, including engineering and construction. This exercise demonstrates the importance of geometry in real-world applications and highlights the need for precise calculations in planning and design processes.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.2 in Detail

