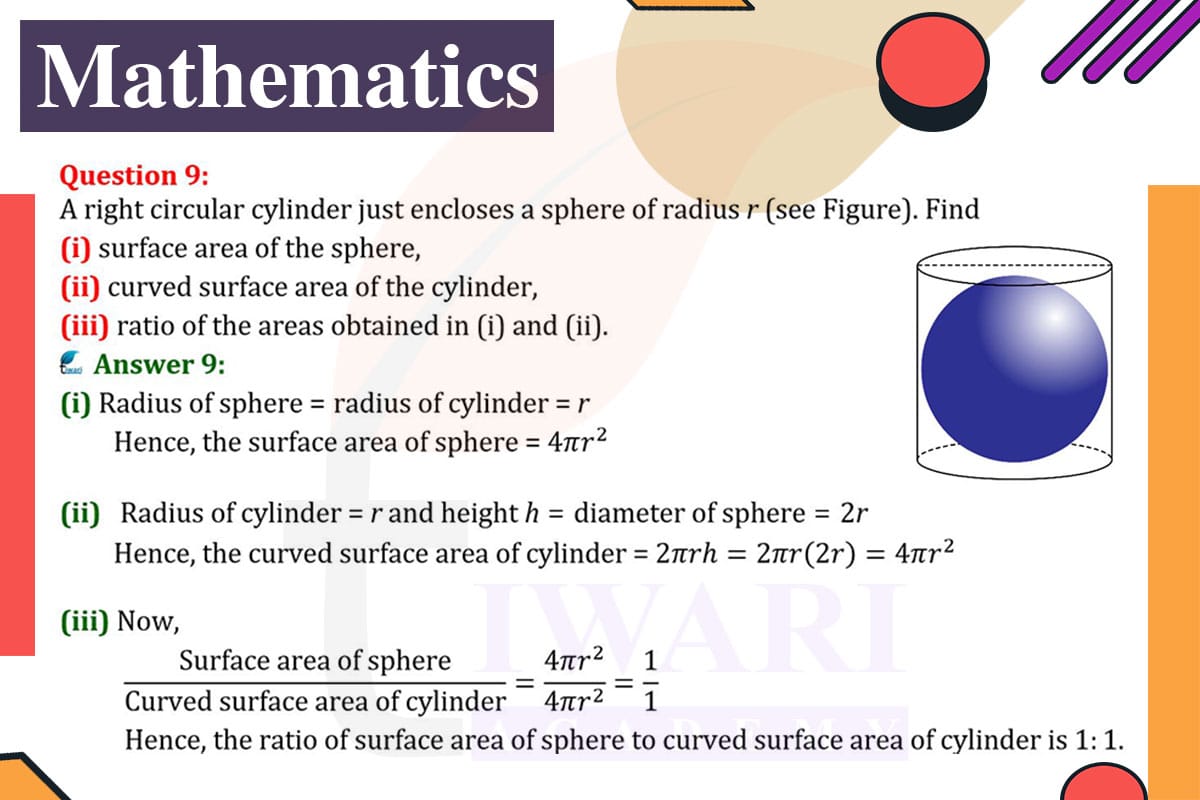
A right circular cylinder just encloses a sphere of radius r. Find (i) surface area of the sphere, (ii) curved surface area of the cylinder, (iii) ratio of the areas obtained in (i) and (ii).

Let’s discuss in detail

This problem explores the intriguing geometric relationship between a sphere and a right circular cylinder that perfectly encloses it. Both shapes share a common radius, r, and this shared dimension is key to understanding their surface area relationship. The task is to calculate the surface area of the sphere, the curved surface area of the cylinder, and then determine the ratio of these areas. This comparison offers insight into the proportional dimensions and surface areas of these fundamental geometric shapes.

The surface area of a sphere is determined by the formula 4πr², where r is the radius of the sphere. This formula encapsulates how the surface area of a sphere is proportional to the square of its radius. In the context of this problem, the sphere’s radius is the defining measurement for both the sphere itself and the cylinder that encloses it. The calculation of the sphere’s surface area is straightforward yet fundamental in understanding the sphere’s geometric properties.

The curved surface area of a right circular cylinder is calculated using the formula 2πrh, where r is the radius and h is the height. In this unique case, the cylinder’s height is equal to the diameter of the sphere, which is 2r. Substituting these values into the formula, the curved surface area of the cylinder becomes 2πr(2r) or 4πr². This calculation reveals that the curved surface area of the cylinder is identical to the surface area of the sphere.

The ratio of the surface areas of the sphere and the cylinder is found by comparing their respective surface areas. Since both the sphere’s surface area and the cylinder’s curved surface area are calculated to be 4πr², their ratio is 1:1. This means that the sphere and the cylinder have equal surface areas, a fascinating and perhaps unexpected result given their different shapes. This ratio is a testament to the elegant symmetry and proportionality inherent in geometric relationships.

The fact that a sphere and its enclosing cylinder have equal surface areas when the cylinder’s height equals the sphere’s diameter has interesting implications. This equality is a unique property of spheres and cylinders and does not generally hold for other shapes. It highlights the special geometric relationship between these two shapes and is a concept that finds relevance in various fields, including physics, engineering, and design. This equality is particularly intriguing because it defies the intuitive expectation that different shapes would have different surface areas.

This exploration of the surface areas of a sphere and its enclosing cylinder illustrates a fundamental principle in geometry: the elegant relationships between different shapes. The discovery that these two distinct shapes have equal surface areas when certain conditions are met is a beautiful example of the surprises and insights geometry can offer. Such relationships are not only mathematically interesting but also have practical applications in design, manufacturing, and scientific research, where understanding the properties of shapes and their relationships is crucial. This example underscores the beauty and utility of geometric principles in understanding the world around us.

Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.2 in Detail