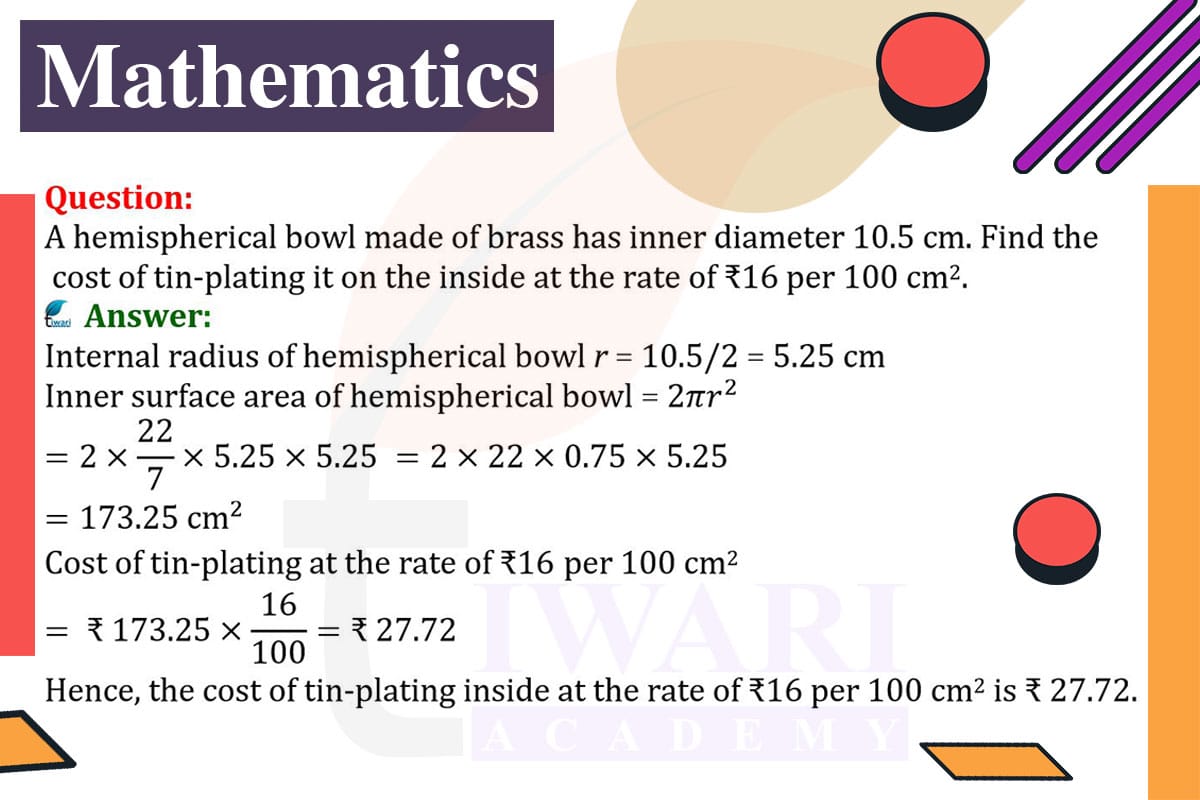
To find the cost of tin-plating the inside of a hemispherical brass bowl with an inner diameter of 10.5 cm, we first calculate the inner surface area and then determine the cost based on the given rate.
The radius of the hemisphere is half the diameter, so it’s 10.5 cm / 2 = 5.25 cm.
The surface area of a hemisphere is 2πr². Substituting the radius, we get 2 × 3.14 × 5.25² ≈ 173.55 cm².
The cost for tin-plating per 100 cm² is ₹16. Therefore, for 173.55 cm², the cost is (173.55/100) × 16 ≈ ₹27.77.
Thus, the total cost for tin-plating the inside of the bowl is approximately ₹27.77.
Tin-Plating a Hemispherical Bowl
The task involves calculating the cost of tin-plating the interior of a hemispherical brass bowl, a process that requires precise measurement of the bowl’s surface area and an understanding of the cost per unit area for tin-plating. The bowl in question has an inner diameter of 10.5 cm. Tin-plating is a common method used to coat and protect metal surfaces, often enhancing durability and resistance to corrosion. The cost calculation is based on the given rate of ₹16 per 100 cm², making it a practical application of geometry in a real-world scenario.
Determining the Radius of the Hemisphere
The first step in the calculation involves determining the radius of the hemispherical bowl. The radius is half of the diameter, so for a bowl with a diameter of 10.5 cm, the radius is 10.5 cm / 2, which equals 5.25 cm. This radius is a crucial measurement as it is used in the formula to calculate the surface area of the hemisphere, which in turn is needed to determine the cost of tin-plating.
Calculating the Surface Area of the Hemisphere
The surface area of a hemisphere is given by the formula 2πr², where r is the radius. Substituting the radius of 5.25 cm into this formula, the calculation becomes 2 × 3.14 × 5.25². This results in an area of approximately 173.55 cm². This surface area represents the total interior surface of the bowl that requires tin-plating.
Understanding the Cost Calculation
The cost of tin-plating is given as ₹16 per 100 cm². To find the total cost for the surface area of the bowl, this rate must be applied to the calculated surface area. This involves converting the cost rate to a per cm² basis and then multiplying it by the total surface area of the hemisphere. Such calculations are common in manufacturing and material costing, where surface treatments are charged based on the area covered.
Computing the Total Cost of Tin-Plating
To compute the total cost, the surface area of 173.55 cm² is divided by 100 cm² (the area for which the cost rate is given) and then multiplied by the cost rate of ₹16. This calculation is (173.55/100) × 16, which equals approximately ₹27.77. This figure represents the total cost for tin-plating the entire inner surface of the hemispherical brass bowl.
This exercise demonstrates the practical application of geometric principles in everyday scenarios, such as calculating the cost of material treatments based on surface area. Understanding how to calculate surface areas and apply unit costs is essential in various fields, including manufacturing, construction, and crafts. The ability to perform such calculations accurately ensures efficient material usage and cost-effectiveness, which are crucial in both industrial and artisanal contexts. This example highlights the relevance of geometry in solving real-world problems and in the understanding of spatial properties of common objects.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.2 in Detail

