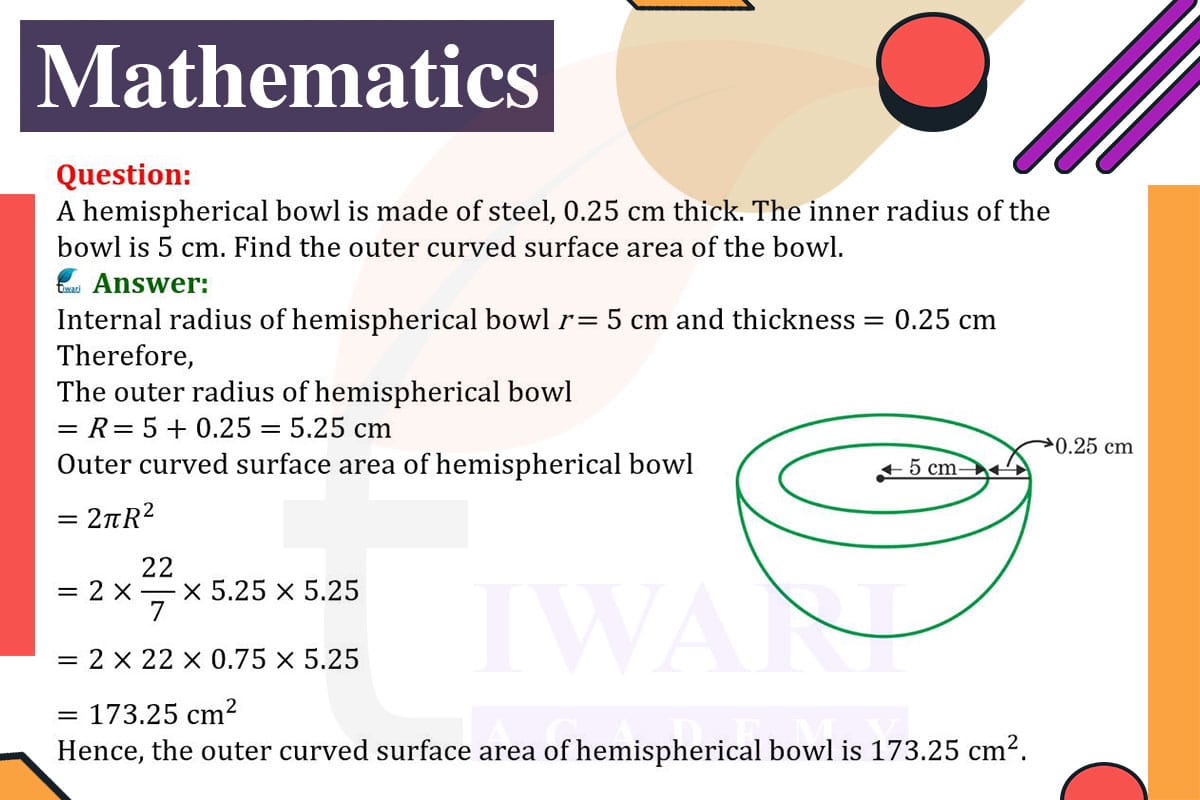
To find the outer curved surface area of a hemispherical steel bowl with an inner radius of 5 cm and a thickness of 0.25 cm, we first determine the outer radius of the bowl. The outer radius is the sum of the inner radius and the thickness of the bowl, which is 5 cm + 0.25 cm = 5.25 cm.
The formula for the curved surface area of a hemisphere is 2πr², where r is the radius.
Substituting the outer radius, the calculation becomes 2 × 3.14 × 5.25², which equals approximately 173.55 cm². This area represents the external surface of the bowl that is exposed. This calculation is essential in applications like material cost estimation, heat transfer analysis in engineering, and even in artistic design considerations.
Let’s discuss in detail
Calculating Curved Surface Area
The task involves calculating the outer curved surface area of a hemispherical steel bowl, a common problem in applied geometry with practical implications in manufacturing and design. The bowl has an inner radius of 5 cm and a steel thickness of 0.25 cm. This calculation is not just a theoretical exercise but also has real-world applications, such as determining the amount of material needed for the bowl’s production or the surface area available for external treatments like painting or coating.
Understanding the Hemispherical Bowl’s Dimensions
The bowl in question is hemispherical, meaning it is half of a sphere. Its inner radius is given as 5 cm. However, the bowl is made of steel with a thickness of 0.25 cm. This thickness is crucial because it affects the overall outer dimensions of the bowl. The outer radius, which is needed to calculate the outer surface area, is larger than the inner radius by the thickness of the material used to make the bowl.
Calculating the Outer Radius
To find the outer radius of the bowl, we add the thickness of the steel to the inner radius. The inner radius is 5 cm, and the steel thickness is 0.25 cm. Therefore, the outer radius is 5 cm + 0.25 cm = 5.25 cm. This outer radius is a key measurement as it determines the extent of the bowl’s outer surface, which is what we are interested in calculating.
Applying the Surface Area Formula
The formula for the curved surface area of a hemisphere is 2πr², where r is the radius. This formula derives from the surface area formula of a sphere, considering that a hemisphere is half of a sphere. To find the outer curved surface area of the bowl, we use the outer radius in this formula. This calculation will give us the area of the steel that forms the external part of the bowl.
Performing the Calculation
Substituting the outer radius of 5.25 cm into the formula, the calculation for the outer curved surface area becomes 2 × 3.14 × 5.25². This equals approximately 173.55 cm². This value represents the total area of the steel on the outside of the bowl. Such calculations are essential in various practical scenarios, such as determining the amount of paint needed for coating or the cost of the material used in the bowl’s production.
This exercise in calculating the outer curved surface area of a hemispherical bowl demonstrates the practical application of geometry in everyday objects. Understanding how to calculate surface areas of complex shapes is crucial in fields like manufacturing, engineering, and design. It allows for precise estimation of materials and costs, and is essential for efficient design and production processes. This example highlights the importance of geometry in solving real-world problems and in the understanding of spatial properties of common objects.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.2 in Detail

