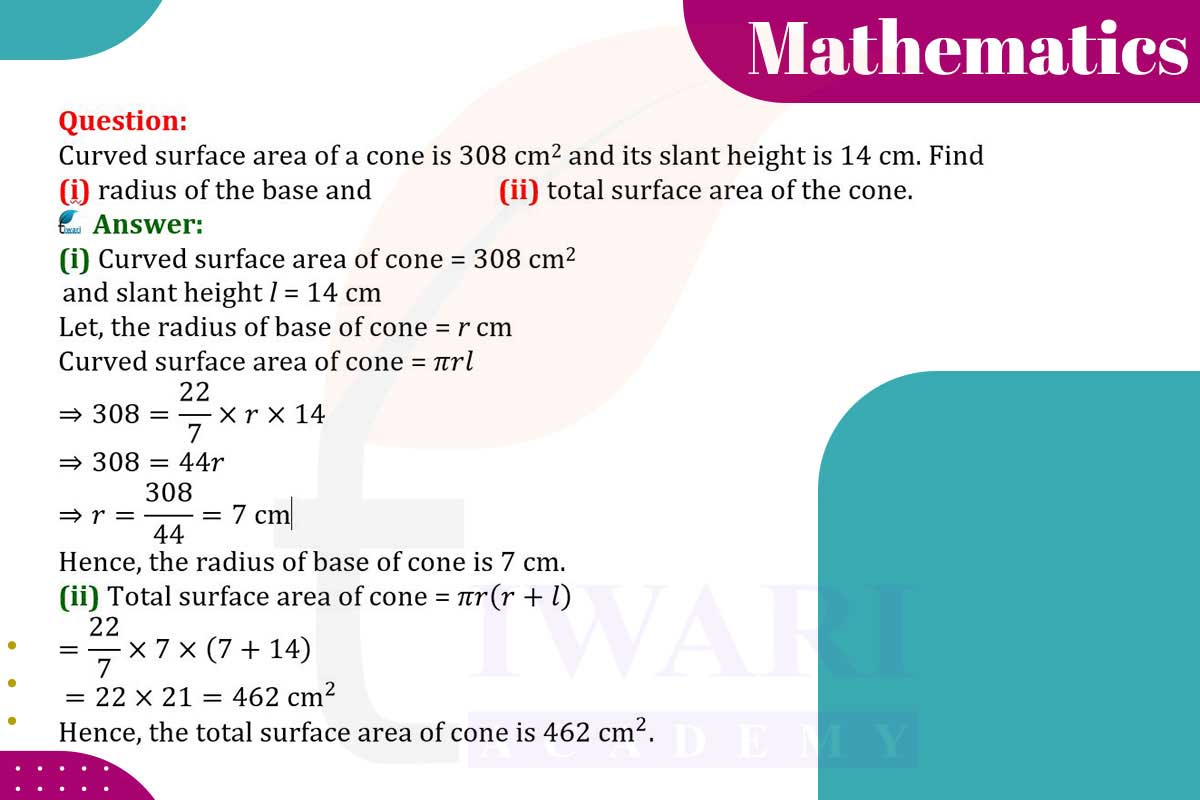
To find the radius, use the curved surface area formula πrl = 308 cm². With l = 14 cm, r = 308/14π ≈ 7 cm. For the total surface area, add the base area πr² to the curved surface area: 308 + π × 7² ≈ 308 + 153.94 = 461.94 cm²
Let’s discuss in detail
Understanding Cone Geometry and Surface Area
Cones are unique geometric shapes characterized by a circular base and a pointed top, known as the vertex. The surface area of a cone is an important concept in geometry, particularly in applications involving volume and surface calculations. The surface area of a cone is divided into two parts: the curved surface area and the base area. The curved surface area is the area of the cone’s outer surface, excluding the base, while the base area is simply the area of the circular base. Understanding how to calculate these areas is crucial in fields such as architecture, engineering, and design, where precise measurements are necessary for creating accurate models and structures.
The Formula for Curved Surface Area
The curved surface area of a cone is calculated using the formula πrl, where r is the radius of the base and l is the slant height of the cone. The slant height is the straight-line distance from the top of the cone (vertex) to the edge of the base, measured along the surface of the cone. This formula is derived from the properties of a circle and the concept of a sector, as the curved surface of a cone can be conceptualized as a circular sector that has been rolled up.
Given Problem and Initial Data
In the given problem, we are told that the curved surface area of a cone is 308 cm², and its slant height is 14 cm. Our task is to determine two things: (i) the radius of the base of the cone, and (ii) the total surface area of the cone. The total surface area includes both the curved surface area and the area of the base. This problem requires us to apply our understanding of the cone’s geometry and the formulas for surface area calculation.
Calculating the Radius of the Base
To find the radius of the base, we rearrange the formula for the curved surface area to solve for r. The formula πrl = 308 cm² becomes r = 308/14π. Substituting the given values, we find that the radius r is approximately 7 cm. This calculation is crucial as the radius is a key component in determining the total surface area of the cone.
Determining the Total Surface Area
Once we have the radius, we can calculate the total surface area of the cone. The total surface area is the sum of the curved surface area and the base area. The base area is calculated using the formula for the area of a circle, πr². Therefore, the total surface area is 308 cm² + π × 7² cm². This calculation combines both aspects of the cone’s surface, providing a comprehensive understanding of its total area.
Final Results and Practical Implications
After performing the calculations, we find that the total surface area of the cone is approximately 461.94 cm². This result is significant in practical scenarios where precise material estimations are needed, such as in manufacturing or construction. Understanding how to calculate the surface area of a cone is not only crucial for academic purposes but also has real-world applications in various technical and creative fields. The ability to accurately determine these measurements can lead to more efficient designs and better utilization of materials.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.1 in Detail

