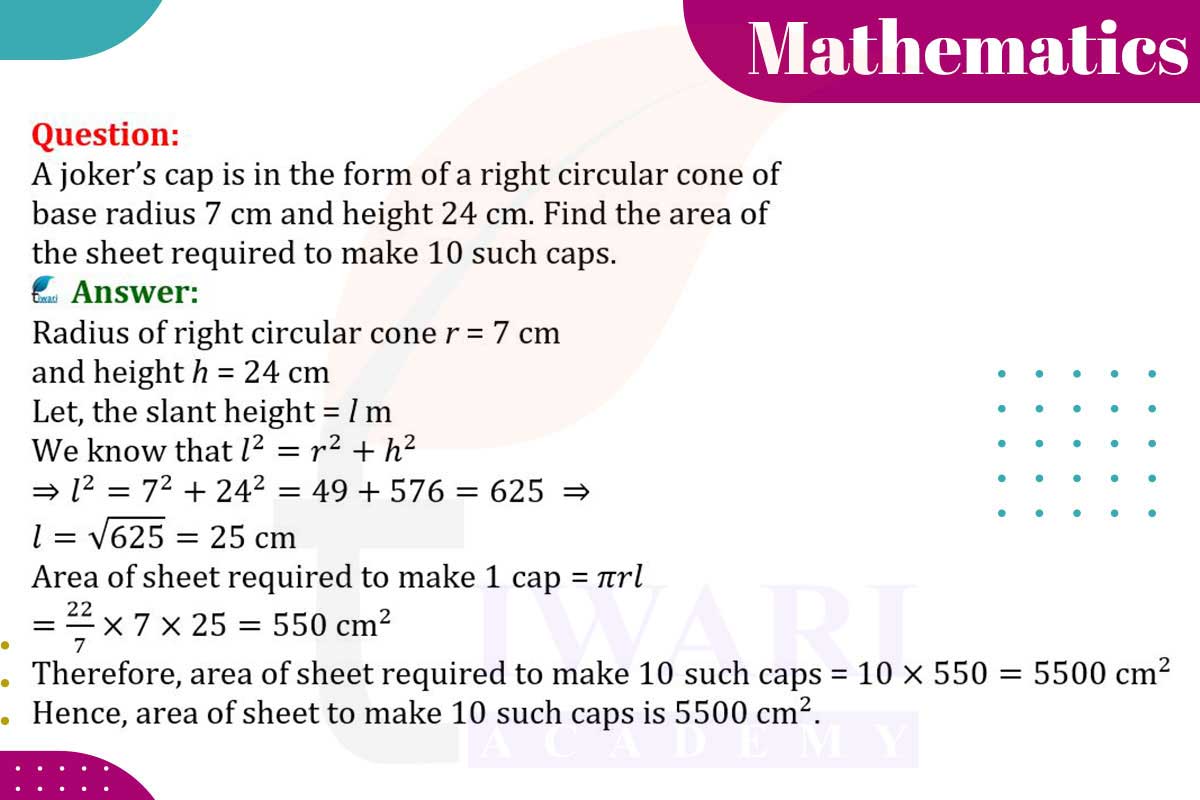
To find the area of the sheet required to make 10 joker’s caps, each shaped as a right circular cone with a base radius of 7 cm and a height of 24 cm, we first calculate the slant height using Pythagoras’ theorem: l = √[h² + r²] = √[7² + 24²] = √[49 + 576] = √[625] = 25 cm. The area of the sheet for one cap is the curved surface area of the cone, given by πrl = 3.14 × 7 × 25 ≈ 549.5 cm². For 10 caps, the total area is 10 × 549.5 = 5495 cm².
Let’s discuss in detail
Introduction to Crafting Joker’s Caps
The task at hand involves calculating the area of material needed to create 10 joker’s caps, each fashioned into the shape of a right circular cone. These caps, often seen in costumes and festive attire, have specific dimensions: a base radius of 7 cm and a height of 24 cm. The process of determining the required material area combines concepts of geometry with practical applications in design and manufacturing, showcasing how mathematical principles are applied in everyday creative processes.
Understanding Cone Geometry
A right circular cone, the shape of the joker’s cap, is a three-dimensional geometric figure with a circular base and a pointed top, known as the vertex. The height of the cone is the perpendicular distance from the base to the vertex, while the slant height is the length of the line segment from the vertex to any point on the circumference of the base. The slant height is crucial for calculating the curved surface area, which represents the material needed to make the cap.
Calculating the Slant Height
The slant height of the cone is a key dimension in determining the amount of material required. It can be calculated using Pythagoras’ theorem, which states that in a right-angled triangle, the square of the hypotenuse (slant height) is equal to the sum of the squares of the other two sides (radius and height of the cone). For our joker’s cap, the slant height (l) is calculated as l = √[7² + 24²] = √[49 + 576] = √[625] = 25 cm.
Determining the Curved Surface Area
The curved surface area of the cone is the area of the sheet required to make one joker’s cap. This area is calculated using the formula for the surface area of a cone, πrl, where r is the radius and l is the slant height. Substituting the values for our joker’s cap, we get 3.14 × 7 × 25, which equals approximately 549.5 cm². This is the area of material needed for one cap.
Calculating Material for Multiple Caps
To find the total area of the material required for 10 caps, we simply multiply the area needed for one cap by 10. This straightforward multiplication gives us the total area of material needed for the entire batch of caps. Therefore, the total area for 10 joker’s caps is 10 × 549.5 = 5495 cm². This calculation is essential for efficient material usage and cost estimation in the production process.
Conclusion and Practical Application
This exercise in calculating the material required for joker’s caps illustrates the practical application of geometric principles in design and manufacturing. Accurate calculations ensure efficient use of materials, minimizing waste and optimizing production costs. Such skills are invaluable in fields like costume design, event planning, and manufacturing, where precision and efficiency are key. This example underscores the relevance of geometry in creative and practical endeavors, bridging the gap between abstract mathematical concepts and their real-world applications.
Discuss this question in detail or visit to Class 9 Maths Chapter 11 for all questions.
Questions of 9th Maths Exercise 11.1 in Detail

