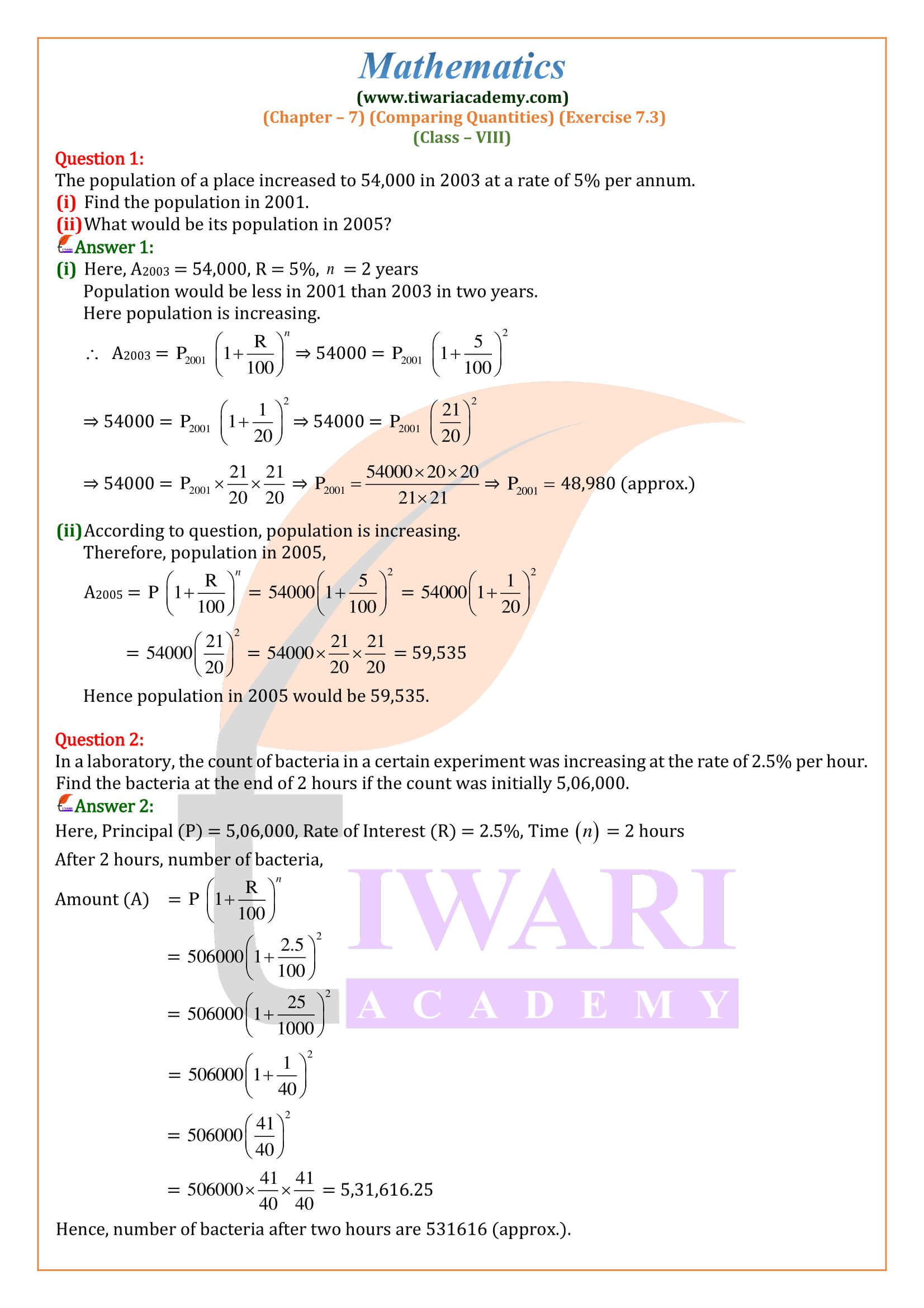
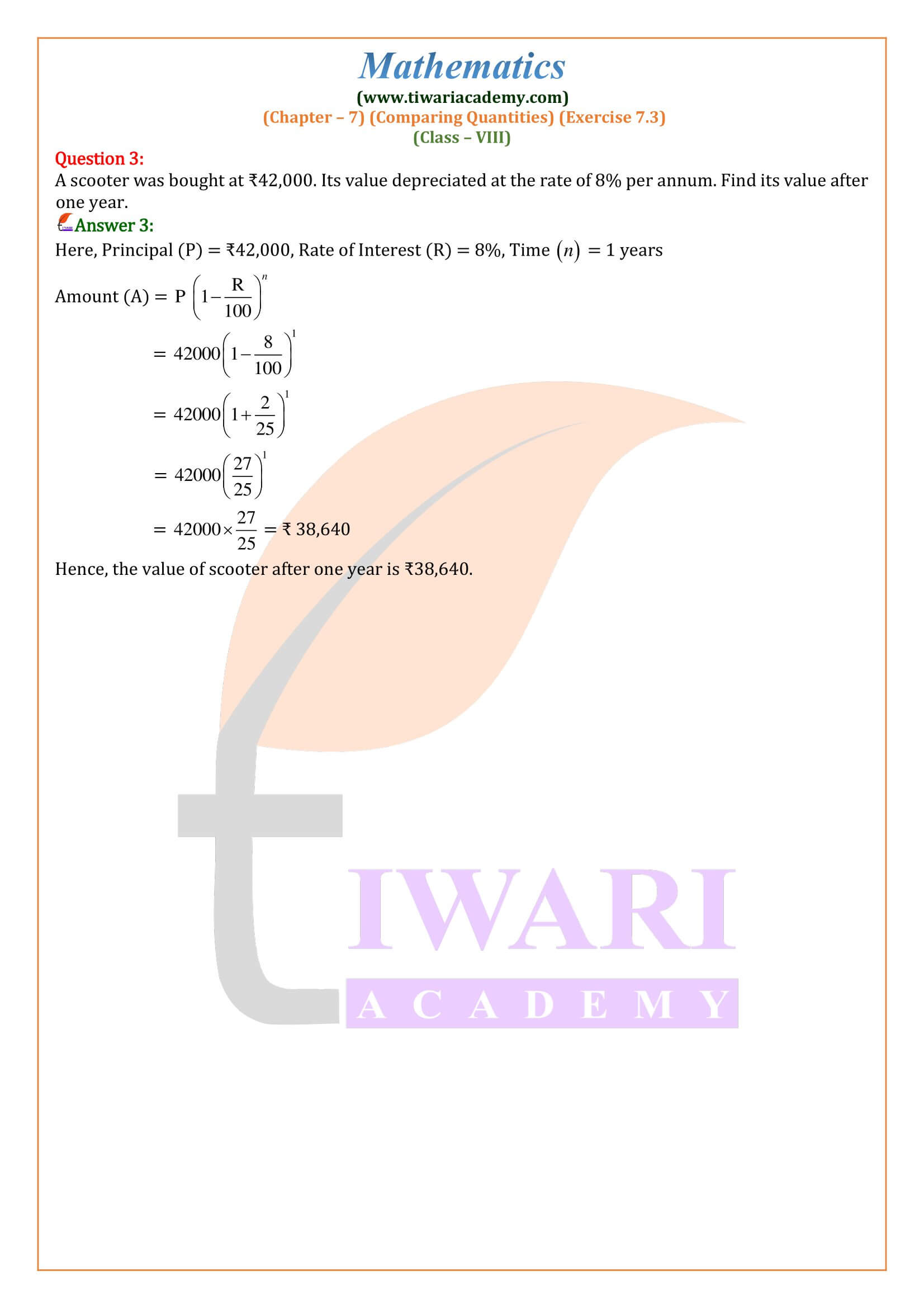
CBSE NCERT Solutions for Class 8 Maths Chapter 7 Exercise 7.3 Comparing Quantities in Hindi and English Medium updated for new session 2025-26. All the questions given in ex. 7.3 of 8th Maths are revised according to new syllabus and revised NCERT books for 2025-26 examination.
8th Maths Exercise 7.3 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 7 | Exercise: 7.3 |
| Chapter Name: | Comparing Quantities |
| Content: | NCERT Exercise Solution |
| Content Type: | Text and Videos Format |
| Session: | CBSE 2025-26 |
| Medium: | Hindi and English |
Class 8 Maths Chapter 7 Exercise 7.3 Solution
Class VIII Mathematics NCERT chapter 7 Ex. 7.3 Comparing Quantities updated for CBSE and State board students appearing in 2025-26 exams. All the exercises are in Hindi and English Medium free to use online or download in PDF file format. Videos related to exercise 7.3 is also given here, so that students can easily understand each questions. If someone is facing problem to access NCERT textbook solutions or other contents on Tiwari Academy, please contact us for help.

Interest
Interest on capital is the interest paid to owners for providing a firm with the required capital to start a business. It is similar to obtaining a loan from any financial institution. The partners are paid interest on the capital that remains outstanding.
Simple interest
When money is borrowed on simple interest then the interest is calculated uniformly on the original principal throughout the loan period.
S. I. = P X R X T 100
To Find Compound Interest When Interest Is Compounded Annually
In such cases where interest is compounded yearly, the interest accrued during the first year is added to the principal and the amount so obtained becomes the principal for the second year. The amount at the end of the second year becomes the principal for the third year, and so on.
Find the compound interest on 25000 for 3 years at 10% per annum, compounded annually.
Principal for the first year = 25000.
S. I. = (P X R X T)/100
Interest for the first year = (25000 x 10 x 1)/100 = Rs. 2500
Amount at the end of the first year = (25000 + 2500) = Rs. 27500.
Principal for the second year = 27500.
Interest for the second year = (27500 x 10 x 1)/100 = Rs. 2750
Amount at the end of the second year = (27500 + 2750) = 30250.
Principal for the third year = 30250.
Interest for the third year = (30250 x 10 x 1)/100 = Rs. 3025
Amount at the end of the third year = (30250 + 3025) = 33275.
Compound interest = (33275 – 25000) = 8275.
To Find Compound Interest When Interest is Compounded Half- Yearly
In such cases, if the rate of interest is R% per annum then it is clearly % per half-year. The amount alter the first half-year becomes the principal for the next half-year, and so on. The method for calculating the compound interest in such cases is shown in the example given below.
Find the compound interest on 5000 for 1 year at 8% per annum, compounded half-yearly.
Rate of interest = 8% per annum = 4% per half-year.
Time = 1 year = 2 half-years.
Original principal = 5000.
Interest for the first half-year = Rs. (5000 x 4 x 1)/100 = Rs. 200
Amount at the end of the first half-year = (5000 + 200) = 5200.
Principal for the second half-year = 5200.
Interest for the second half-year = Rs. (5200 x 4 x 1)/100 = Rs. 208.
Amount at the end of the second half-year = (5200 + 208) = 5408
Compound interest = (5408 – 5000) = 408.
To Find Compound Interest When Interest is Compounded Quarterly
If the rate of interest is R% per annum and the interest is compounded quarterly, then the rate is R/4% per quarter.
Find the compound interest on 8000 for 1 year at 20% per annum, interest being payable quarterly.
Principal = 8000
Rate of Interest = 20% per annum = 20/4% = 5% per quarter
Time = 1 year = 4 quarters
Interest for 1st quarter = Rs. (8000 x 5 x 1)/100 = Rs. 400
Amount at the end of 1st quarter = (8000 + 400) = 8400.
Principal for 2nd quarter = 8400.
Interest for 2nd quarter = Rs. (8400 x 4 x 1)/100 = 420.
Amount at the end of 2nd quarter = (8400 + 420) = 8820.
Principal for 3rd quarter = 8820
Interest for 3rd quarter = Rs. (8820 x 5 x 1)/100 = 441.
Amount at the end of 3rd quarter = (8820 + 441) = 9261.
Principal for 4th quarter = 9261
Interest for 4th quarter = Rs. (9261 x 5 x 1)/100 = 463.05.
Amount at the end of 4th quarter = (9261 + 463.05) = 9724.05.
So, Compound Interest = (9724.05 – 8000) = 1724.05
Calculating Compound Interest by Using Formulae
The method of calculating the compound interest discussed previously is quite long and cumbersome, especially when the time period is large.
We can derive general formulae for calculating compound interest in various cases, as given below. It is very easy to calculate compound interest by using these formulae.
When the interest is compounded annually
Let principal = P, rate = R% per annum and time = n years. Then, the amount A is given by the formula.
A = Rs. P (1 + r/100)ⁿ
Find the amount of 8000 for 3 years, compounded annually at 10% per annum. Also, find the compound interest.
Here, p = 8000, R = 10% per annum and n = 3 years
amount after 3 years = Rs. 8000 (1 + 10/100)3
= 8000 x 11/10 x 11/10 x 11/10 = 10648
Thus, amount after 3 years = 10648. And, compound interest = (10648 – 8000) = 2648.
Applications of Compound Interest Formula
In each of the following situations we use the CI formula.
- Increase (or decrease) in population.
- The growth of bacteria when the rate of growth is known.
- Depreciation in the values of machines, etc., at a given rate.
Note: For decrease (or depreciated value), we use the formula:
Decreased value (or depreciated value) = P {1 – R/100}ⁿ
Interest Compounded Half-Yearly
Let principal = P, rate = R% per annum, time = n years.
Suppose that the interest is compounded half-yearly. Then,
Rate = R/2% per half-year, time = (2n) half-years, and amount = Rs. P x (1 + R/200)2n
Compound interest = (amount) – (principal)
Interest Compounded Quarterly
Let principal = P, rate = R% per annum, time = n years.
Suppose that the interest is compounded quarterly, Then,
Rate = R/4% per half-year, time = (4n) half-years, and amount = Rs. P x (1 + R/400)4n
Compound interest = (amount) – (principal)
Unitary Method
(i) Suppose A can finish a piece of work in 8 days.
Then, work done by A in 1 day = [by unitary method].
(ii) Suppose that the work done by A is 1 day is.
Then, time taken by A to finish the whole work = 6 days.
General Rules
(i) Suppose A can finish a work in n days.
Then, work done by A in 1 day = 1/n
(ii) Suppose that the done by A in 1 day is 1/n.
Then, time taken by A to finish the whole work = n days.
Problems on Pipes and Cistern
A cistern or a water tank is connected with two types of pipes.
(i) Inlet: The pipe which fills the tank is called on inlet.
(ii) Outlet: The pipe which empties the tank is called an outlet.
Rule 1. Suppose an outlet fills a tank in n hours.
Then, part of the tank filled in 1 hour = 1/n,
i.e., work done by the inlet in 1 hour = 1/n.
Rule 2. Suppose an outlet empties a full tank in m hours.
Then, part of the tank emptied in 1 hour = 1/m
i.e., work done by the outlet in 1 hour = (-1/m)
How do we solve work time questions in exercise 7.3 of 8th Maths?
The following example explains about work time question:
A tap A can fill a cistern in 8 hours while tap B can fill it in 4 hours. In how much time will the cistern be filled if both A and B are opened together?
Explanation:
Time taken by tap A to fill the cistern = 8 hours.
Time taken by tap B to fill the cistern = 4 hours.
Work done tap A in 1 hour = 1/8
Work done by tap B in 1 hour = ¼
Work done by (A + B) in 1 hour = (1/8 + ¼) = 3/8
Time taken (A + B) to fill the cistern = 8/3 hours = 2 hours 40 min.
Is compound interest questions given in exercise 7.3 of class 8 Maths easy?
The questions based on compound interest are simple as like as simple interest. Just go through the concepts of CI and apply the formula. Do you know, what is the difference between simple interest and compound interest Why do you end up with more money with compound interest?
Why do you end up with more money with compound interest? Simple interest is interest paid only on the original investment whereas compound interest paid both on the original investment and on all interest that has been added to the original investment.
What are the application based questions of class 8 Maths exercise 7.3?
There are time work, day to day life and other questions are given in exercise 7.3 of 8th Mathematics. For example:
The population of a town was 176400 in the years 2009. It increases at the rate of 5% per annum. (i) What would be its population in the year 2011? (ii) What was its population in the year 2007?
Explanation:
Population in the year 2009 = 176400.
Rate of increase = 5% per annum.
(i) population in the year 2011 = population after 2 years
= 176400 {1 + 5/100}2
= 176400 {21/20 x 21/20}
= 194481.
Hence, the population of the town in 2011 would be 194481.
(ii) Let the population in the year 2007 be x. Then,
(ii) Let the population in the year 2007 be x. Then,
population in 2009 = x (1 + 5/100)2
x (21/20 x 21/20) = (441/400) x
(441/400) x = 176400
So, x = (176400 x 400)/441 = 160000
Hence, the population of the town in 2007 was 160000.