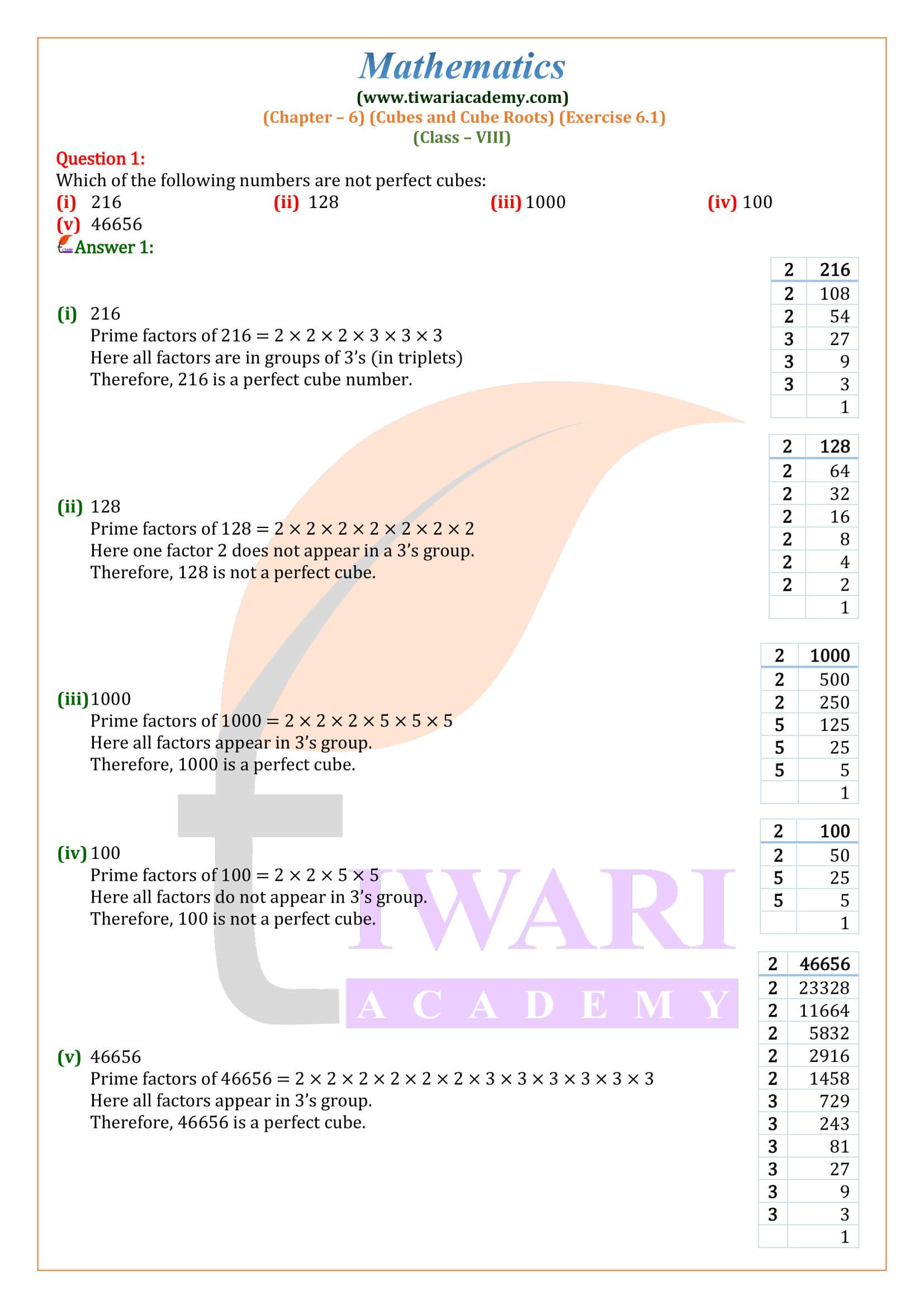
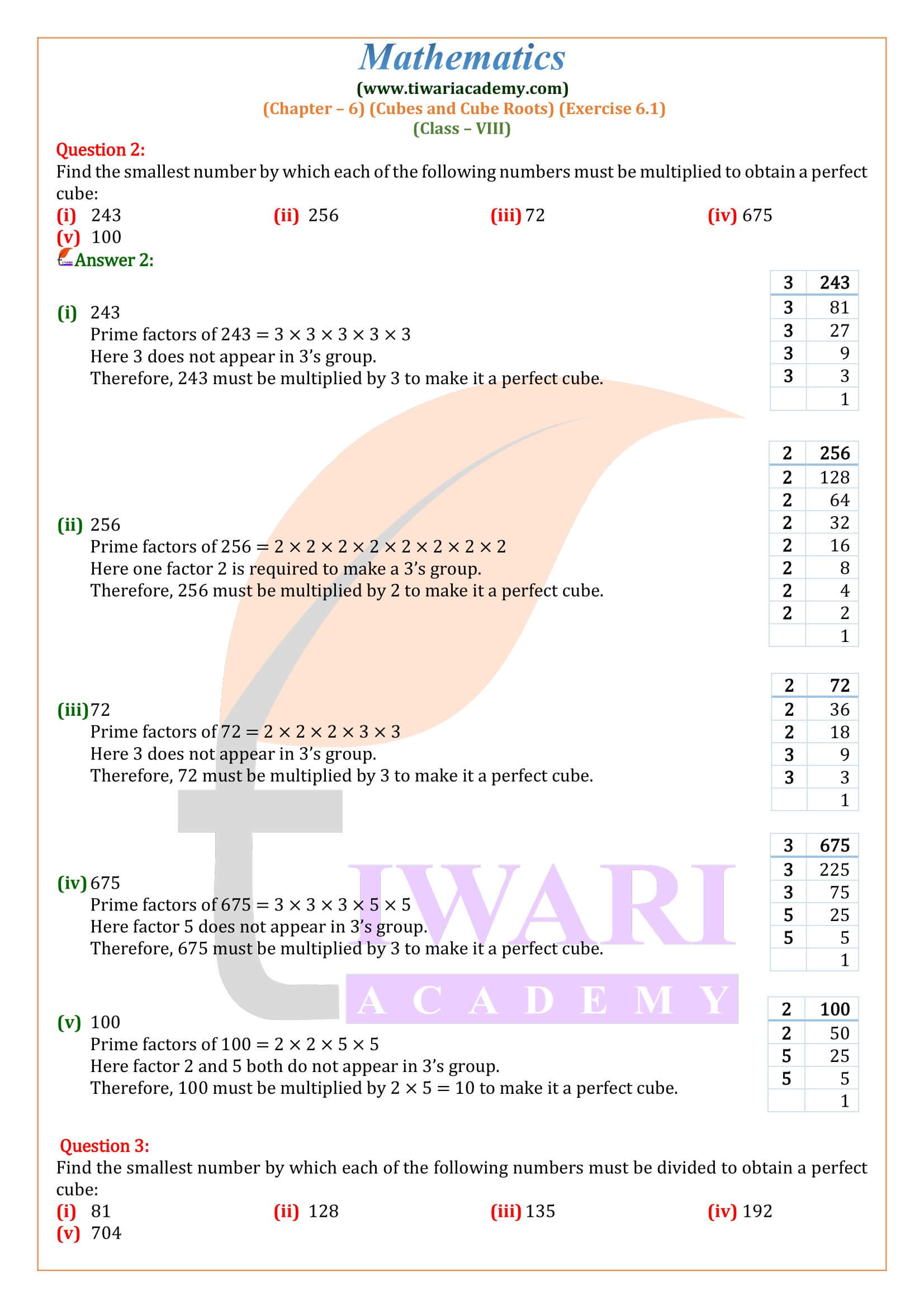
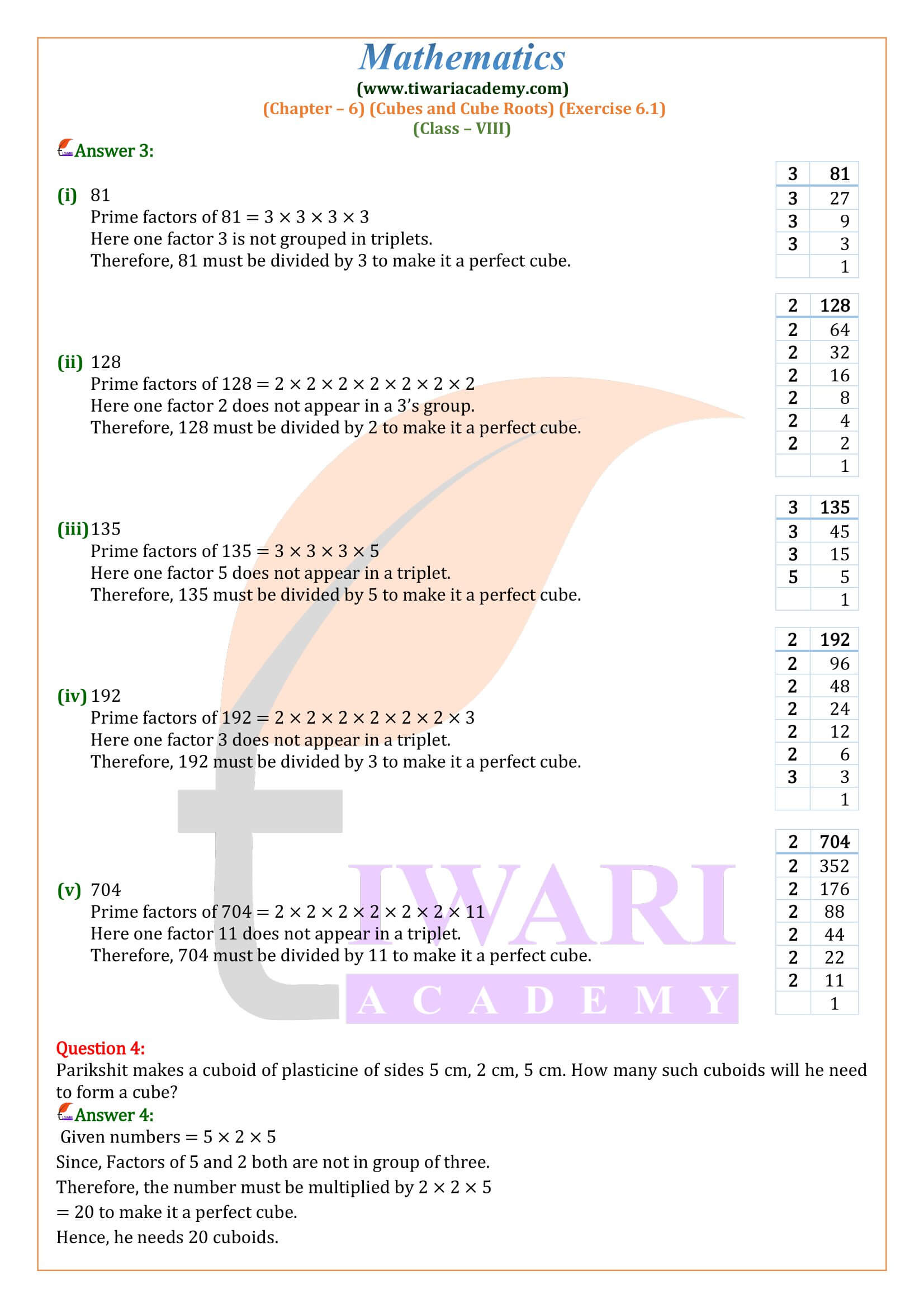
NCERT Solutions for Class 8 Maths Chapter 6 Exercise 6.1 Cube and Cube Roots in Hindi and English Medium updated for new session 2025-26. Get here the revised and modified format solutions ex. 6.1 of class 8 mathematics following the new textbooks for academic year 2025-26.
8th Maths Exercise 6.1 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 6 | Exercise: 6.1 |
| Chapter Name: | Cube and Cube Roots |
| Content Type: | Online Text and Videos |
| Session: | CBSE 2025-26 |
| Medium: | Hindi and English Medium |
Class 8 Maths Chapter 6 Exercise 6.1 Solution
Class VIII Mathematics NCERT Textbook chapter 6 Ex. 6.1 Cube and Cube Roots updated for academic session 2025-26 free to download or use online. All the contents of class 8 Maths chapter 6 is updated for CBSE and State Boards (Like UP Board, MP Board, Haryana, Rajasthan, Gujrat, and other also). Here we will learn about Cubes, Properties of cubes of numbers, Cube roots, and how to find the cube roots by factorisation method.
Cubes of a Number
We know that when a number is multiplied by itself, we get the square of that number. If the number ‘x’ is multiplied by itself, then square of x = x ´ x = x²
Similarly, if a number is multiplied by itself 3 times, we get cube of that number. If x is a number.
then, cube of x = x X x X x = x³
2³ = 2 x 2 x 2 = 8 Thus, cube of 2 is 8.
3³ = 3 x 3 x 3 = 27 Thus, cube of 3 is 27.
Perfect Cube
A natural number is said to be a perfect cube, if it is the cube of a natural number.
1³ = 1, 2³ = 8, 3³ = 27, 4³ = 64.
Thus, 1, 8, 27, 64, are perfect cubes.
Here are the cubes of first 10 natural numbers:
| Number | a x a x a = a³ | Result |
|---|---|---|
| 1 | 1 x 1 x 1 = 1³ | 1 |
| 2 | 2 x 2 x 2 = 2³ | 8 |
| 3 | 3 x 3 x 3 = 3³ | 27 |
| 4 | 4 x 4 x 4 = 4³ | 64 |
| 5 | 5 x 5 x 5 = 5³ | 125 |
| 6 | 6 x 6 x 6 = 6³ | 216 |
| 7 | 7 x 7 x 7 = 7³ | 343 |
| 8 | 8 x 8 x 8 = 8³ | 512 |
| 9 | 9 x 9 x 9 = 9³ | 729 |
| 10 | 10 x 10 x 10 = 10³ | 1000 |
Properties of Cubes of Numbers
From the above table, it is observed that:
- 1. 1, 8, 27, 64, 125, …….are the cubes of natural numbers.
- 2. The cubes of even numbers are even and the cubes of odd numbers are odd.
- 3. In a perfect cube, each prime number appears three times in its prime factorisation.
- 4. Cube of a negative number is negative. For example: (-2)³ = (-2) x (-2) x (-2) = -8, (-3)³ = (-3) x (-3) x (-3) = -27
- 5. Cubes of the numbers ending with 0, 1, 4, 5, 6 and 9, also end with the same digits.
- 6. Cubes of the numbers ending with 2 will end with 8 and cube of the numbers ending with 8 will end with 2.
- 7. Cubes of numbers ending with 3 and 7 will end with 7 and 3 respectively.
To Find Whether the Given Number is a Perfect Cube
Step 1: Write the given number as a product of its prime factors.
Step 2: Group the factors such that three identical prime factors make one group.
Step 3: If no factor is left over, then the given number is a perfect cube otherwise not.
Step 4: To find the natural number whose cube is the given number, take one number from each group and multiply them. The product of the numbers so obtained will be the required number.
Find the cube of the following number: (i) 11 (ii) 12 (iii) 30 (iv) 41
(i) Cube of 11 = (11)³ = 11 x 11 x 11 = 1331
(ii) Cube of 12 = (12)³ = 12 x 12 x 12 = 1728
(iii) Cube of 30 = (30)³ = 30 x 30 x 30 = 27000
(iv) Cube of 41 = (41)³ = 41 x 41 x 41 = 68921
What do you learn about cube root in exercise 6.1 of 8th Maths?
In class 8 Maths exercise 6.1, we learn that the cube root of a number is the factor that we multiply by itself three times to get that number. The operations of cube roots are given here in simplified manner.
What is the use of cube roots in class 8 Maths Exercise 6.1?
There are many solved and practice questions given based on cube roots. The cube root is often used to solve cubic equations. In particular, it can be used to solve for the dimensions of a three-dimensional object of a certain volume.
How do you use the concepts of exercise 6.1 of 8th Maths to find edge if the volume of a cubical box is 0.027 m?
Length of each edge
= ∛ (volume of cube)
= ∛0.027
= ∛27 /∛1000
= 3/10
Hence, the length of the edge of the cubical box is 3/10 m or 0.3 m.