NCERT Solutions for Class 8 Maths Chapter 5 Exercise 5.4 in Hindi and English Medium updated for CBSE 2025-26 exams. All question answers and explanation are revised according to new syllabus and new NCERT textbooks published for academic session 2025-26.
8th Maths Exercise 5.4 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 5 | Exercise: 5.4 |
| Chapter Name: | Square and Square Roots |
| Content Type: | Text, Images, PDF and Vidoes |
| Academic Session: | CBSE 2025-26 |
| Medium: | Hindi and English Medium |
Class 8 Maths Chapter 5 Exercise 5.4 Solution
Class VIII Mathematics NCERT textbook Ex. 5.4 of Square and Square Roots in PDF file format free to download or use online with video NCERT solution. Using prime factorisation, we can find the square root of any number. For this we have to make group of two. It should be noted that if any number or numbers are left whose grouping is not possible, then the number is not a perfect square.
Square Roots
The square root of a number x is that number which when multiplied by itself gives x² as the product. We denote the square root of a number x by √x.
Clearly. √4 = 2, √9 = 3, √16 = 4, √25 = 5, etc.
Square Root of a Perfect Square by the Prime Fractorisation Method:
When a given number is a perfect, we find its square root by the following steps:
- Resolve the given number into prime factors.
- Make pairs of similar factors.
- Take the product of the prime factors, choosing one factor out of every pair
Class 8 Maths Exercise 5.4 Extra Questions
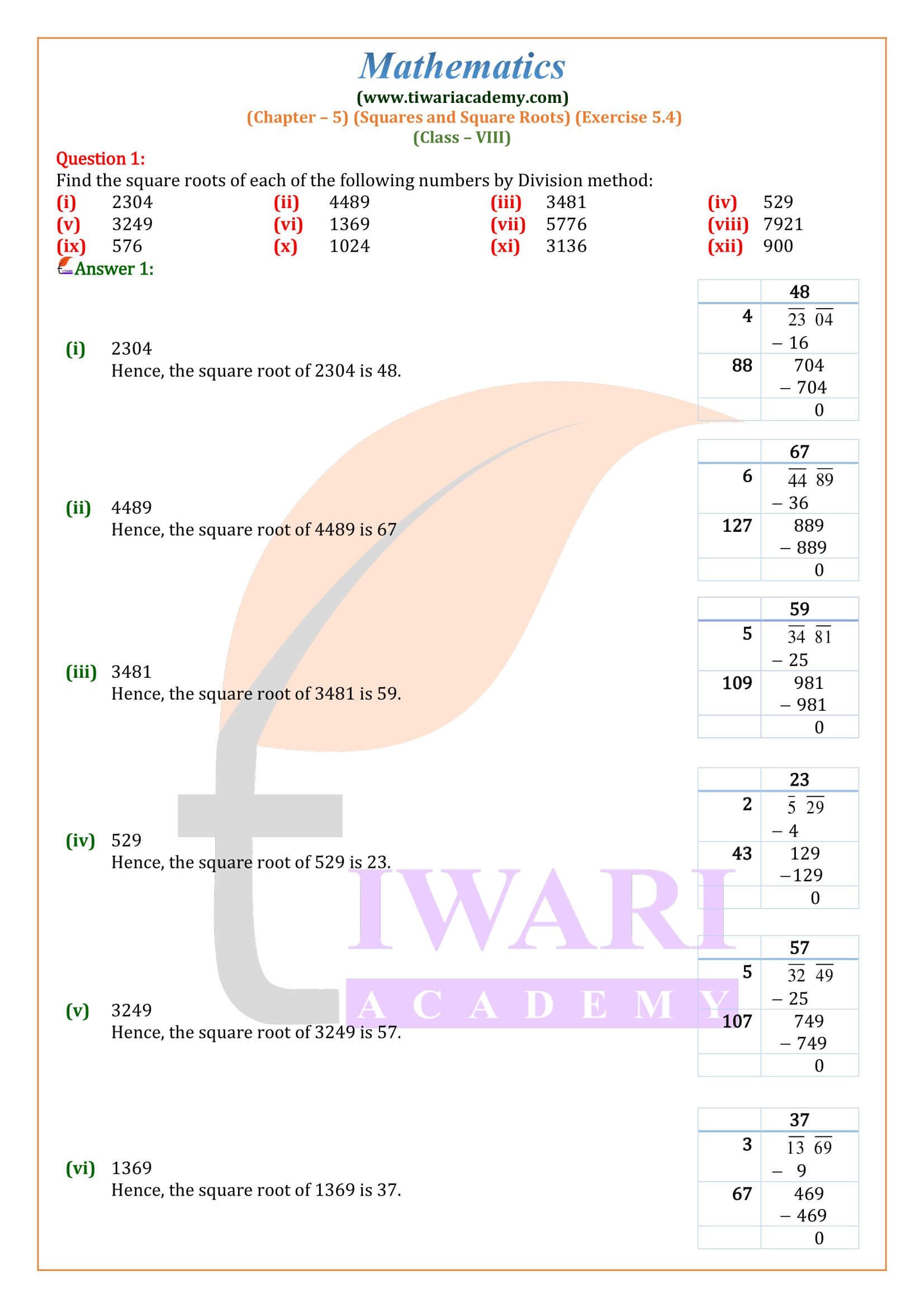
Find the square root of 324.
| 2 | 324 |
| 2 | 162 |
| 3 | 81 |
| 3 | 27 |
| 3 | 9 |
| 3 |
By prime factorisation, we get
324 = 2 x 2 x 3 x 3 x 3 x 3
So, √324 = 2 x 3 x 3 = 18
Evaluate √11025
By prime factorisation, we get
| 5 | 11025 |
| 5 | 2205 |
| 3 | 401 |
| 3 | 147 |
| 7 | 49 |
| 7 | 7 |
| 1 |
11025 = 5 x 5 x 3 x 3 x 7 x 7
So, √11025 = 5 x 3 x 7 = 105
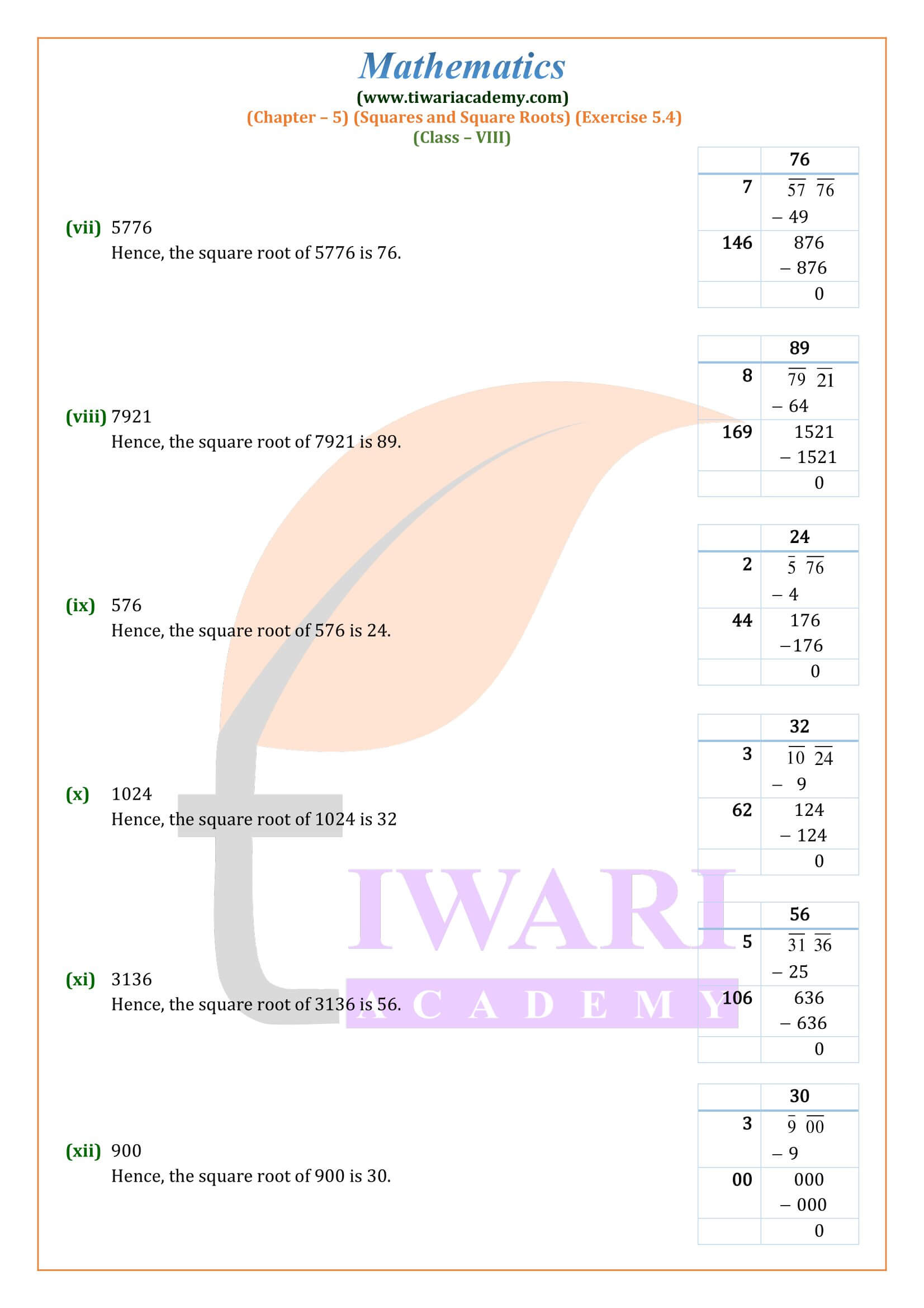
Find the square root of 784 by the long-division method.
Marking periods and using the long-division method, we have:
So, √784 = 28
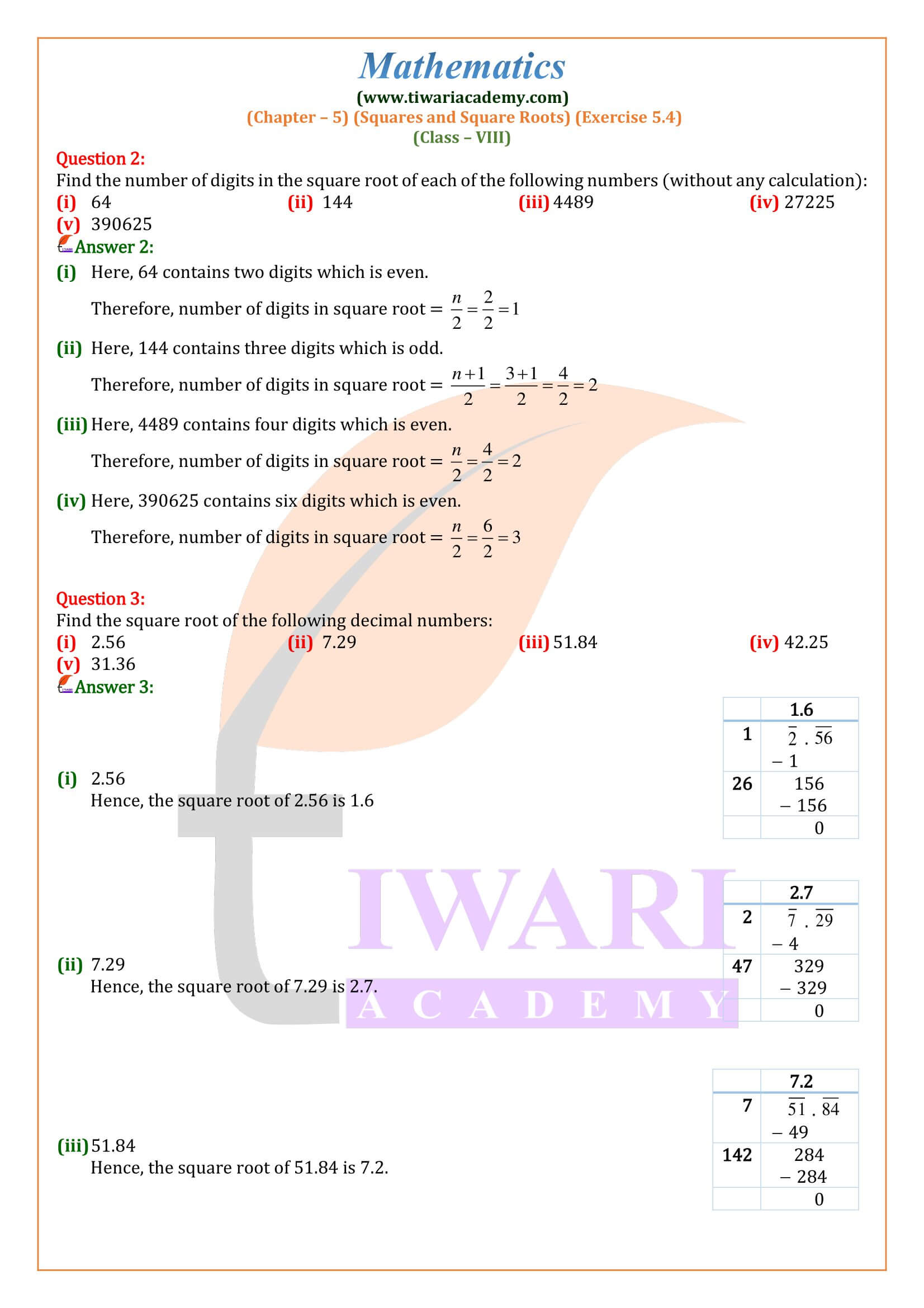
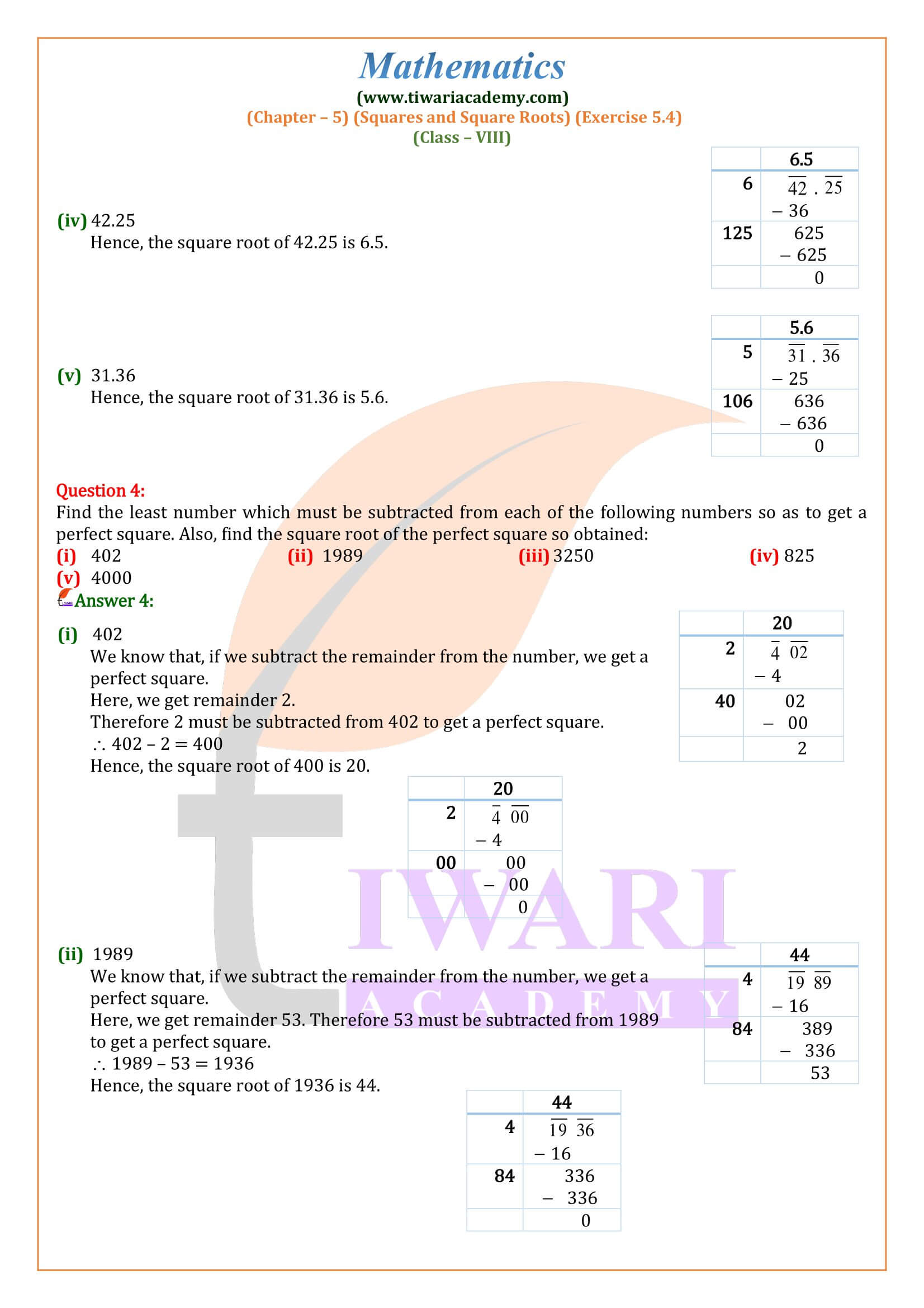
Evaluate 42.25.
Using the division method, we may find the square root of the given number as shown below:
So, √42.25 = 6.5
Square Root of a Perfect Square by The Long-Division Method
When numbers are very large, the method of finding their square roots by factorisation becomes lengthy and difficult. So, we use the long-division method is explained in the following steps.
Long-Division Method for Finding Square Roots
- Step 1: Group the digits in pairs, starting with the digit in the unit place, Each pair and the remaining digit (if any) is called a period.
- Step 2: Think of the largest number whose square is equal to or just less than the first period. Take this number as the divisor and also as the quotient.
- Step 3: Subtract the product of the divisor and the quotient from the first period and bring down the next period to the right of the remainder. This becomes the new dividend.
- Step 4: Now, the new divisor is obtained by taking two times the quotient and annexing with it a suitable digit which is also taken as the next digit of the quotient, chosen in such a way that the product of the new divisor and this digit is equal to or just less than the new dividend.
- Step 5: Repeat steps (2), (3) and (4) till all the periods have been taken up. Now, the quotient so obtained is the required square root of the given number.
Class 8 Maths Exercise 5.4 Important Questions
How do you find the square root of a decimal?
We can find the square root of decimals by converting it into rational numbers.
Examples. 1) √1.44 = √144/√100 = 12/10 = 1.2.
find square root of 37.0881.
First convert 37.0881 into a rational number and then find the square root by prime factorization or long division method.
6.08
| 6
+6 1209 |
37.0881
-36 10881 -10881 X |
How do you solve square roots manually?
First group the numbers under the root in pairs from right to left, leaving either one or two digits on the left (6 in this case). For each pairs of number you will get one digit in the square root. Square the 2, giving 4, write that underneath the 6, and subtract. Bring down the next pair of digits.
Evaluate √2 correct up to two places of decimal.
Using the division method, we may find the value of √2 as shown below.
1.414
| 1
+1 24 +4 281 +1 2824 |
2.000000
-1 100 -96 400 -281 11900 11296 604 |
So, √2 = 1.414 = 1.41 (correct up to two place of decimal)
Square Roots of Numbers in Decimal Form
Method:
Make the number of decimal places even by affixing a zero, if necessary. Now mark periods and, find out the square root by the long-division method. Put the decimal point in the square root as soon as the integral part is exhausted.
To find the value of square root correct up to certain places of decimal:
If the square root is required correct up to two places of decimal, we shall find it up to 3 places of decimal and then round it off up to two places of decimal.
Similarly, if the square root is required correct up to three places of decimal, we shall find it up to 4 places of decimal and then round it off up to three places of decimal, and so on.