NCERT Solutions for Class 8 Maths Chapter 5 Exercise 5.3 in Hindi and English medium updated for CBSE 2025-26 exams. The solutions of ex. 5.3 class 8 math are revised on the basis of new revised syllabus and latest NCERT books for academic session 2025-26.
8th Maths Exercise 5.3 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 5 | Exercise: 5.3 |
| Topic Name: | Square and Square Roots |
| Content Type: | Video and PDF Solutions |
| Session: | CBSE 2025-26 |
| Medium: | Hindi and English |
Class 8 Maths Chapter 5 Exercise 5.3 Solution
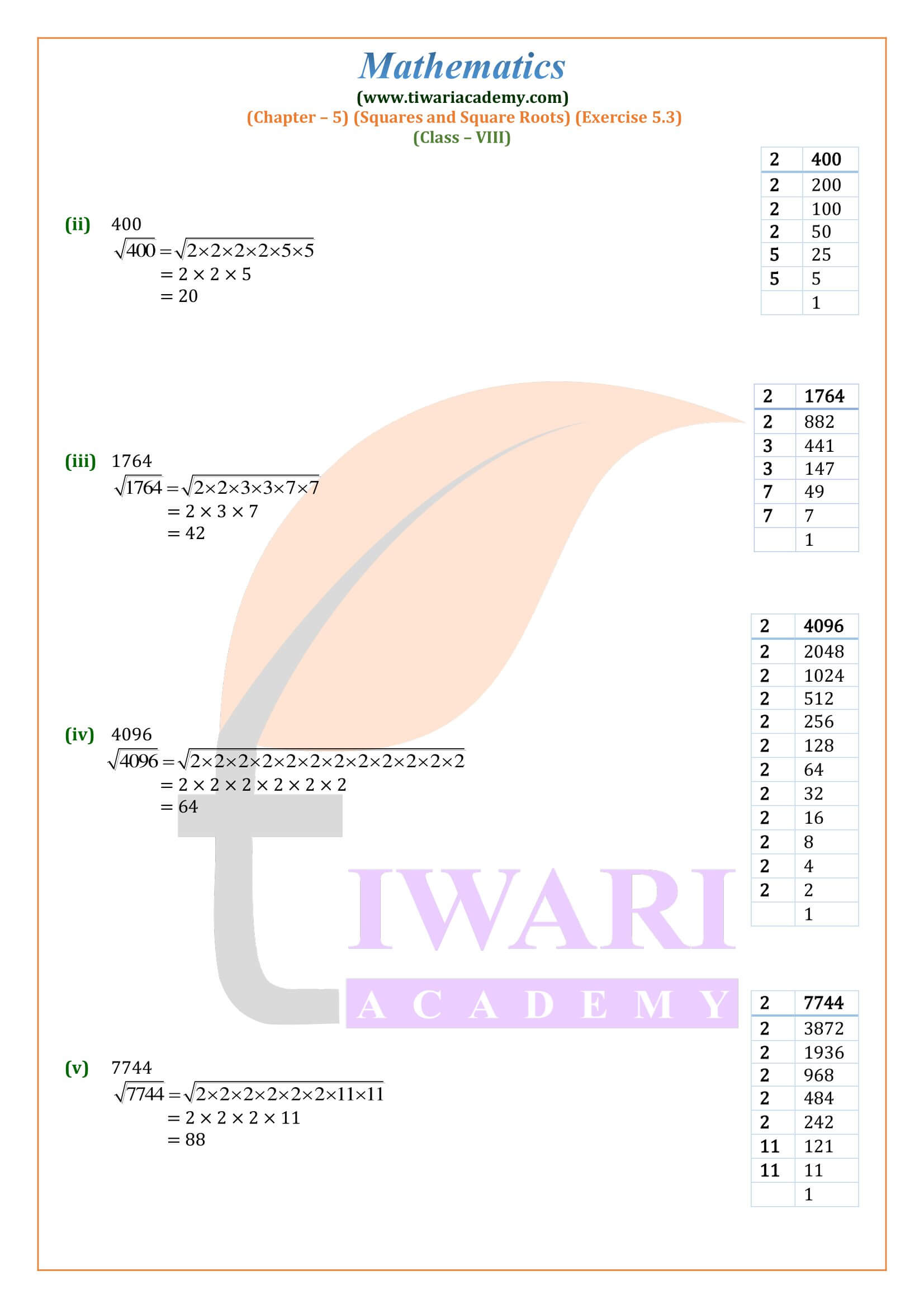
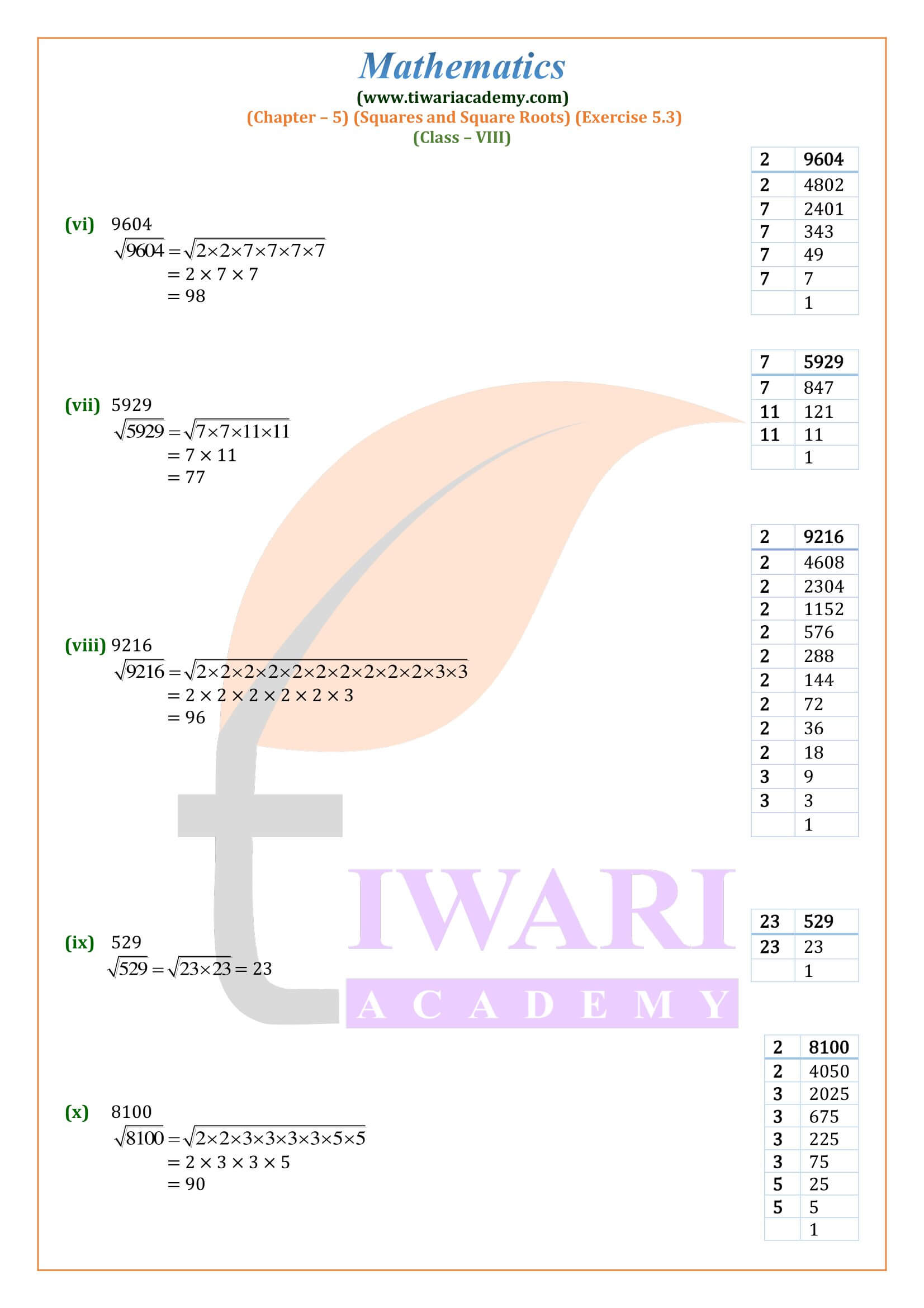
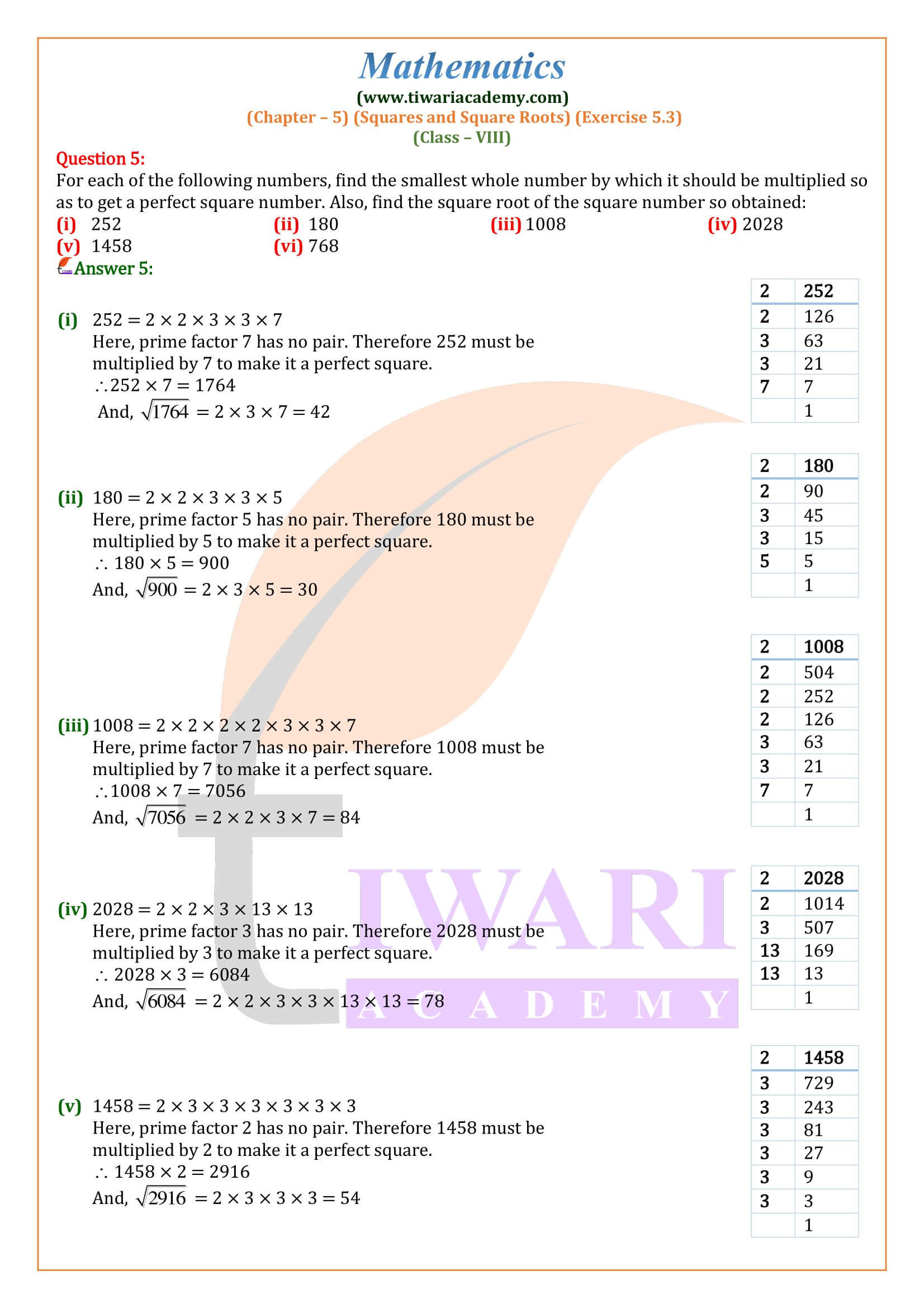
Class VIII Mathematics Ex. 5.3 of Square and Square Roots in Hindi and English free to use or download updated for 2025-26. Do you know that when the exponent (power) of a natural number is 2, the number so obtained is called a square number or a perfect square? Thus, when a number is multiplied by itself, the product is a perfect square. In 8th Maths NCERT Book exercise 5.3, we will go through the concepts of square and square roots or natural numbers.

Pythagorean Triplet
If a, b, c are three numbers where c > a and c > b such that a² + b² = c². Then (a, b, c) is called Pythagorean triplet.
Example:
(3, 4, 5), (6, 8, 10) and (5, 12, 13) and Pythagorean triplets, because
(i) (3)² + (4)² = (5)²
(ii) (6)² + (8)² = (10)²
(iii) (5)² + (12)² = (13)²
This, for any number m < 1, 2m, m² –1 and, m² + 1 is a Pythagorean triplet.
Example 1. Write a Pythagorean triplet whose one member is:
(i) 14 (ii) 16
We know that 2m, m² –1 and, m² + 1 is a Pythagorean triplet.
(i) Let 2m = 14
m = 7
So, m² – 1 = 72 – 1 = 48 and m² + 1 = 72 + 1 = 50
Hence, 14, 48, 50 is a Pythagorean triplet.
(ii) Let 2m = 16
Or, m = 8
So, m² – 1 = 82 – 1 = 63 and m² + 1 = 8² + 1 = 65
Hence, 16, 63, 65 is a Pythagorean triplet.
Short-Cut Methods for Squaring a Number
Column method for squaring a two-digit number Let the given number have the tens digit = a and the units digit = b. Then, we have to square this number.
Class 8 Maths Exercise 5.3 Extra Questions
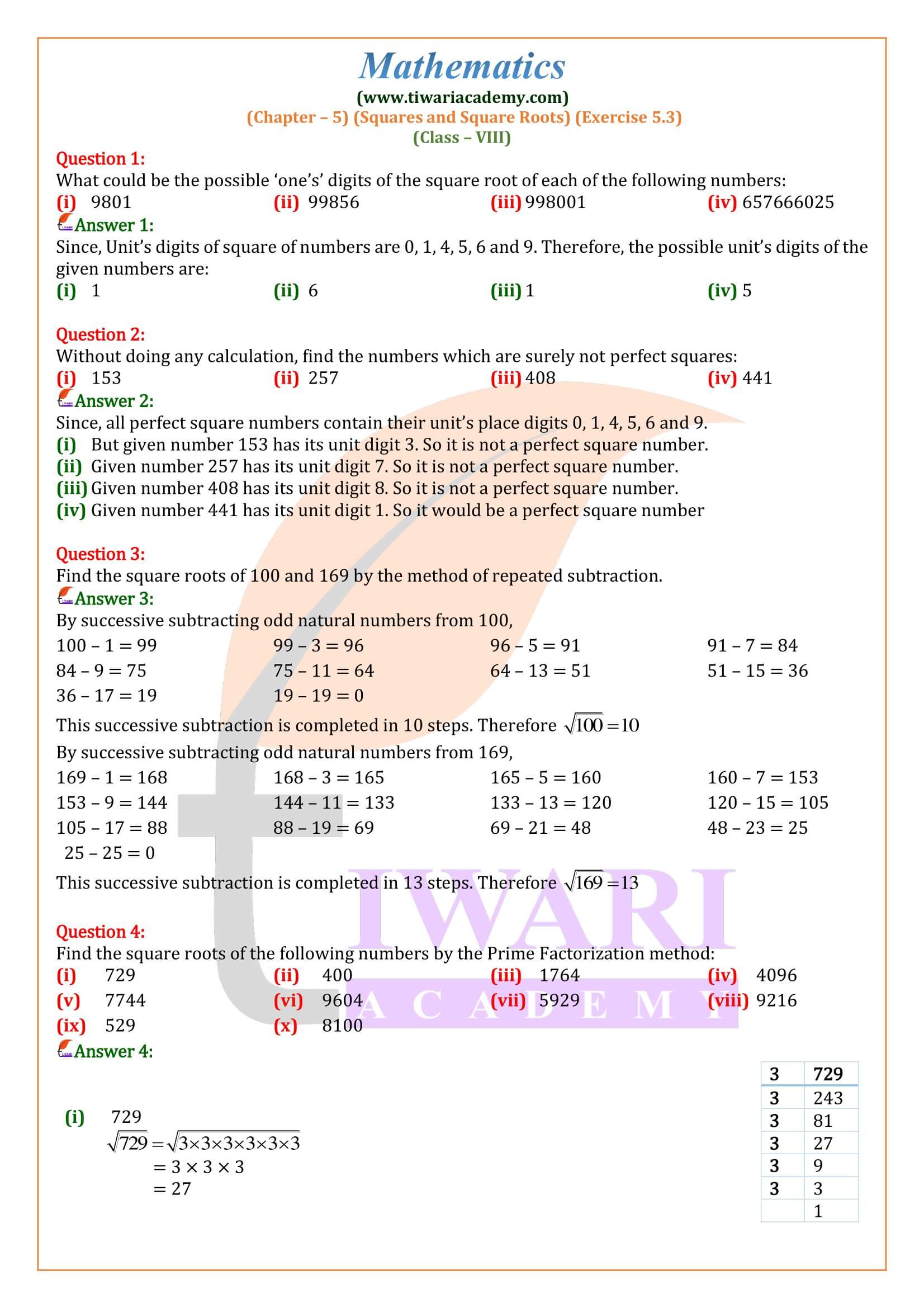
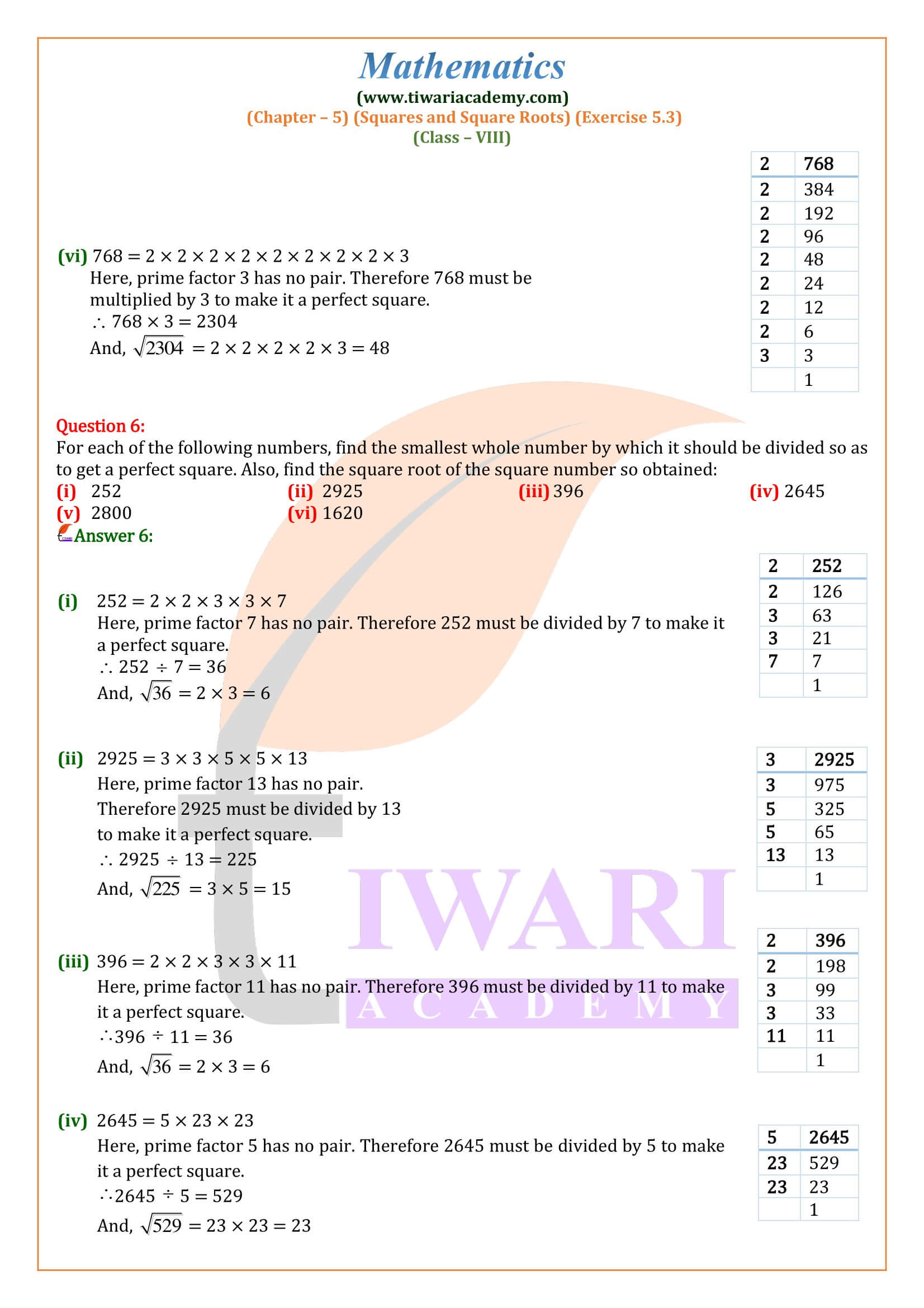
Give reason to show that none of the numbers given below is a perfect square: (i) 2162 (ii) 6843 (iii) 9637 (iv) 6598
We know that a number ending in 2, 3, 7 or 8 is never a perfect square.
Hence, none of the numbers 2162, 6843, 9637 and 6598 is a perfect square.
Give reason to show that none of the numbers 640, 81000 and 3600000 is perfect square.
We know that a number ending in an odd number of zeros is never a perfect square. So, none of the numbers 640, 81000 and 3600000 is a perfect square.
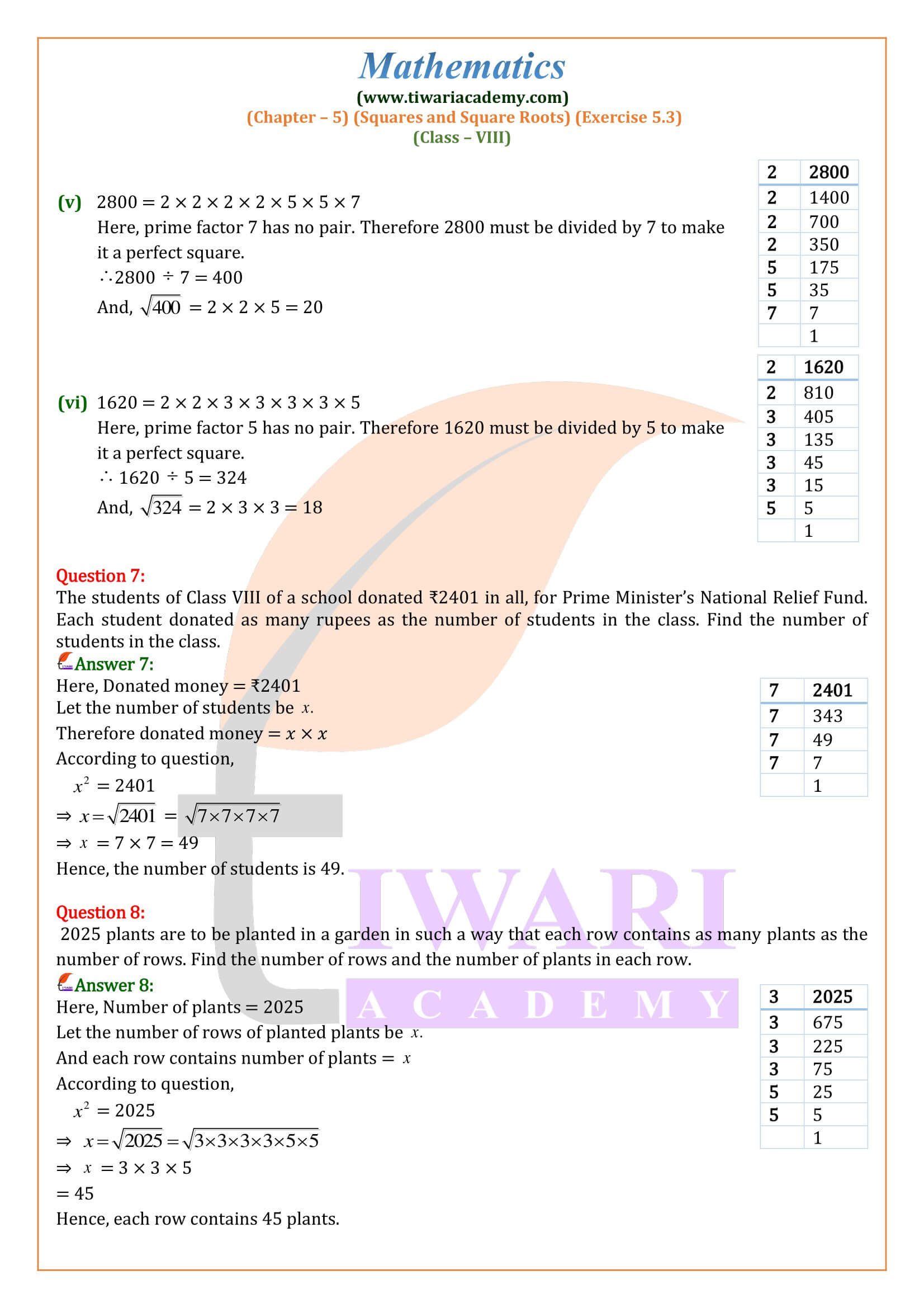
Express: (i) 64 as the sum of 8 odd natural numbers. (ii) 121 as the sum of 11 odd natural numbers.
We have
(i) 64 = 8² = sum of 8 odd natural numbers = (1 + 3 + 5 + 7 + 9 + 11 + 13 +15).
(ii) 121 = (11)² = sum of first 11 odd natural numbers = (1 + 3 + 5 + 7 + 9 + 11 + 13 +15 + 17 + 19 + 21).
Find the Pythagorean triplet whose smallest members is 12.
For every natural number m < 1, (2m, m -1, m +1) is a Pythagorean triplet. Putting 2m = 12, we get m = 6. Thus, we get the triplet (12, 35, 37). Product of Two Consecutive Odd or Consecutive Even Numbers
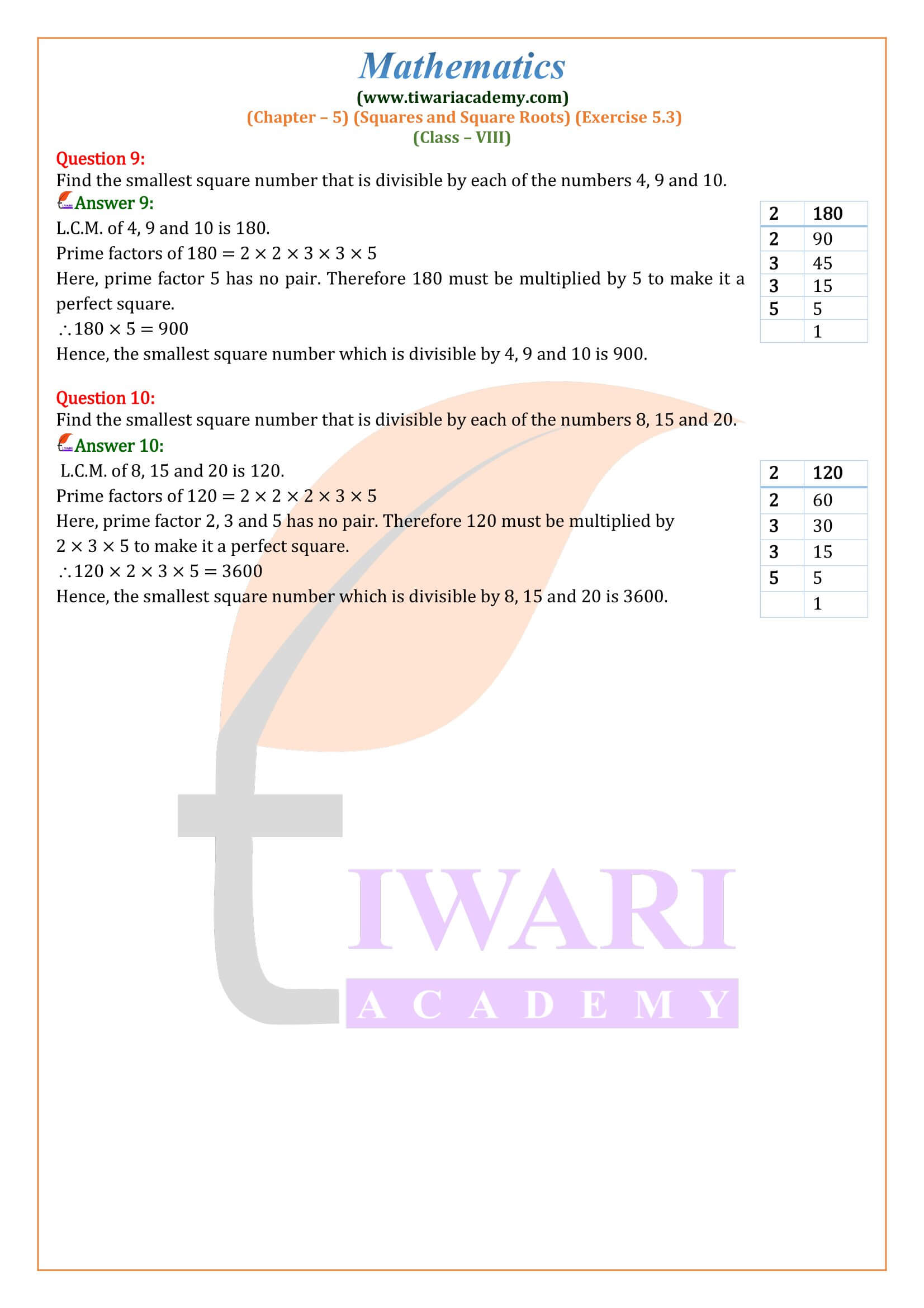
Evaluate (i) 49 x 51 (ii) 30 x 32.
We have:
(i) 49 x 51 = (50 – 1) x (50 + 1)
= [(50)² -1²] = (2500 – 1) = 2499.
(ii) 30 x 32 = (31 – 1) x (31 + 1)
= [(31)² -1²] = (961 – 1) = 960.
Step 1
Make three columns, I, II and III, headed by a, (2 x a x b) and b respectively. Write the values of a², (2 x a x b) and b2 in columns I, II and III respectively.
Step 2
In Column III, underline the unit digit of b and carry over the tens digit of it to Column II and add it to the value of (2 x a x b).
Class 8 Maths Exercise 5.3 Important Questions
What is the square of an even number?
Squares of even numbers are even (and in fact divisible by 4), since (2n)² = 4n².
What is the square of an odd number?
Squares of odd numbers are odd, since (2n + 1)² = 4(n² + n) + 1.
Express 81 as the sum of 9 odd numbers.
The sum of first nine odd numbers are 1 + 3 + 5 + 7 + 9 + 11 + 13 + 15 + 17 = 81 = 9²
Find the square of 47..
Given number = 47. a = 4 and b = 7
| I | II | III |
| a2 | (2 x a x b) | b2 |
| 16 + 6 = 22
|
56 + 4 = 60 | 49 |
So, (47)2 = 2209
Step 3
In Column II, underline the unit digit of the number obtained in Step 2 and carry over the tens digit of it to Column I and add it to the value of a.
Step 4
Underline the number obtained in Step 3 in Column 1. The underlined digits give the required square number.