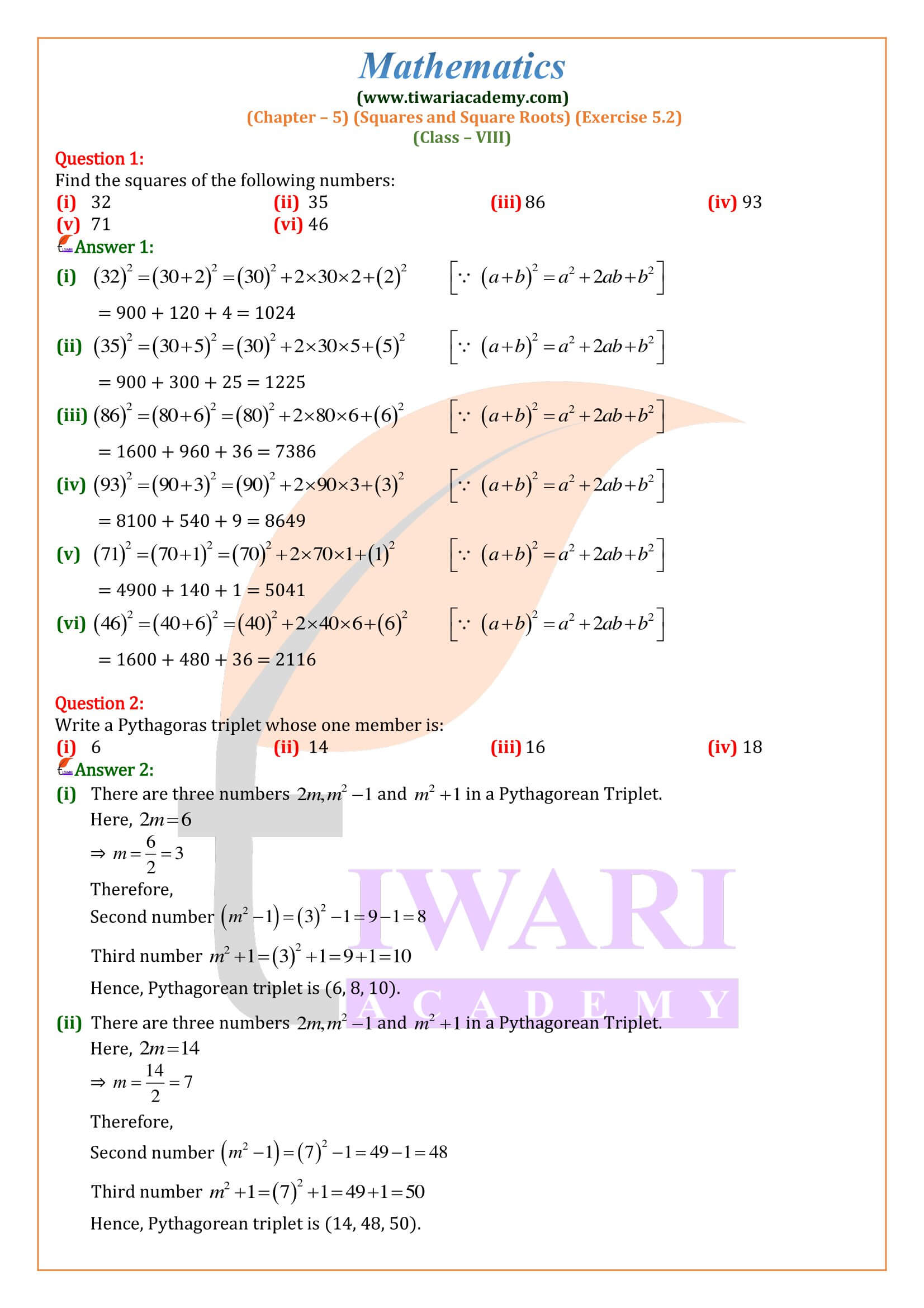
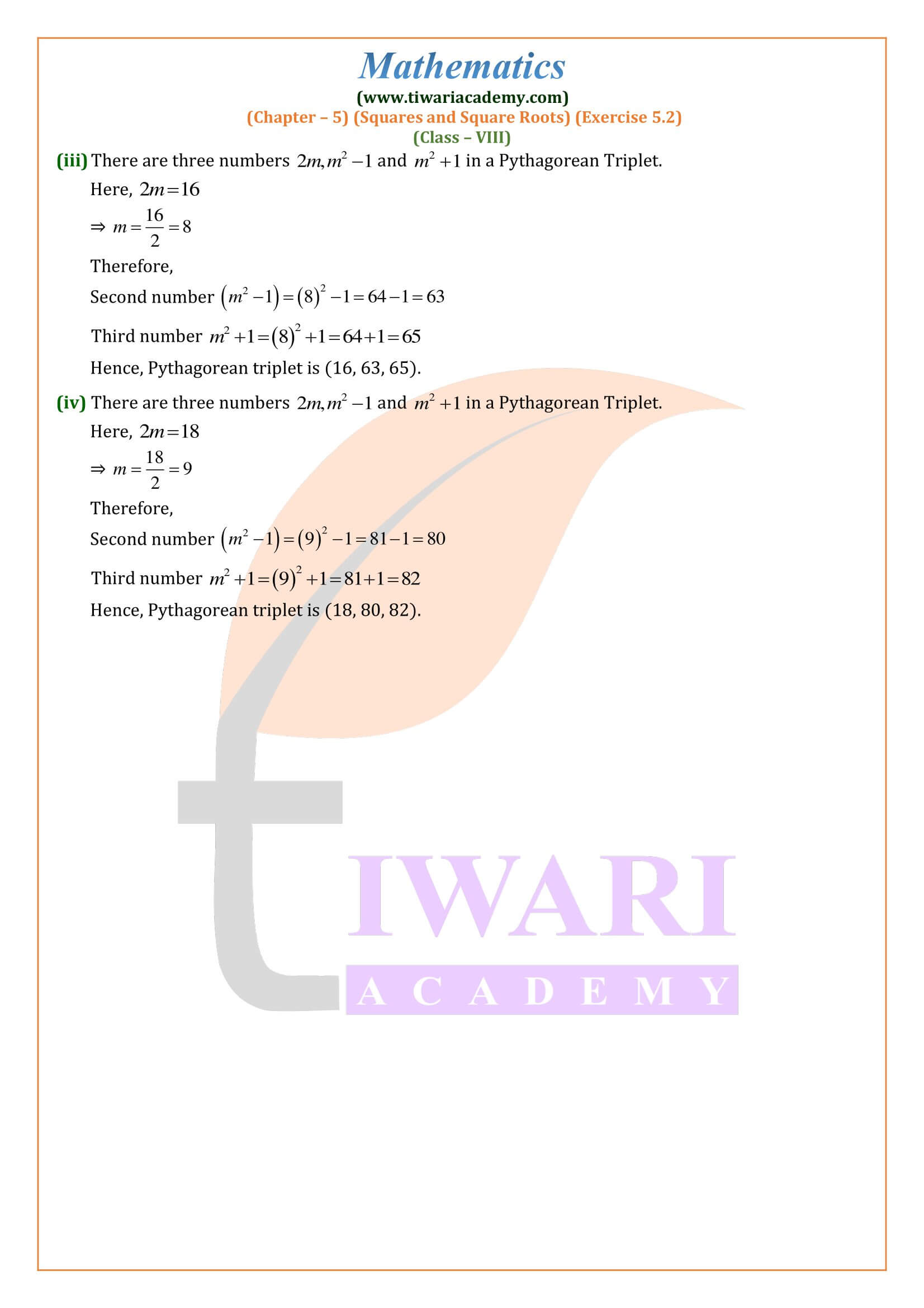
NCERT Solutions for Class 8 Maths Chapter 5 Exercise 5.2 in Hindi and English Medium updated for CBSE 2025-26 exams. Get here the revised question answers and solutions of ex. 5.2 class 8th mathematics based on new NCERT textbooks issued for academic year 2025-26.
8th Maths Exercise 5.2 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 5 | Exercise: 5.2 |
| Chapter Name: | Square and Square Roots |
| Medium: | Hindi and English Medium |
| Session: | Academic Year 2025-26 |
| Content: | Text and Videos Format |
Class 8 Maths Chapter 5 Exercise 5.2 Solution
Class VIII Mathematics Ex. 5.2 Square and Square Roots free to use without any login or registration updated for CBSE session 2025-26. Class 8 NCERT book Maths Exercise 5.2 is helpful for state board students who are using NCERT like books for their course books. Here we will learn how to find the prime factorisation of the given number and then group the prime factors in such a way that in each pair, both factors are the same. In this way we will get the square root of a given number by prime factorisation.
Properties of Perfect Squares
Property 1
A number ending in 2, 3, 7 or 8 is never a perfect square.
Example:
The numbers 82, 93, 187, 248 end in 2, 3, 7, 8 respectively. So, none of them is a perfect square.
Property 2
A number ending in an odd number of zeros is never a perfect square.
Example:
The numbers 160, 4000, 900000 end in one zero, three zeros and five zeros respectively. So, none of them is a perfect square.
Property 3
If a number when divided by 3 leaves a remainder 2, then it is not a perfect square.
Example:
170, 578, 617, 722, etc.
Property 4
If a number when divided by 4 leaves a remainder 2 or 3, then it is not a perfect square.
Example:
578, 654, 798, 1002, etc.
Property 5
The square of an even number is always even.
Example:
2² = 4, 4² = 16, 6² = 36, 8² = 64, etc.
Property 6
The square of an odd number is always odd.
Example:
1² = 1, 3² = 9, 5² = 25, 7² = 49, 9² = 81, etc.
Property 7
The square of a proper fraction is smaller than the fraction.
Example:
(2/3)² = (2/3 x 2/3) = 4/9 and 4/9 < 2/3, since (4 x 3) < (9 x 2)
Property 8
For every natural number n, we have:
(n + 1)² –n² = (n + 1 + n) (n + 1 – n) = {(n + 1) + n)}.
{(n + 1)² –n²} = {(n + 1) + n)}.
Example:
(i) {(36)² – (35)²} = (36 + 35) = 71
Property 9
For every natural number n, we have:
sum of first n odd natural numbers = n².
Example:
(i) (1 + 3 + 5 + 7 + 9) = sum of first 5 odd numbers = 5².
(ii) (1 + 3 + 5 + 7 + 9 + 11 + 13 + 15) = sum of first 8 odd numbers = 8².
Property 10
Between two consecutive square numbers n and (n + 1), there are 2n non-perfect square numbers.
Example:
Square numbers are 1², 2², 3², 4², ….., etc.
- Between 1² and 2², there are 2 x 1 = 2 non-perfect square numbers, viz., 2², 3².
- Between 4² and 5², there are 4 x 2 = 8 non-perfect square number, viz., 17, 18, 19, 20, 21, 22, 23, 24.
Property 11
The square of an even number is always an even number and the square of an odd number is always an odd number.
Property 12
- (i) The square of a natural number (except 1) is either a multiple of 3 or exceeds a multiple of 3 by 1.
- (ii) The square of a natural number (except 1) is either a multiple of 4 or exceeds a multiple of 4 by 1.
Property 13
The square of a natural number ending with 5 follows a definite pattern.
(25)² = (2 x 3) hundred + 25 = 625
(35)² = (3 x 4) hundred + 25 = 1225
(45)² = (4 x 5) hundred + 25 = 2025
Property 14
The sum of first n odd natural numbers is n².
Examples:
First odd number = 1 = 1²
Sum of first two odd numbers = 1 + 3 = 4 = 2²
Sum of first three odd numbers = 1 + 3 + 5 = 9 = 3²
Sum of first four odd numbers = 1 + 3 + 5 + 7 = 16 = 4²
In other words, if a number is a prefect square, then it is always equal to the sum of consecutive odd numbers starting from 1.
Property 15
Some square numbers follow interesting patterns. Observe the following squares:
Example:
- (1)² = 1
- (11)² = 121
- (111)² = 12321
- (1111)² = 1234321
- (11111)² = 123454321
Class 8 Maths Exercise 5.2 Important Questions
What are the properties of a perfect square?
(i) A Square number can only end with digits 0, 1, 4, 5, 6, 9.
(ii) Number of zeroes at the end of a perfect square is always even.
(iii) Square of even numbers are always even,
(iv) Square of odd numbers are always odd.
(v) If a number has 1 or 9 in its unit place,
(vi) its square ends with 1.
(vii) If a number has 4 or 6 in its unit place,
How do you solve a perfect square question?
Steps to Solving Equations by Completing the Square:
Rewrite the equation in the form x² + bx = c.
Add to both sides the term needed to complete the square.
Factor the perfect square trinomial.
Solve the resulting equation by using the square root property.
Perfect Square Trinomial Formula
An expression is said to a perfect square trinomial if it takes the form ax² + bx + c and satisfies the condition b² = 4ac. The perfect square formula takes the following forms: (ax)² + 2abx + b² = (ax + b)²
If we look at the results of these squares, we find that, they have a special property i.e., the sum of the digits of every number on the right hand side is a perfect square.
121 = 1 + 2 + 1 = 4 = 2²
12321= 1 + 2 + 3 + 2 + 1 = 9 = 3²
1234321 = 1 + 2 + 3 + 4 + 3 + 2 + 1 = 16 = 4²
123454321 = 1 + 2 + 3 + 4 + 5 + 4 + 3 + 2 + 1 = 25 = 5²
And so on…
(7)² = 49
(67)² = 4489
(667)² = 444889
(6667)² = 44448889
If we look at the results of these squares, we find that they follow another interesting property. Using this pattern, we can find the square of the numbers 66667, 666667, ….