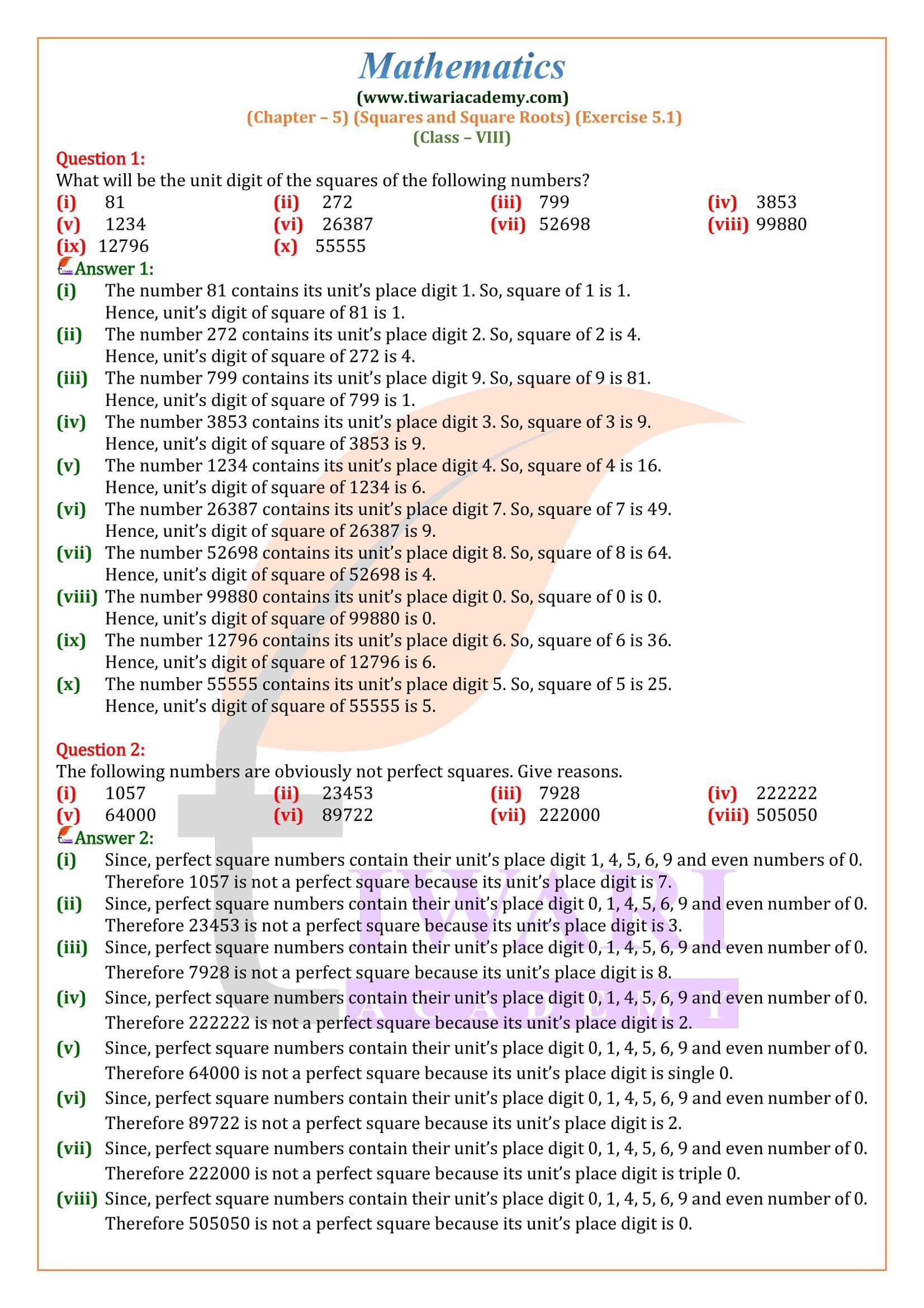
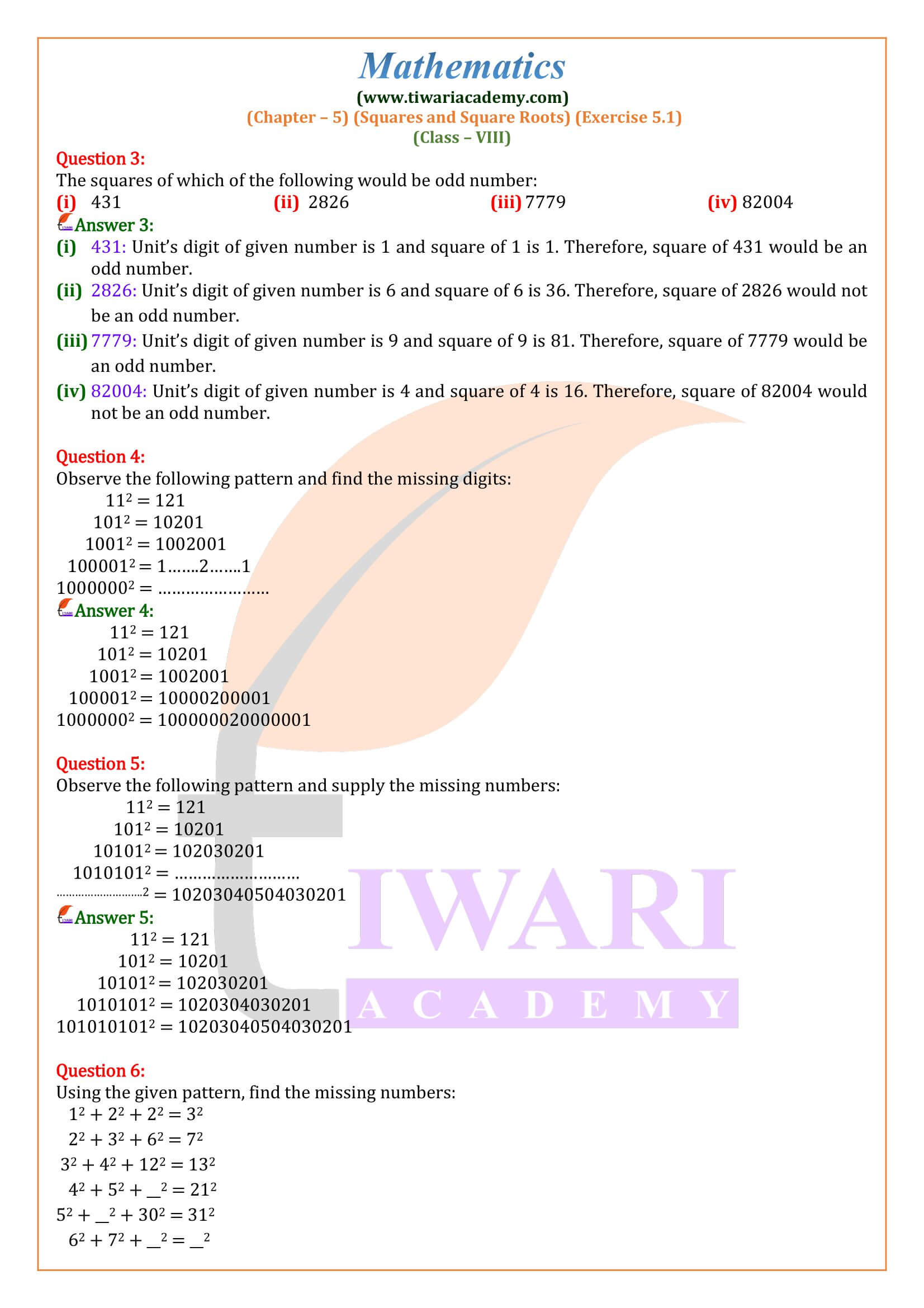
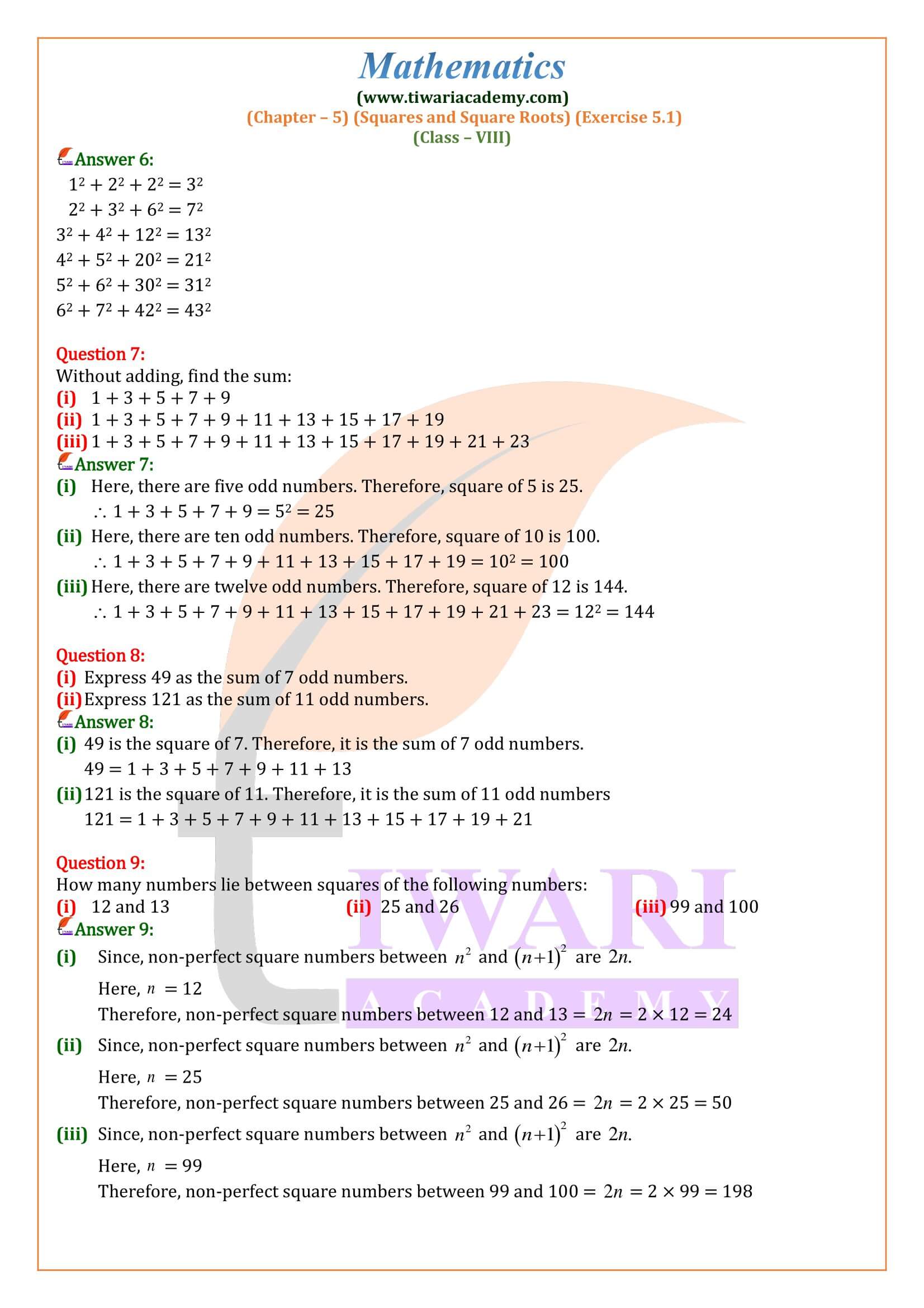
NCERT Solutions for Class 8 Maths Chapter 5 Exercise 5.1 Squares and square roots in Hindi and English Medium updated for CBSE 2025-26 Exams. All the questions of ex. 5.1 are revised according to new syllabus and based on new NCERT books issued for new session 2025-26.
8th Maths Exercise 5.1 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 5 | Exercise: 5.1 |
| Topic Name: | Square and Square Roots |
| Medium: | Hindi and English Medium |
| Session: | CBSE 2025-26 |
| Content: | NCERT Exercise Solution |
Class VIII Mathematics NCERT textbook Ex. 5.1 Square and Square Roots for academic session 2025-26 in Hindi and English Medium free to download. Here, we will learn to find square and square roots of numbers using short cut method, factorisation, and long division method, square roots in decimal form and square roots of fractions. NCERT book Exercise 5.1 videos solutions also contain the complete explanation of each question of ex. 5.1 class 8 Maths.
Square of A Number
The square of a number is the product of the number with the number itself.
examples:
- The square of 2 is 2² = 2 x 2 = 4
- The square of 3 is 3² = 3 x 3 = 9
- The square of 4 is 4² = 4 x 4 = 16
- The square of 5 is 5² = 5 x 5 = 25.
Class 8 Maths Chapter 5 Exercise 5.1 Solution
Perfect Squares
When the exponent (power) of a natural number is 2, the number so obtained is called a square number or a perfect square. Thus, when a number is multiplied by itself, the product is a perfect square.
For example: 1² = 1, 2² = 4, 3² = 9, 4² = 16, 5² = 25.
Therefore, 1, 4, 9, 16, 25 are perfect squares.
To Find, whether a Number is a Perfect Square or Not:
- Write the prime factorisation of the given number.
- Group the prime factors in such a way that in each pair, both factors are the same.
- If no factor is left over after grouping, the number is a perfect square.
- If any number or numbers are left whose grouping is not possible, then the number is not a perfect square.
8th Maths Exercise 5.1 Examples
Class 8 Maths Exercise 5.1 Important Questions
Show that 1764 is a perfect square. Find the number whose square is 1764.
Resolving 1764 into prime factors, we get,
1764 = 2 x 2 x 3 x 3 x 7 x 7
Thus, 1764 is the product of pairs of equal factors.
1764 is a perfect square.
Also, 1764 = (2² x 3² x 7²) = (2 x 3 x 7)² = (42)²
Hence, 42 is the number whose square is 1764.
Show that 900 is a perfect square. Find the number whose square is 900.
Resolving 900 into prime factors, we get,
900 = 2 x 2 x 3 x 3 x 5 x 5
Thus, 900 is the product of pairs of equal factors.
900 is a perfect square.
Also, 900 = (2² x 3² x 5²) = (2 x 3 x 5)² = (30)²
Hence, 30 is the number whose square is 900.
Show that 35721 is a perfect square. Find the number whose square is 35721.
Resolving 35721 into prime factors, we get,
35721 = 3 x 3 x 7 x 7 x 9 x 9
Thus, 35721 is the product of pairs of equal factors.
35721 is a perfect square.
Also, 35721 = (3² x 7² x 9²) = (3 x 7 x 9)² = (189)²
Hence, 189 is the number whose square is 35721.
Is 196 a perfect square? If so, find the number whose square is 196.
Resolving 196 into prime factors, we get
196 = 2 x 2 x 7 x 7
Or, 142 = 2² x 7²
= (2 x 7)² = (14)²
Thus, 196 can be expressed as a product of pairs of equal factors.
So, 196 is a perfect square.
Hence, 14 is the number whose square is 196.