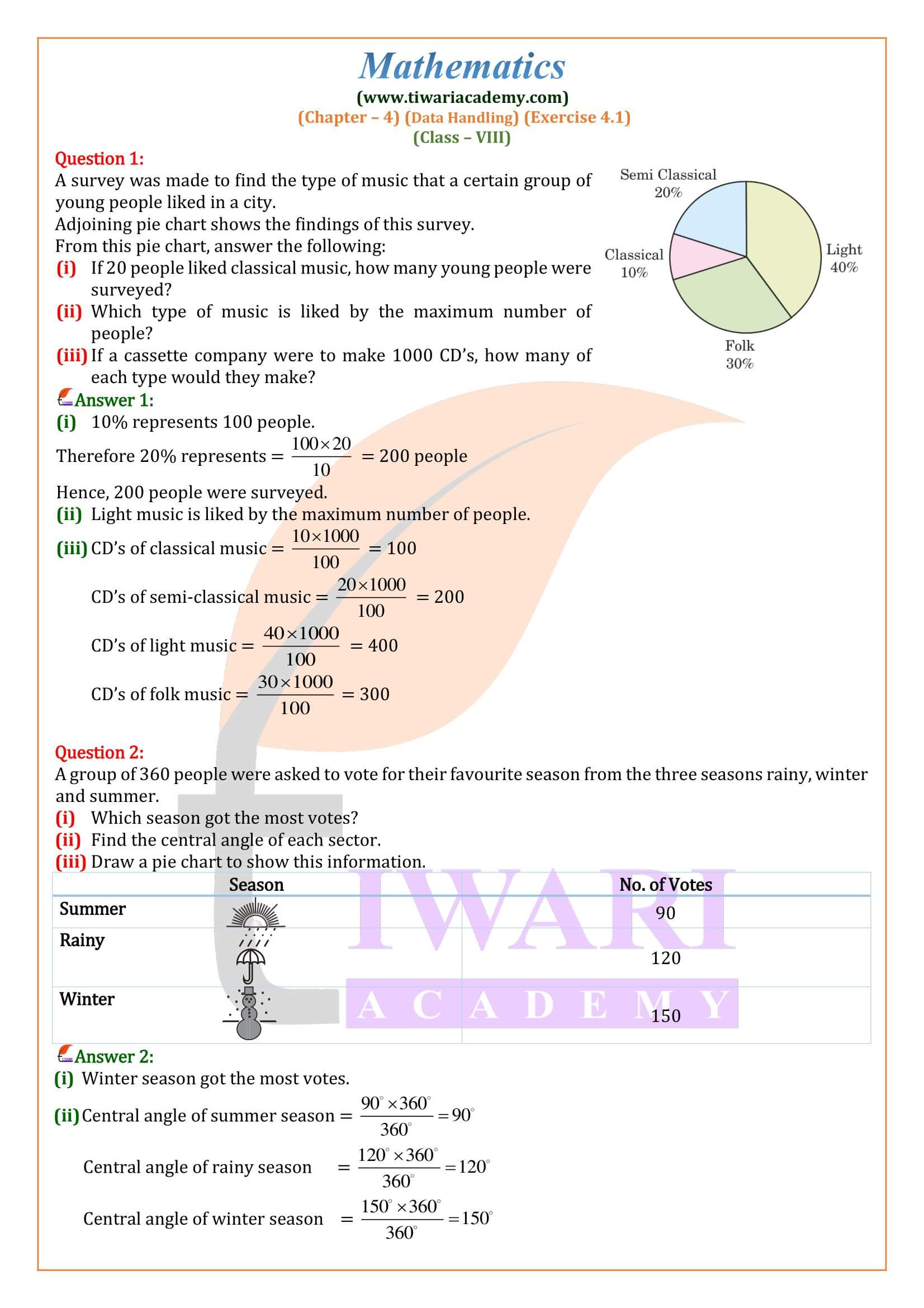
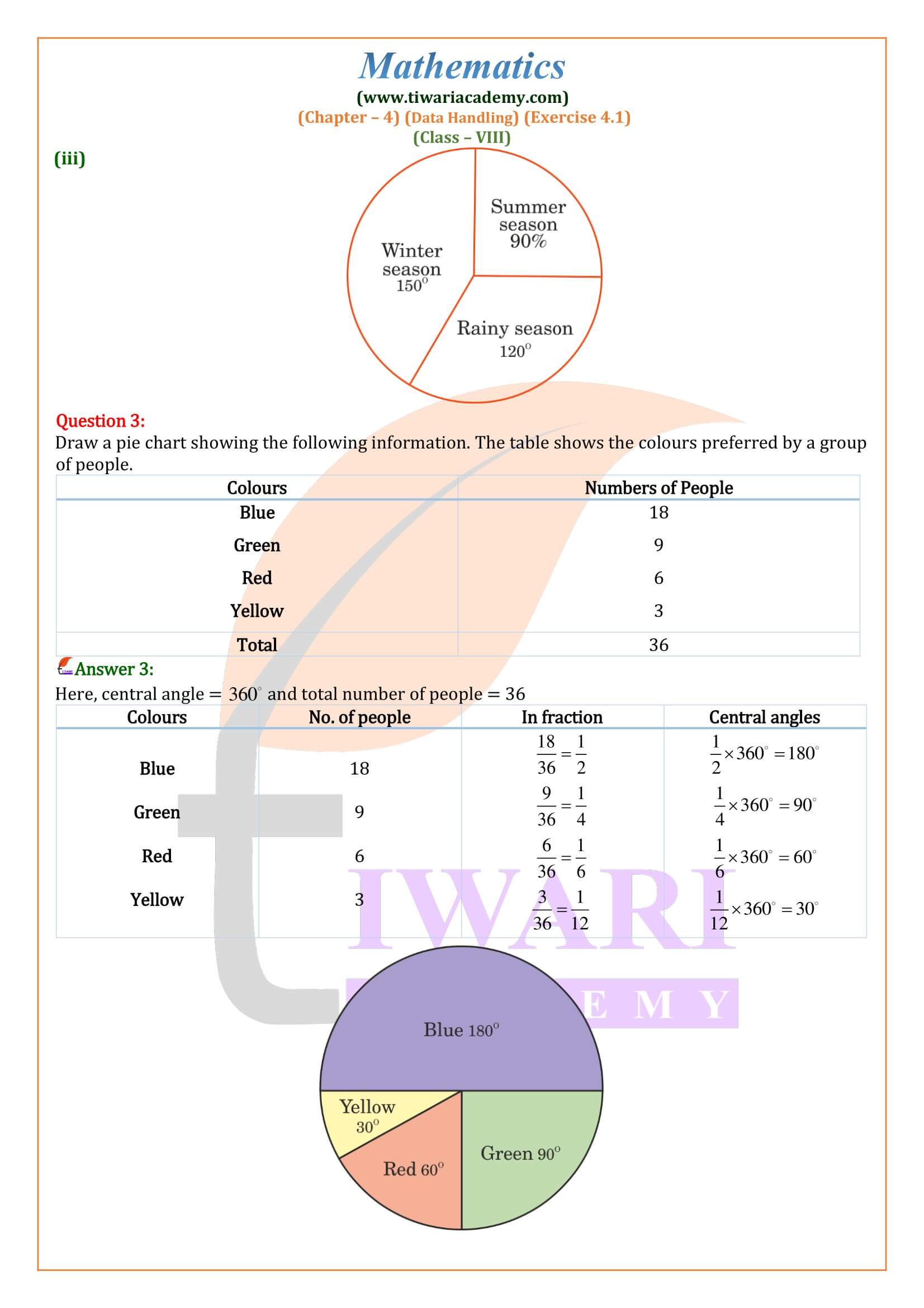
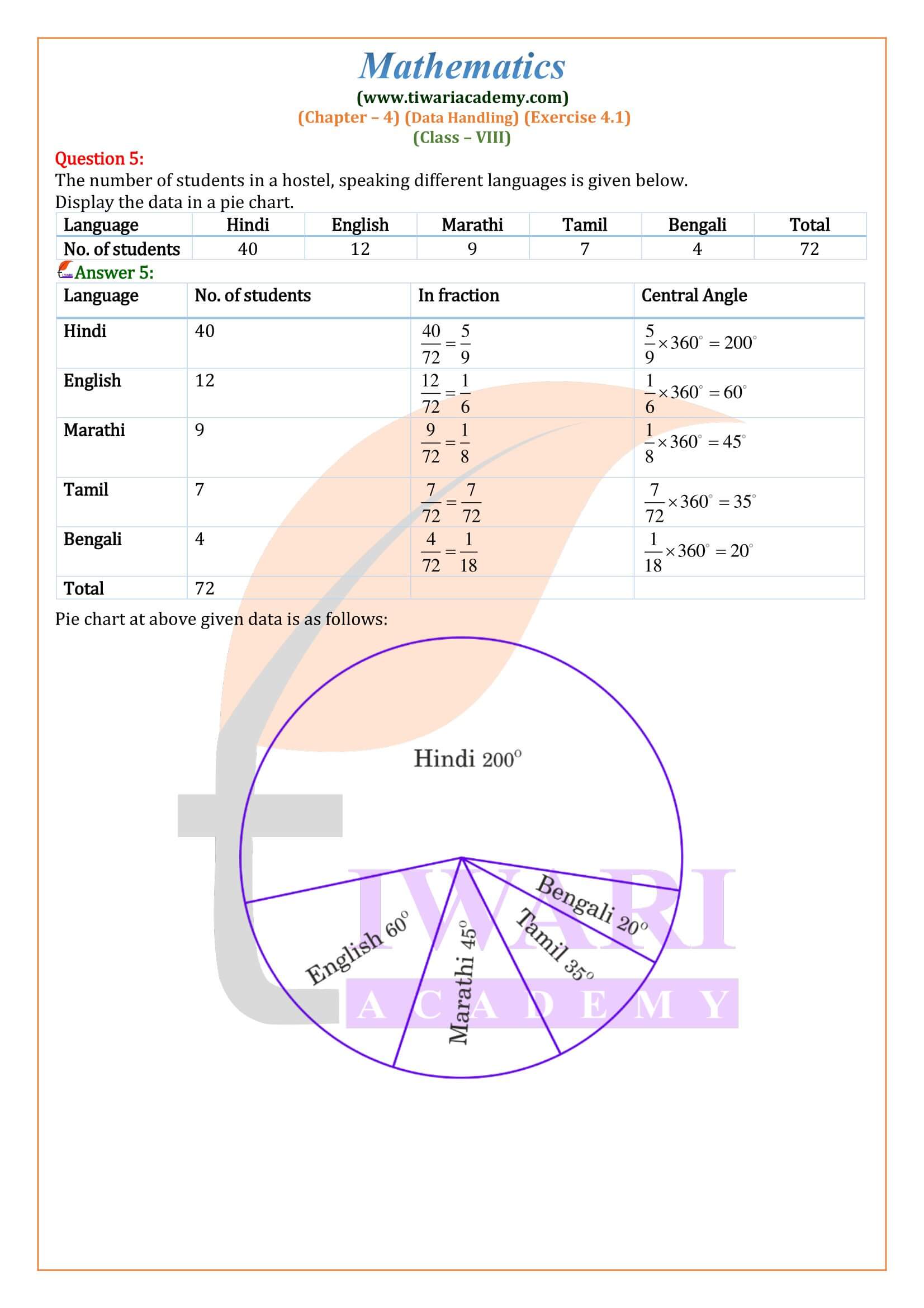
NCERT Solutions for Class 8 Maths Chapter 4 Exercise 4.1 in Hindi and English medium prepared for CBSE 2025-26 session. Get here updated solutions of ex. 4.1 class 8th mathematics revised according to new NCERT textbooks issued for 2025-26 Examination.
8th Maths Exercise 4.1 Solution in Hindi and English Medium
Class 8 Maths Chapter 4 Exercise 4.1 Solution
Class VIII Mathematics NCERT Ex. 4.1 Data Handling free to download or use online updated for academic session 2025-26. All the questions of ex. 4.1 class 8 mathematics is solved using proper steps in easier way. Explanation of questions are also given in videos format, so that students can understand question answers in better way. Solutions are given in Hindi and English Medium free to use online.

| Class: 8 | Mathematics |
| Chapter: 4 | Exercise: 4.1 |
| Topic Name: | Data Handling |
| Content: | NCERT Book Solutions |
| Session: | CBSE 2025-26 |
| Medium: | Hindi and English Mediun |
Frequency Distribution
Frequency of an Observation
The number of times a particular observation occurs in a given data is called its frequency.
Suppose we make a survey of 20 families of a locality and find out the number of children in each family. Let the observations be 2, 2, 3, 1, 1, 2, 3, 2, 2, 1, 2, 2, 3, 1, 2, 1, 1, 3, 2, 2.
State the frequency of each observation.
Arranging the data in ascending order, we get the observations as
1, 1, 1, 1, 1, 1, 2, 2, 2, 2, 2, 2, 2, 2, 2, 2, 3, 3, 3, 3.
We find that 1 occurs 6 times;
2 occurs 10 times;
and 3 occurs 4 times.
We say that the frequency of families having 1 child is 6,
The frequency of families having 2 children is 10,
And the frequency of families having 3 children is 4.
Thus, the frequency distribution table of the above data may be presented as given below:
| Number of children | Number of families (frequency) |
|---|---|
| 1 | 6 |
| 2 | 10 |
| 3 | 4 |
| Total | 20 |
Grouping of Data
So far, we have considered ungrouped data. When the number of observations is large, we may condense the data into several groups. We record the frequency of observation falling in each group.
Presentation of data in groups along with the frequency of each group is known as the frequency distribution of the grouped data.
The marks obtained by 40 students of class VIII in an examination are given below: 16, 17, 18, 3, 7, 23, 18, 13, 10, 21, 7, 1, 13, 21, 13, 15, 19, 24, 16, 2, 23, 5, 12. 18, 8, 12, 6, 8, 16, 5, 3, 5, 0, 7, 9, 12, 20, 10, 2, 23. Divide the data into five groups, namely 0- 5, 5-10, 10-15, 15-20 and 20-25, where 0-5 means marks greater than or equal to O but less than 5, and similarly 5-10 means marks greater than or equal to 5 but less than10, and so on. Prepare a frequency table for the grouped data.
Arranging the given observations in ascending order, we get them as 0, 1, 2, 2, 3, 3, 5, 5, 5, 6, 7, 7, 7, 8, 8, 9, 10, 10, 12, 12, 12, 13, 13, 13, 15, 16, 16, 16, 17, 18, 18, 18, 19, 20, 21, 21, 23, 23, 23, 24, Thus, the frequency distribution may be given as under:
| Marks (class of Interval) | Frequency (Number of students) |
|---|---|
| 0-5 | 6 |
| 5-10 | 10 |
| 10-15 | 8 |
| 15-20 | 9 |
| 20-25 | 7 |
| Total | 40 |
Here, each of the groups 0-5, 5-10, 10-15, 15-20 and 20-25 is called a class interval.
Class 8 Maths Exercise 4.1 Important Questions
What is frequency distribution in data handling?
A frequency distribution is a representation, either in a graphical or tabular format, that displays the number of observations within a given interval. The interval size depends on the data being analyzed and the goals of the analyst. Frequency distributions are typically used within a statistical context.
How do you construct a frequency distribution?
Steps to Making Your Frequency Distribution:
Step 1: Calculate the range of the data set.
Step 2: Divide the range by the number of groups you want and then round up.
Step 3: Use the class width to create your groups.
Step 4: Find the frequency for each group.
How do you find the grouped frequency distribution?
Creating a Grouped Frequency Distribution
Find the largest and smallest values.
Compute the Range = Maximum – Minimum.
Select the number of classes desired.
Find the class width by dividing the range by the number of classes and rounding up.
Pick a suitable starting point less than or equal to the minimum value.
In class interval 10-15, the number 10 is called the lower limit and 15 is called the upper limit of the class interval.
The difference between the upper limit and the lower limit of any class interval is called the class size. Thus, the class size in the above frequency distribution is 5.
The mid value of a class is called its class mark and is obtained by adding its upper and lower class limits and dividing the sum by 2.
Thus, the class mark of 0-5 is (0 + 5)/2 = 2.5
the class mark of 5-10 is (5 + 10)/2 = 7.5