by Tiwari Academy
Class 8 Maths Exercise 3.4 Solutions
Class 8 Maths Exercise 3.4 in Hindi
Class 8 Maths Book Download in PDF
Class 8 Maths Chapter 3 Solutions
Class 8 Maths NCERT Solutions
Class 8 All Subjects Solutions
NCERT Solutions for Class 8 Maths Chapter 3 Exercise 3.4 in Hindi and English medium modified and updated for academic year 2024-25. Students of class 8 can get the revised solutions of ex. 3.4 here which is based on the new revised NCERT textbooks issued for 2024-25 exam pattern.
8th Maths Exercise 3.4 Solution in Hindi and English Medium
Class VIII Mathematics textbook Ex. 3.4 of Understanding Quadrilaterals free to download in PDF updated for CBSE exams 2024-25. Learn here all the properties of quadrilateral, special type of quadrilateral and practice questions based on properties of quadrilaterals. The NCERT Books questions are solved in text file as well as videos format to help the students. Solution and explanation videos are given separately. No login or registration is required for the access of contents. We never disturb the user by call or message.
| Class: 8 | Mathematics |
| Chapter: 3 | Exercise: 3.4 |
| Topic Name: | Understanding Quadrilaterals |
| Medium: | Hindi and English |
| Content Type: | Images, Online Text and Videos |
| Academic Session: | 2024-25 |
Download App for Class 8
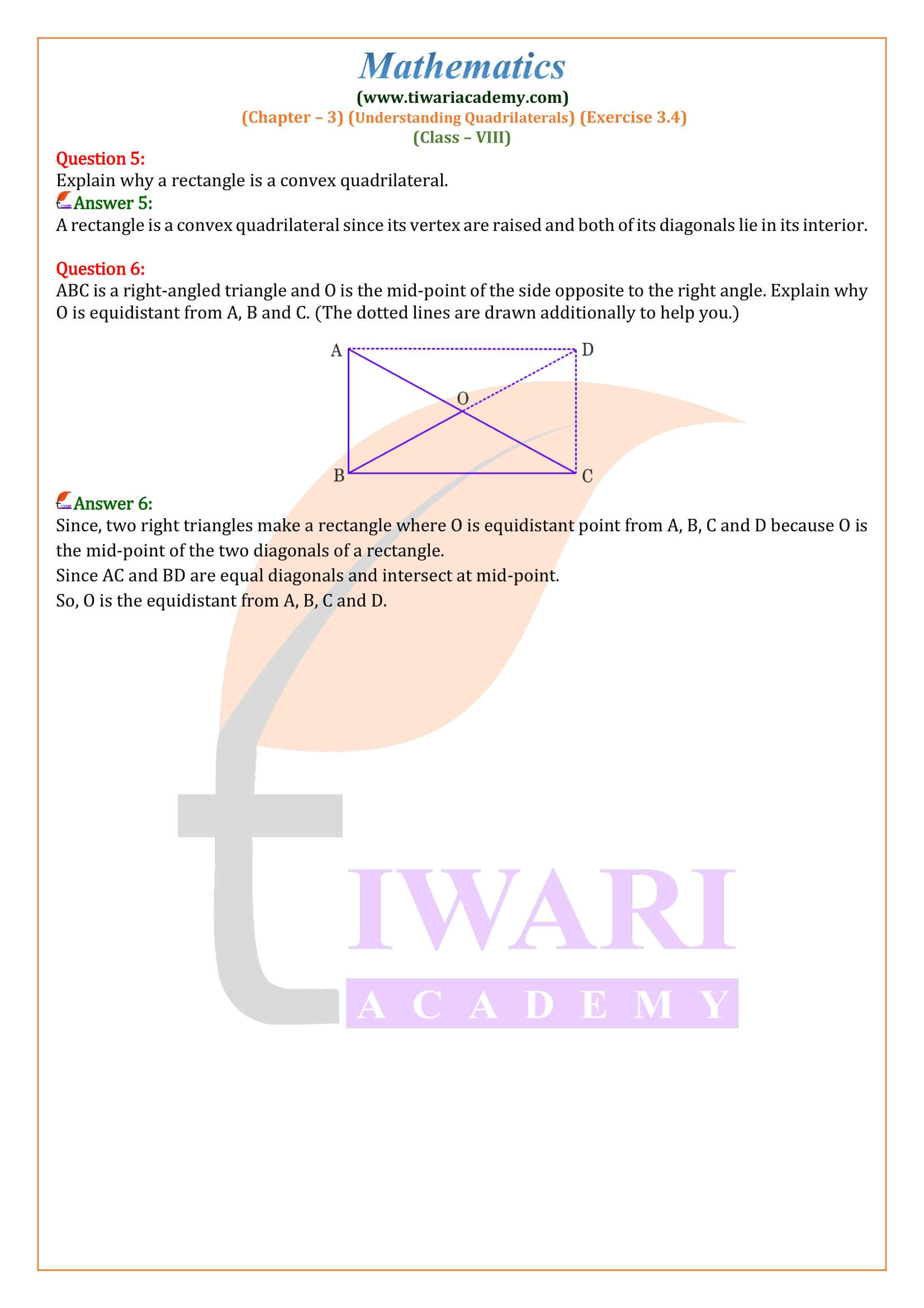
Diagonal Properties of Rectangle
Result 2
Prove that the diagonals of a rectangle are equal and bisect each other.
PROOF
Let ABCD be a rectangle whose diagonals AC and BD intersect at the point O. From DABC and DBAD, we have
AB = BA (common)
∠ABC = ∠BAD (each equal to 90°)
BC = AD (opposite sides of a rectangle
So, triangle ABC congruent triangle BAD (by SAS congruence)
Or, AC = BD.
Hence, the diagonals of a rectangle are equal.
From triangle OAB and triangle OCD.
we have ∠OAB = ∠OCD (alternate angles)
∠OBA = ∠ODC (alternate angles)
AB = CD (opposite sides of a rectangle)
So, triangle OAB congruent triangle OCD (by ASA congruence)
Or, OA = OC and OB =OD.
This shows that the diagonals of a rectangle bisect each other.
Hence. the diagonals of a rectangle are equal and bisect each other.
Diagonal Properties of a Rhombus
Result 3
Prove that the diagonals of a rhombus bisect each other at right angles.
PROOF
Let ABCD be a rhombus whose diagonals AC and BD intersect at the point O.
We know that the diagonals of a parallelogram bisect each other.
Also, we know that every rhombus is a parallelogram.
So, the diagonals of a rhombus bisect each other.
OA = OC and OB = OD
From DCOB and DCOD,
we have: CB = CD (sides of a rhombus)
CO = CO (common)
OB = OD (proved)
So, triangle COB congruent triangle COD (by SSS congruence)
Or, ∠COB = ∠COD. (Rule)
But, ∠COB + ∠COD = 2 right angles (linear pair)
So, ∠COB = ∠COD = 1 right angle.
Hence, the diagonals of a rhombus bisect each other at right angles.
Diagonal Properties of a Square
Result 3
Prove that the diagonals of a square are equal and bisect each other at right angles.
Proof
We know that the diagonals of a rectangle are equal.
Also, we know that every square is a rectangle.
So the diagonals of a square are equal.
Again we know that the diagonals of a rhombus bisect each other at right angles.
But every square is a rhombus.
So, the diagonals of a square bisect each other at right angles.
Hence, the diagonals of a square are equal and bisect each other at right angles.
Area of Parallelogram
Area of a parallelogram = base x height = b x h
Area of Rhombus
Rhombus is a special type of parallelogram in which all four sides are equal.
Area of a rhombus = (Product of diagonals)/2
Area of a Triangle
Area of a triangle = (base x height)/2
Class 8 Maths Exercise 3.4 Extra Questions for Practice
The area of a square PQRS is 36 sq. cm. Find the area of the square joining the mid-points of the sides of PQRS.
Area of square PQRS = 36 sq. cm
So, Each side of square PQRS = 36 cm = 6 cm
AQ = (PQ) = x 6 cm = 3 cm (ABCD being a square and A is the mid-point of PQ)
we have Similarly, QB = 3 cm
In right angled DAQB, by Pythagoras theorem
AB2 = AQ2 + QB2
AB2 = (3)2 + (3)2 = 9 + 9 = 18
So, AB = √18 cm = 3 √2 cm
Thus, area of the square ABCD = (side x side)
= 3 √2 x 3 √2 sq. cm = 18 sq. cm
The length of a rectangle is 8 cm and each of its diagonals measures 10 cm. Find its breadth.
Let ABCD be the given rectangle in which length AB = 8 cm and
diagonal AC = 10 cm.
Since each angle of a rectangle is a right angle, we have
∠ABC = 90°.
From the right DABC, we have
AB2 + BC2 = AC2
Then, BC2 = (AC2 – AB2) = {(10)2 – (8)2} cm = (100 – 64) cm = 36 cm
Or, BC = 36 cm = 6 cm.
Hence, breadth = 6 cm.
The area of the parallelogram ABCD is 360 sq. cm. If AB = 30 cm and DE = 5 cm, from the given figure, find the side BC of the parallelogram.
Area of the parallelogram ABCD = base x height
360 = DC x AE
Or, 360 = 30 x AE (QDC = AB = 30 cm)
Or, AE = 12 cm
In right angled DAED, by Pythagoras theorem
AD2 = AE2 + DE2
Or, AD2 = 122 + 52
So, AD2 = 144 + 25
So, AD2 = 169
So, AD = √169 cm = 13 cm or BC = 13 cm (QAD = BC) Hence, side BC of the parallelogram ABCD is 13 cm.
In a right angled triangle, the ratio between the base and the altitude (height) is 5 :3, If the area of the triangle is 1080 sq. m, find the measure of the base and the altitude.
Let the base be 5x then altitude is 3x.
Area of the triangle = (base x altitude)
1080 = (5x x 3x)/2
1080 = (15×2)/2
So, X2 = (1080 x 2)/15 = 144
Or, X = 12
So, Base = 5x = (5 x 12) m = 60 m
And, Altitude = 3x = (3 x 12) m = 36 m
The base and altitude of the right angled triangle are 60 m and 36 m respectively
Summary About Diagonals
- Diagonals of a rectangle are equal and bisect each other.
- Diagonals of a rhombus bisect each other at right angles.
- Diagonals of a square are equal and bisect each other at right angles.
Perimeter and Area of Polygons
| Shapes | Perimeter (in units) | Area (in sq. units) |
|---|---|---|
| Rectangle | 2 (l + b) | l x b |
| Square | 4a | a x a = a2 |
| Parallelogram | 2 (a + b) | b x h |
| Rhombus | 4a | 2 (d1 x d2) |
