NCERT Solutions for Class 8 Maths Chapter 13 Exercise 13.2 Introduction to Graphs in Hindi and English Medium updated for CBSE session 2025-26. Get here the revised solutions of ex. 13.2 class 8th mathematics based on new syllabus and latest NCERT books for CBSE 2025-26.
8th Maths Exercise 13.2 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 13 | Exercise: 13.2 |
| Topic: | Introduction to Graphs |
| Content: | NCERT Exercise Solution |
| Content Type: | Text and Videos Format |
| Session: | CBSE 2025-26 |
| Medium: | Hindi and English Medium |
Class 8 Maths Chapter 13 Exercise 13.2 Solution
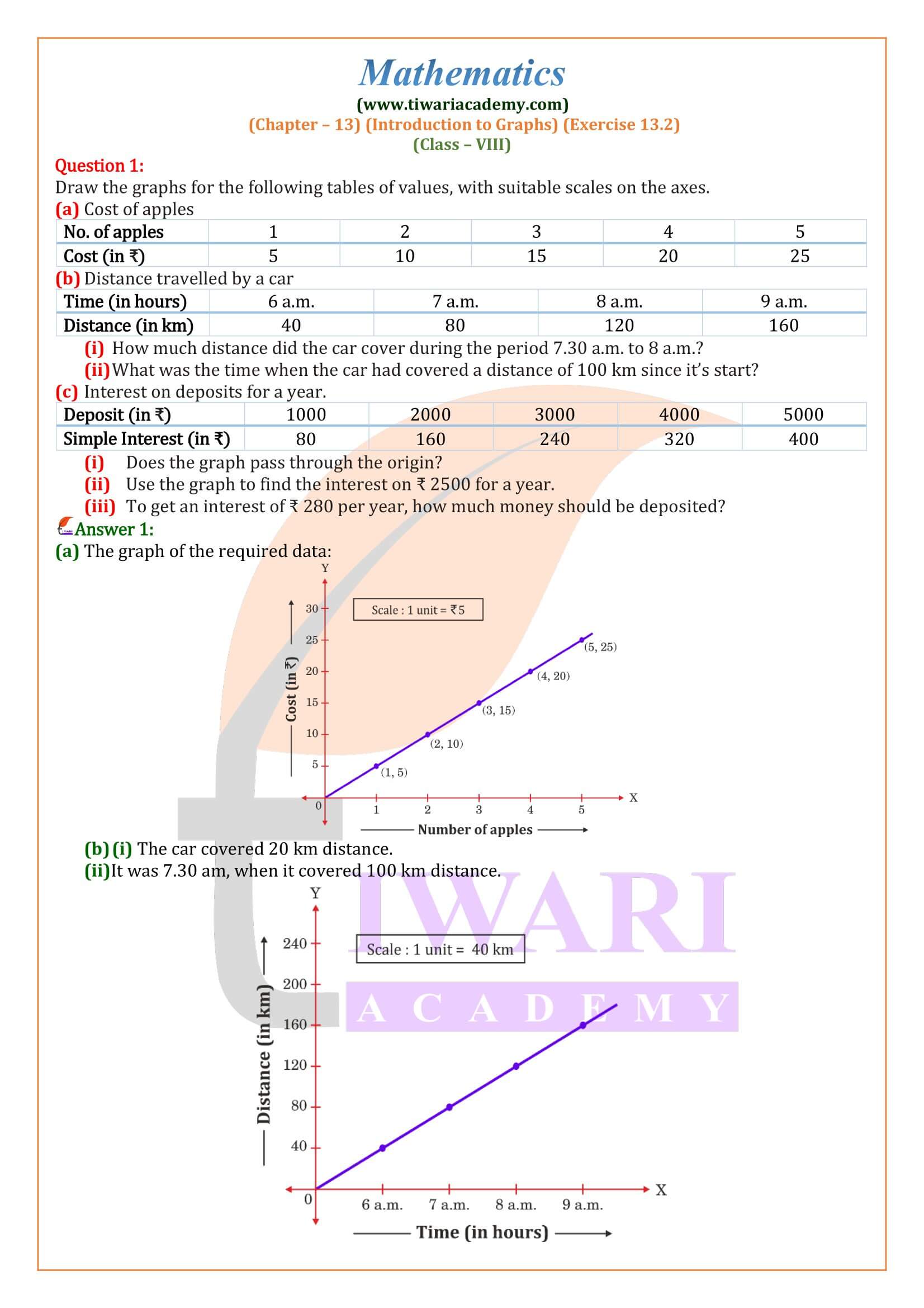
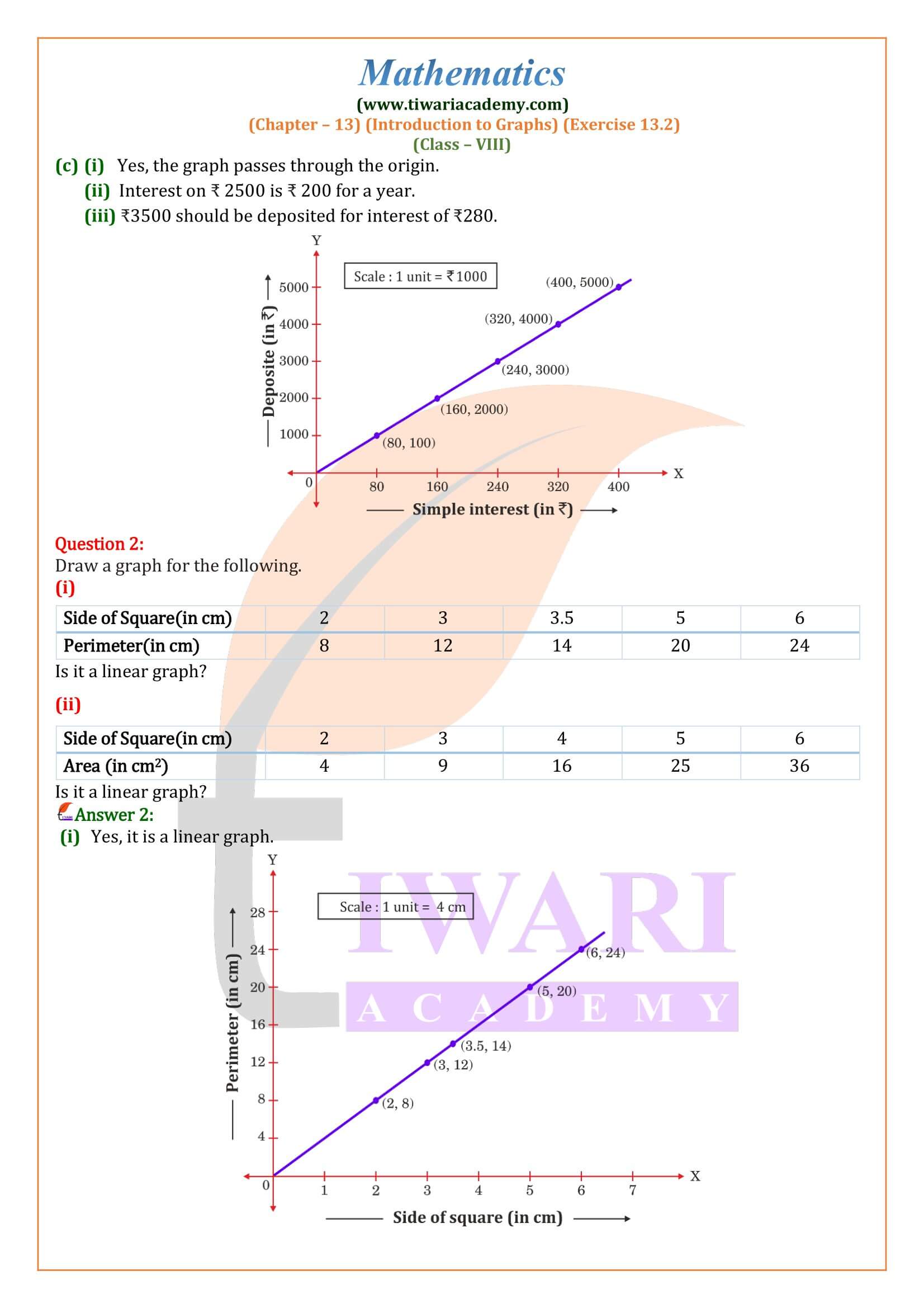
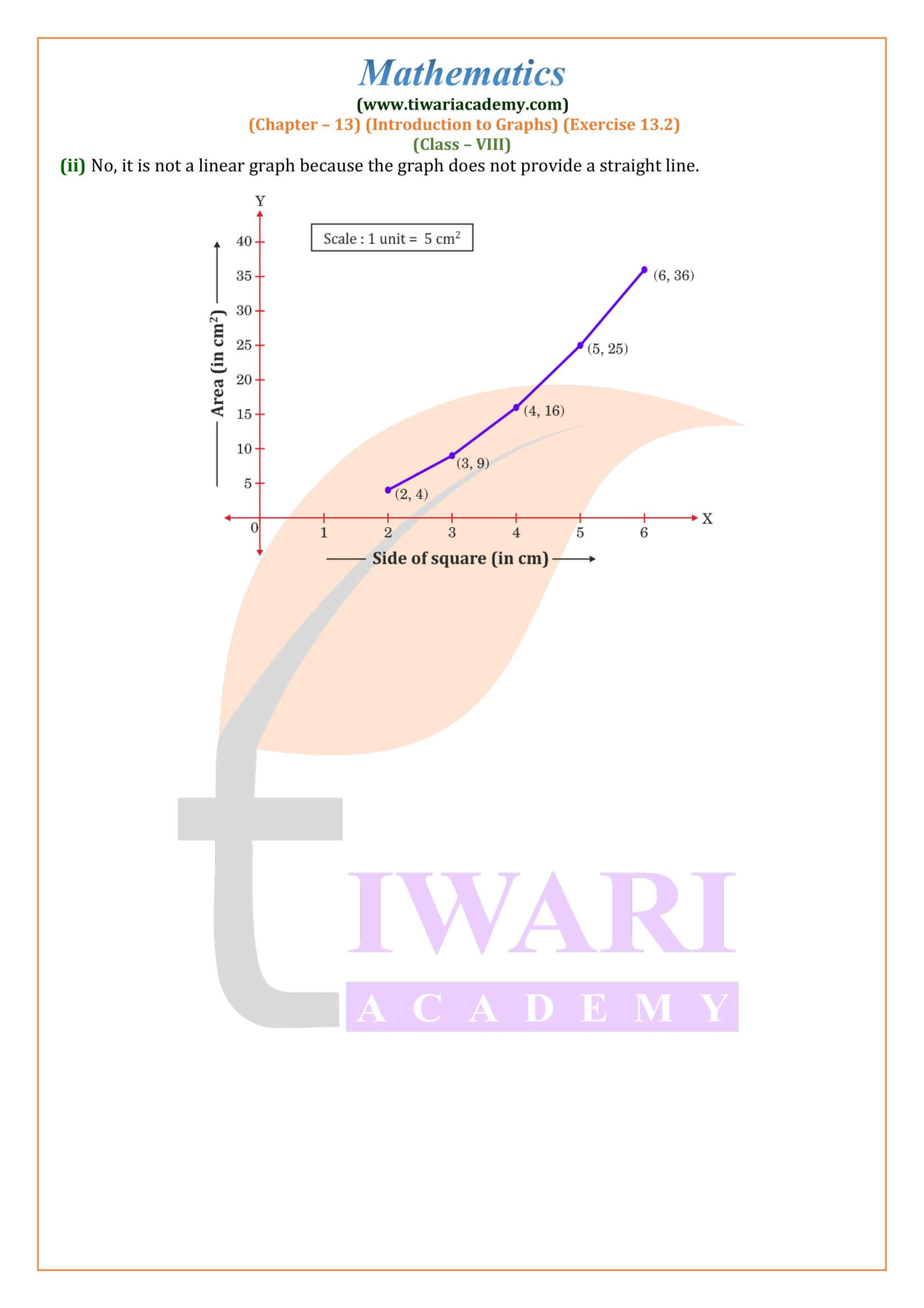
Class VIII Mathematics NCERT Book chapter 13 Ex. 13.2 Introduction to Graphs updated for academic session 2025-26 free to download. In this exercise we will learn how to draw a line using given set of points. CBSE Class 8 Maths exercise 13.2 is based on linear equation in x-y plane. The plotting of graph is described in video and PDF properly step by step. These are explained in such a way that a student can understand easily.
Probability
in everyday life, we come across statements such as:
- Most probably it will rain today.
- Chances are high that the prices of petrol will go up.
- I doubt that he will the race.
The words ‘most probably’, ‘chances’, ‘doubt’, etc., show the probability of occurrence of an event.
Experiment: An operation which can produce some well-defined outcomes is called an experiment. Each outcome is called an event.
Random Experiment
An experiment in which all possible outcomes are known and the exact outcome cannot be predicted in advance, is called a random experiment. Thus, when we throw a coin we know that all possible outcomes are head and tail. But, if we throw a coin at random, we cannot predict in advance whether its upper face will show a head or a tail. Performing a random experiment is often referred as a trial.
Some Experiments and Their Outcomes
Tossing A Coin
Suppose we toss a coin and let it fall on the ground. Its upper face will show either Head (H) or Tail (T). Whatever comes up, is called an outcome.
All possible outcomes are Head (H) and Tail (T).
Tossing two coins
Suppose we toss two coins simultaneously. Then, all possible outcomes are HH, HT, TH and TT.
(Here HH means Head on 1st coin and Head on 2nd coin, HT means Head on 1st coin and Tail on 2nd coin, etc.)
Throwing a die
A die is a solid cube having 6 faces, marked as 1, 2, 3, 4, 5, 6 respectively.
plural of die is dice.
Suppose we throw a die and 1st it falls on the ground.
Then, its upper face will show one of the numbers 1, 2, 3, 4, 5 and 6.
Whatever number comes up, is called an outcome.
All possible outcomes are 1, 2, 3, 4, 5, 6.
Drawing a card from a well- shuffled of 52 cards
A deck of playing cards has in all 52 each.
It has 13 cards of each of 4 suits, namely Spades, clubs, hearts and diamonds.
(i) Cards of spades and clubs are black cards.
(ii) Cards of hearts and diamonds are red cards.
(iii) kings, queens and jacks (or knaves) are known as face cards.
Thus, there are in all 12 face cards.
SUMMARY
- In tossing a coin. all possible outcomes are H, T.
- In tossing 2 coins, all possible outcomes are HH, HT, TH, TT.
- On rolling a die, all possible outcomes are 1, 2, 3, 4, 5, 6.
- In drawing a card from a well-shuffled deck of 52 cards, number of all possible outcomes is 52.
EVENT
The collection of some or all possible outcomes is called an event.
On throwing a coin
(i) H is the event of getting a head;
(ii) T is the event of getting a tail.
On throwing two coins simultaneously
- (i) HH is the event of getting head on each coin;
- (ii) HT is the event of getting head on 1st coin and tail on 2nd coin;
- (iii) TH is the event of getting tail on 1st coin and head on 2nd coin;
- (iv) TT is the event of getting tail on each coin.
In rolling a die
(i) getting a number, say 4, is an event;
(ii) getting an even number is an event E containing 2, 4, 6,
(iii) getting an odd number is an event F containing 1, 3, 5.
Probability of Occurrence of an Event
Let E be an event. Then, the probability of occurrence of E is defined as
P (E) = Number of favourable outcomes/ Total number of outcomes
The adjoining figure shows a spinning wheel divided into eight sectors. Three of these sectors are painted red and the remaining five are painted white, as shown. The wheel is Spinned. What is the probability of getting (i) a white sector? (ii) a red sector?
Total number of sectors = 8
(i) Number of white sector) = 5
So, p (getting a white sector) = 5/8.
(ii) Number of red sectors = 3.
So, p (getting a rad sector) = 3/8.
A bag contains 6 red and 8 green balls. They are mixed thoroughly and one ball is drawn at random. Find the probability of getting (i) a red ball, (ii) a green ball.
Total number of balls = (6 + 8) = 14.
(i) Number of red balls = 6.
So, P (getting a red ball) = 6/14 = 3/7
(ii) Number of green balls = 8.
So, P (getting a green ball) = 8/14 = 4/7
What are some real life examples of probability?
- Card Games. Have you ever wondered why some poker hands are more valuable than others?
- Sports Statistics.
- Natural Disasters.
- Getting Dressed.
- Winning the Lottery.
- Buying Insurance.
- Predicting the Weather.
What event has a probability of 1?
The probability of an event is a number describing the chance that the event will happen. An event that is certain to happen has a probability of 1. An event that cannot possibly happen has a probability of zero.
What 3 ways can you write a probability?
A probability can be written as a ratio, a fraction, and a percent.