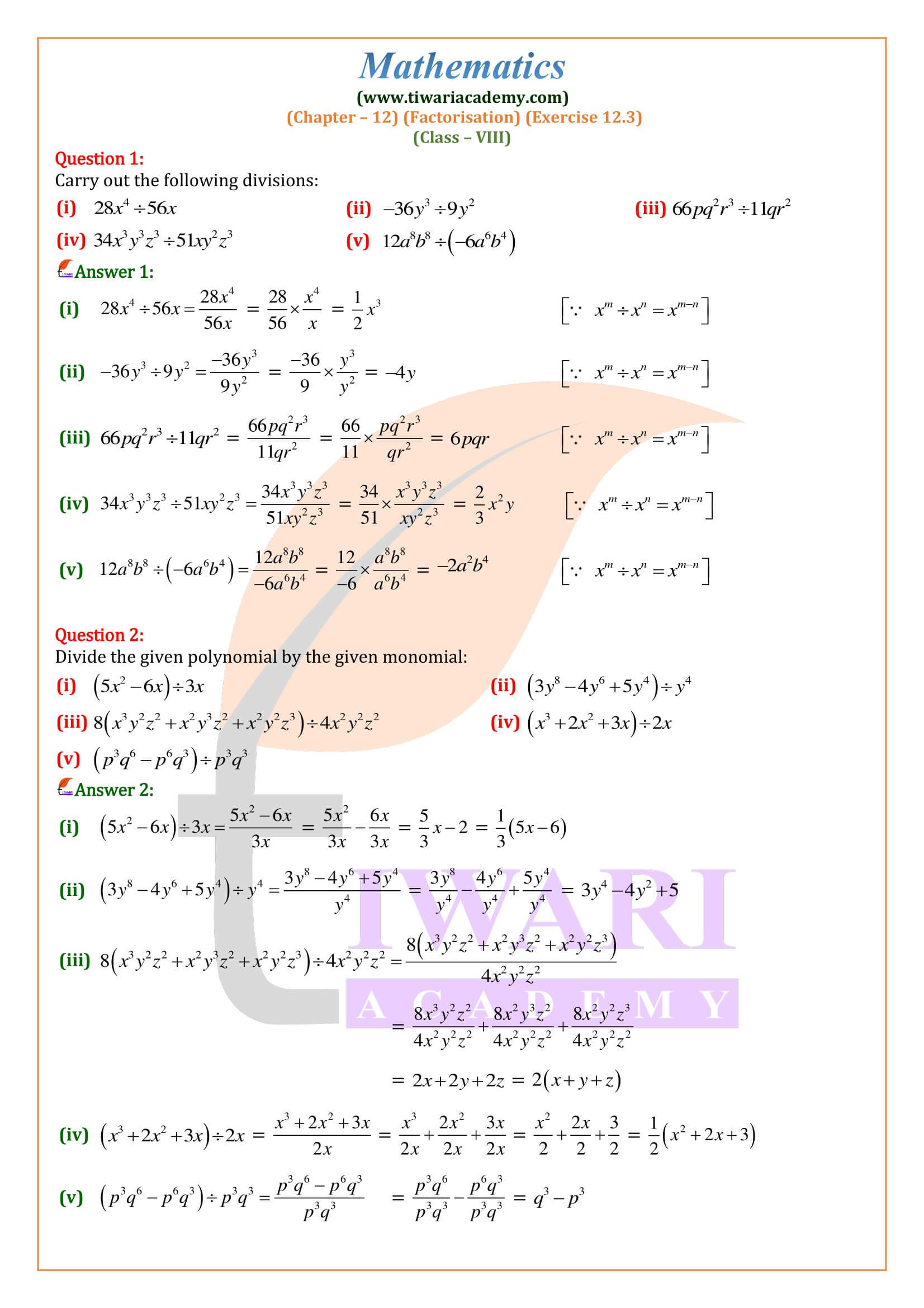
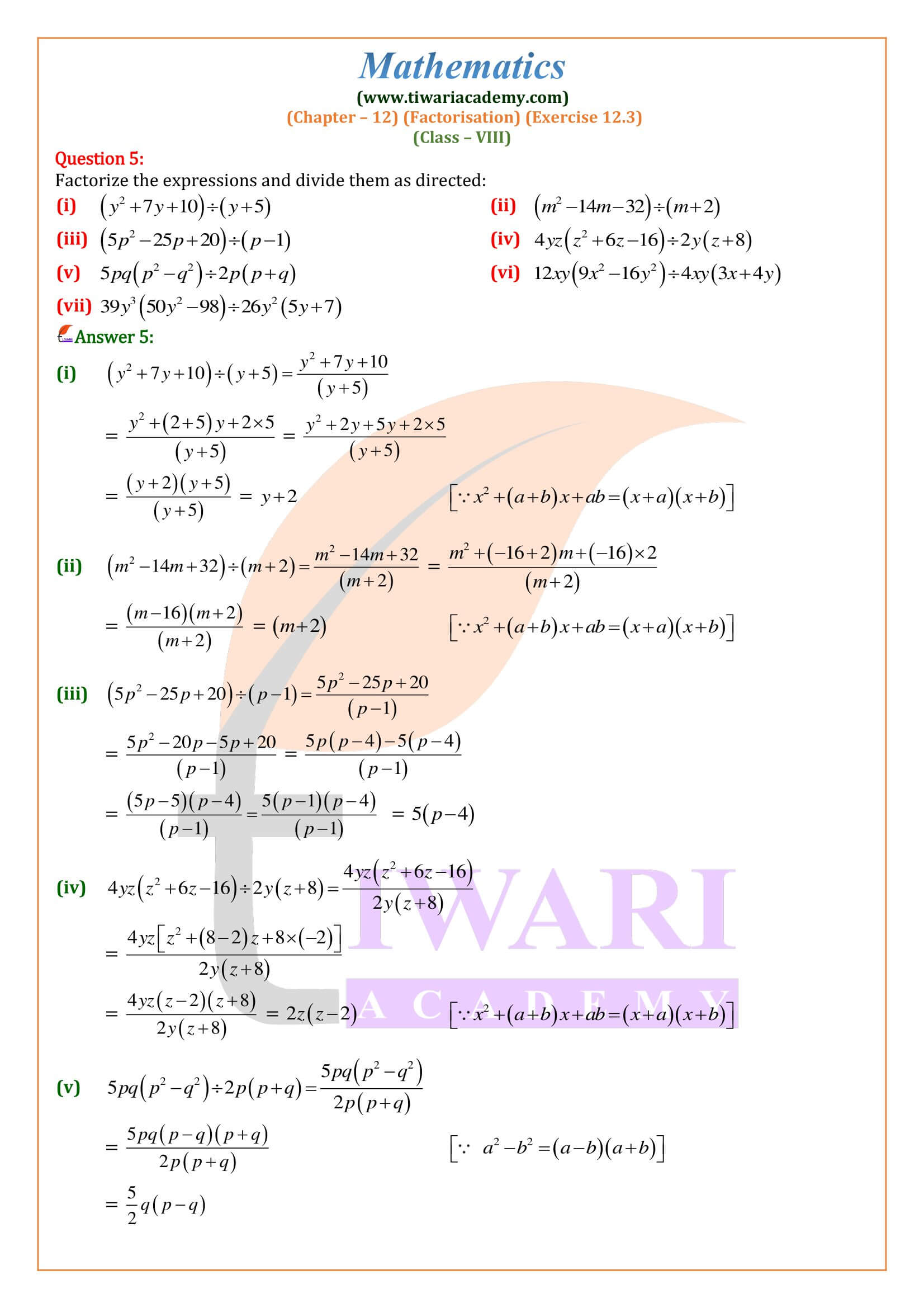
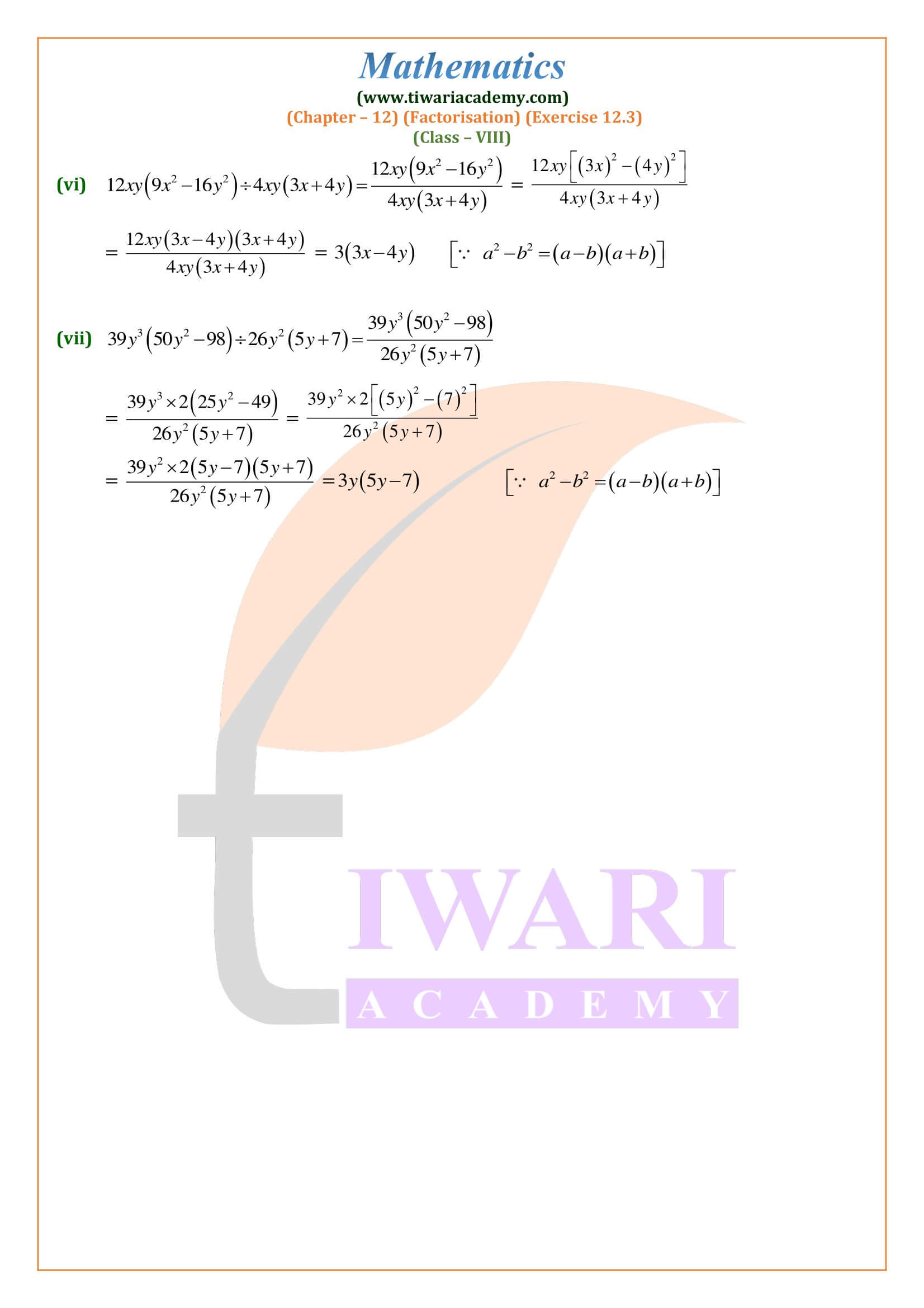
NCERT Solutions for Class 8 Maths Chapter 12 Exercise 12.3 Factorisation in Hindi and English Medium updated for CBSE session 2025-26. All the questions of ex. 12.3 class 8th mathematics are revised according to new syllabus and latest NCERT books issued for CBSE 2025-26.
8th Maths Exercise 12.3 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 12 | Exercise: 12.3 |
| Topic: | Factorisation |
| Content: | NCERT Book Solutions |
| Session: | CBSE 2025-26 |
| Medium: | Hindi and English Medium |
Class 8 Maths Chapter 12 Exercise 12.3 Solution
Class VIII Mathematics NCERT textbook Ex. 12.3 Factorisation updated for 2025-26 CBSE and state boards like MP board, UP board and other also. All the contents are prepared by subject experts using easier methods. Step by step solutions are given in PDF as well as videos solutions. Contact us if you are not getting solutions in videos or text, we here to help you.
Factorisation When Given Expression is Difference of Two Squares
FORMULA: (a – b)² = (a + b) (a – b)
Factorise: (i) 49x² – 16y² (ii) 64 – x² (iii) y² – 121
We have:
(i) 49x² – 16y²
= (7x)² – (4y)²
= (7x + 4y) (7x – 4y) {Applying formula (a – b)² = (a + b) (a – b)}
(ii) 64 – x²
= (8)² – (x)²
= (8 + x) (8 – x) {Applying formula (a – b)² = (a + b) (a – b)}
(iii) y² – 121
= (y)² – (11)²
= (y + 11) (y – 11) {Applying formula (a – b)² = (a + b) (a – b)}
Factorise: 25 (x + y)² – 36 (x – 2y)²
We have:
25 (x + y)² – 36 (x – 2y)²
= {5(x + y)}² – {6(x – 2y)}²
= {5 (x + y) + 6(x – 2y)} {5(x + y) – 6(x – 2y)}
{Applying formula (a – b)² = (a + b) (a – b)}
= (11x – 7y) (7y – x)
Factorisation When Expression is a Perfect Square (Using Identilies)
FORMULAE:
- a² + b² + 2ab = (a + b)²
- a² + b² – 2ab = (a – b)²
Factorise: (i) x² + 10x + 25 (ii) x² – 20x + 100
We have:
(i) x² + 10x + 25
= X2 + (5)² + 2 X x X 5 = (x + 5)² {By applying formula: a² + b² + 2ab = (a + b)²}
(ii) x² – 20x + 100
= x² + 10² – 2 X x X 10 = (x – 10)² {By applying formula: a² + b² – 2ab = (a – b)²}
Factorise: 4x² + 9y² + 12xy
We have:
4x² + 9y² + 12xy
= (2x)² + (3y)² + 2 X 2x X 3y
= (2x + 3y)²
Factorise: x² + 8x + 16
We have:
x² + 8x + 16
we can write this expression in the form of a² + b2 + 2ab
= x² + 4² + 2 X 4 X x
= (x + 2)²
Factorise: p² – 10p + 25
We have:
p² – 10p + 25 we can write this expression in the form of a² + b² – 2ab
= p² + 5² – 2 x p x 5
= (p – 5)² {By applying formula: a² + b² – 2ab = (a – b)²}
Factorise: a⁴ + 25b⁴ – 10a² b²
We can write this expression in the form of a² + b² – 2ab
= (a²)² + (5b²)² – 2 x a² x 5b²
= (a² – 5b²)²
{By applying formula: a² + b² – 2ab = (a – b)²}