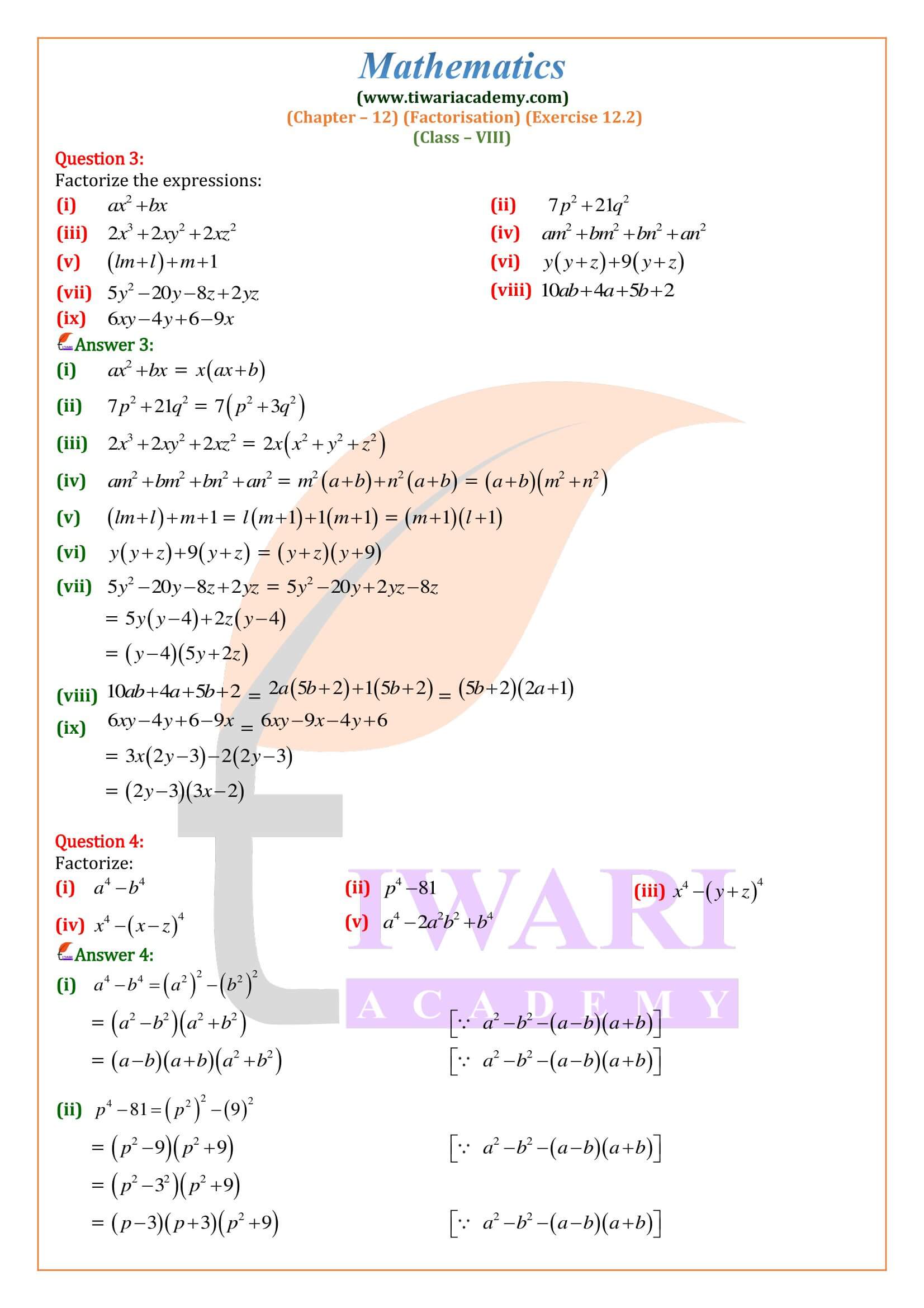
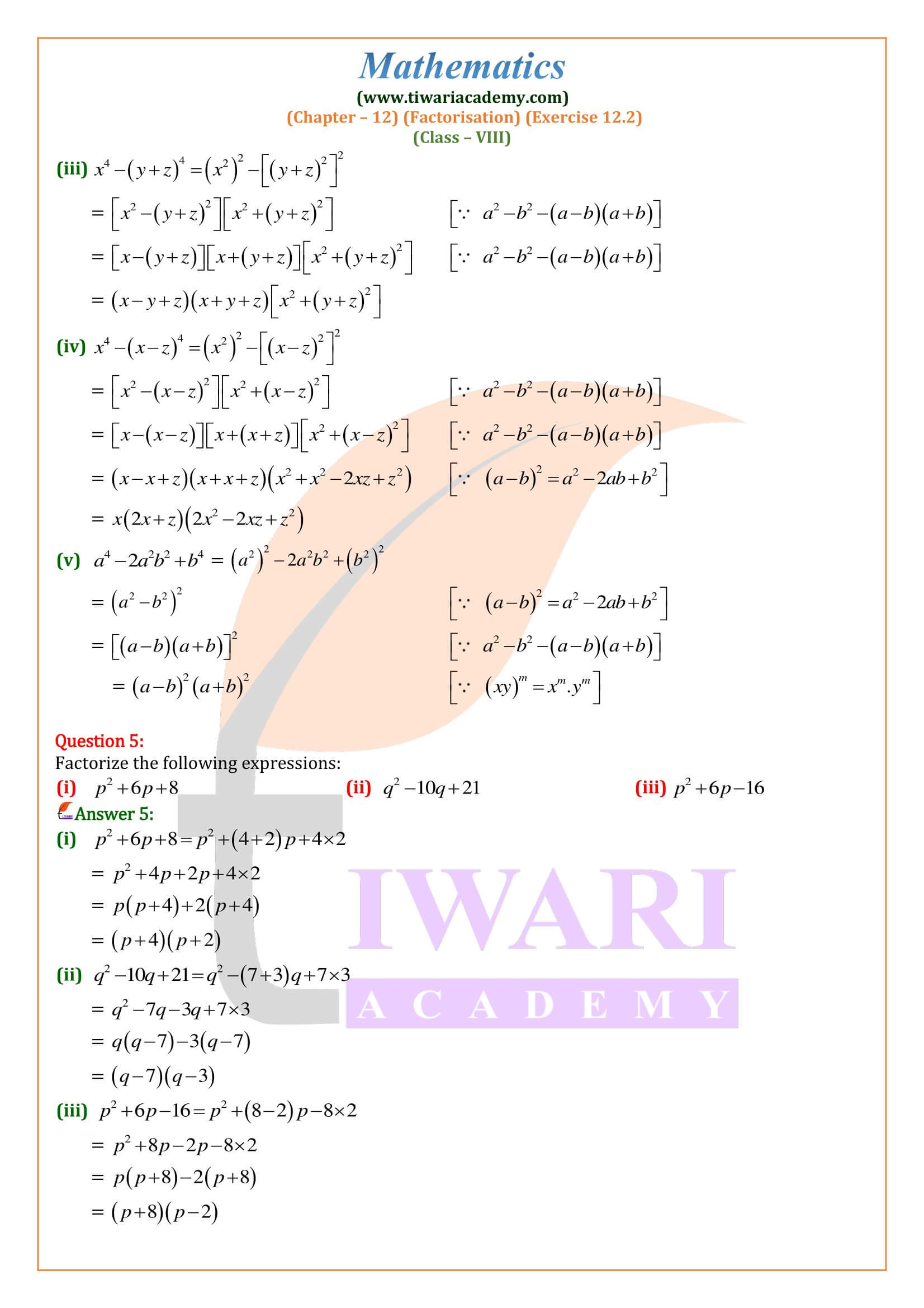
NCERT Solutions for Class 8 Maths Chapter 12 Exercise 12.2 Factorisation in Hindi and English Medium updated for CBSE 2025-26 exams. Get here the revised solutions of ex. 12.2 class 8 mathematics based on new syllabus and latest NCERT textbooks issued for CBSE academic session 2025-26.
8th Maths Exercise 12.2 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 12 | Exercise: 12.2 |
| Topic Name: | Factorisation |
| Medium: | Hindi and English Medium |
| Academic Session: | CBSE 2025-26 |
| Content: | NCERT Exercise Solution |
Class 8 Maths Chapter 12 Exercise 12.2 Solution
Class VIII Mathematics Ex. 12.2 Factorisation in PDF file format free to use online or download for CBSE Examination 2025-26. Students can use entire solutions without any login or registration. Exercise 12.2 of class 8th mathematics NCERT Book contains the questions based on identities. The use of these identities is also helpful in further classes. Take help from videos, if face problem to understand through PDF.
Factorisation When a Binomial is Common
Method of Factorisation
Step-1: Find the common binomial.
Step-2: Write the given expression as the product of this binomial and the quotient obtained on dividing the given expression by this binomial.
Factorise: (i) 5a (2x 3y) + 2b (2x 3y), (ii) 8 (4x + 5y) – 12 (4x + 5y)
We have:
(i) 5a (2x 3y) + 2b (2x 3y) = (2x 3y) (5a + 2b).
(ii) 8x (4x + 5y) – 12 (4x + 5y) = 4 (4x + 5y) {2(4x + 5y) – 3} = 4(4x + 5y) (8x + 10y – 3).
Factorisation by Grouping
Something the terms of the given expression need to be arranged in suitable groups in such a way that all the groups have a common factor. After this arrangement factorisation becomes easy.
Method for Group Factorisation
Step-1: Arrange the terms of the given expression in groups in such a way that all the groups have a common factor.
Step-2: Factorise each group.
Step-3: Take out the factor which is common to all such groups.
Factorise: (i) a² + bc + ab + ac, (ii) ax² + by² + bx² + ay²
By suitably rearranging the terms, we have:
(i) a + bc + ab + ac = a + ab + ac + bc
= a(a + b) + c (a + b)
= (a + b) (a + c)
(ii) ax² + by² + bx² + ay² = ax² + ay² + bx² + by²
= a(x² + y²) + b(x² + y²)
= (x² + y²) (a + b)
What are Binomials in Maths?
In algebra, a binomial is a polynomial that is the sum of two terms, each of which is a monomial. It is the simplest kind of polynomial after the monomials.
What is the importance of factoring polynomials in our daily life?
The purpose of factoring such functions is to then be able to solve equations of polynomials. For example, the solution to x² + 5x + 4 = 0 are the roots of x² + 5x + 4, namely, -1 and -4. Being able to find the roots of such polynomials is basic to solving problems in science classes in the following 2 to 3 years.
How do you do common factorization?
To factorise an algebraic expression, take out the highest common factor and place it in front of the brackets. Then the expression inside the brackets is obtained by dividing each term by the highest common factor.