NCERT Solutions for Class 8 Maths Chapter 10 Exercise 10.1 Exponents and Powers in Hindi and English Medium updated for session 2025-26. The solutions of questions in ex. 10.1 of class 8th mathematics are revised according to the latest NCERT textbooks issued for academic year 2025-26.
8th Maths Exercise 10.1 Solution in Hindi and English Medium
| Class: 8 | Mathematics |
| Chapter: 10 | Exercise: 10.1 |
| Chapter Name: | Exponents and Powers |
| Content Type: | PDF and Videos Solution |
| Academic Session: | CBSE 2025-26 |
| Medium: | Hindi and English Medium |
Class 8 Maths Chapter 10 Exercise 10.1 Solution
Class VIII Mathematics NCERT textbook Ex. 10.1 Exponents and Powers in Hindi and English Medium updated for CBSE session 2025-26 free to use or download. Learn here how to operate exponents of real number, what is the way to solve negative exponents and other similar questions. Videos and PDF solutions of NCERT are given separately. If students feel difficulty in PDF solutions, they may refer exercise 10.1 explanation video solution.
Exponents (Powers)
We have already learnt that 2 x 2 x 2 x 2 can be written in the exponential form as 2⁴, where 2 is the base and 4 is the exponent. It is read as “two raised to the power 4.”
Thus, if x is a rational number and n is a positive integer, then xⁿ = x X x X x …… n times, where x is the base and n is called exponent or power.
Let us recall that for positive integers a and n, we have:
(-a)ⁿ = (an when n is even, -an when n is odd).
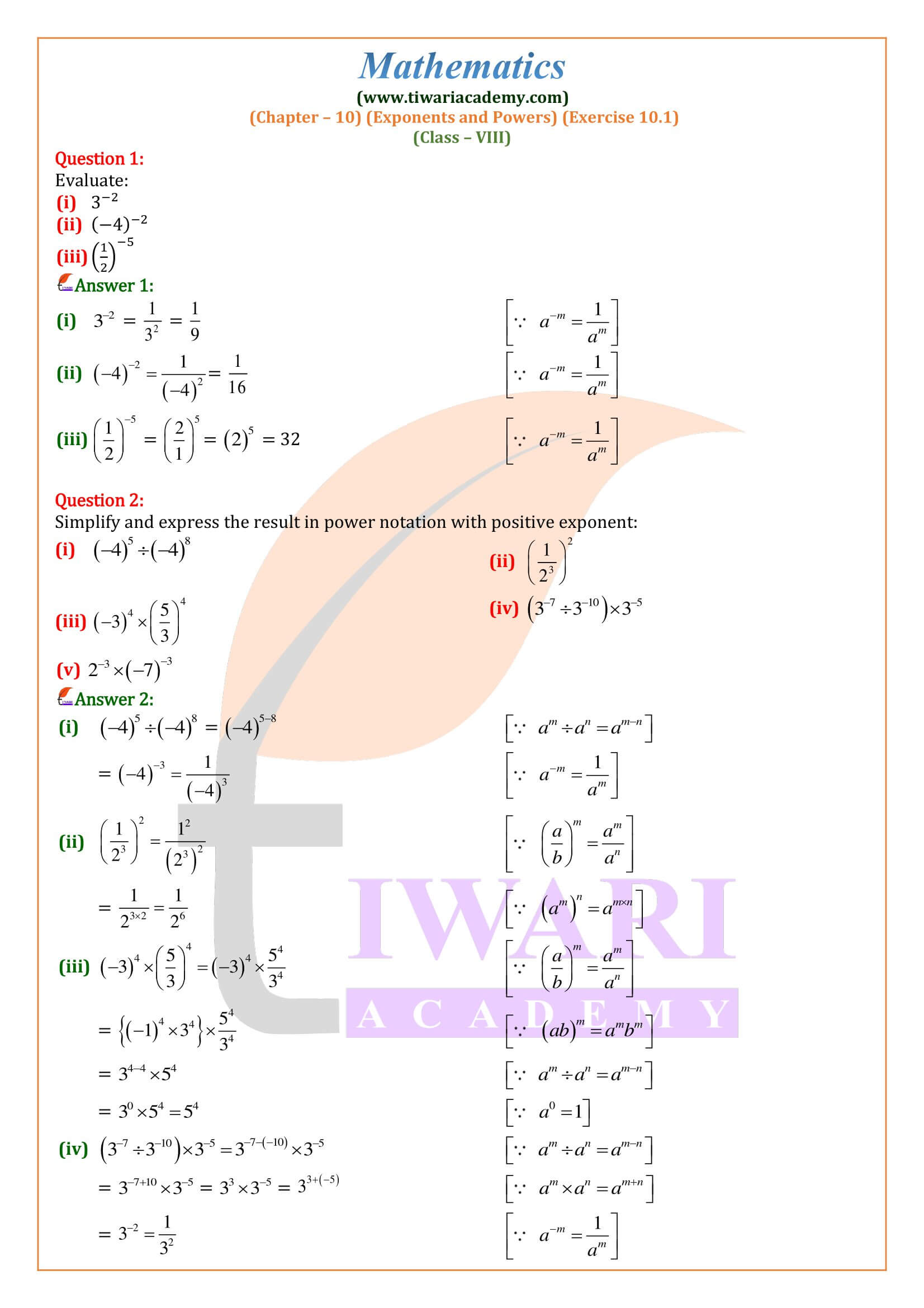
Write down in expanded form: (i) (-2)⁴, (ii) (-2)³
(i) (-2)⁴ = (-2) x (-2) x (-2) x (-2) = 16 =2⁴
(ii) (-2)³ = (-2) x (-2) x (-2) = -8 = (-2)³
In this chapter, we shall be dealing with the exponents of rational numbers.
Positive integral Exponent of a Rational Number
Let a/b be any rational number and n be a positive integer. Then,
(a/b)ⁿ =
a/b x a/b x a/b x………..n times
= (a x a x a x ….n times)/ (b x b x b x……..n times)
= aⁿ /bⁿ
Thus, (a/b)ⁿ = aⁿ /bⁿ for every positive integer n.
Evaluate: (i) (3/5)³ (ii) (-3/4)⁴
We have:
(i) (3/5)³
= 3³ /5³
= 27/125
(ii) (-3/4)⁴
= (-3)⁴ /4⁴
= (-3 x -3 x -3 x -3)/(4 x 4 x 4 x 4)
= 81/256
Negative Integral Exponent of a Rational Number
Let a/b be any rational number and n be a positive integer.
Then, we define, (a/b)⁻ⁿ = (b/a)ⁿ
Example
(i) (¾)⁻⁵ = (4/3)⁵
(ii) (4)⁻³ = (1/4)³
Laws of Exponents
Let a/b be any rational number. and m and n be any integers. Then, we have:
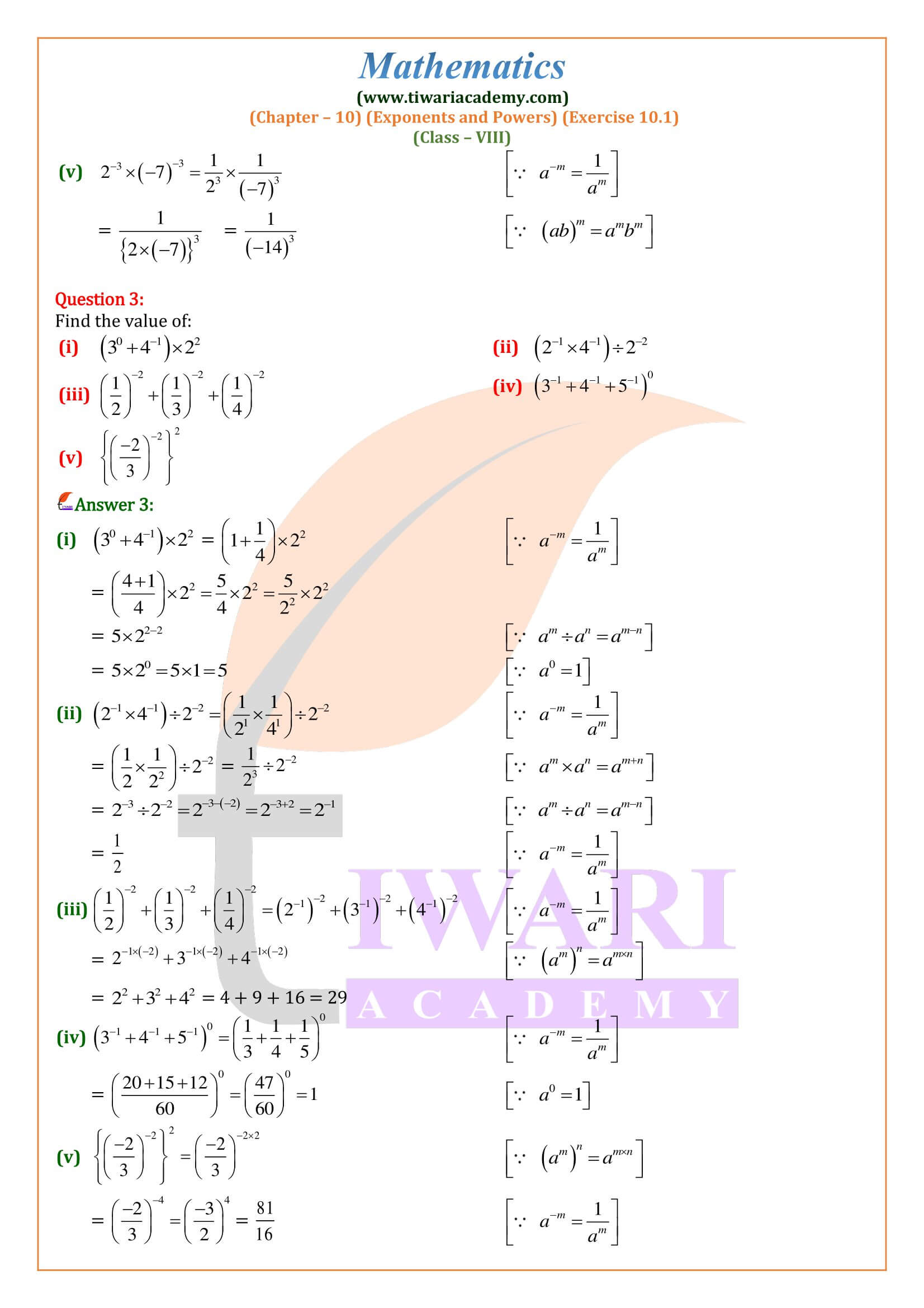
- (i) (a/b)ᵐ x (a/b)ⁿ = (a/b)ᵐ⁺ⁿ
- (ii) (a/b)ᵐ / (a/b)ⁿ = (a/b)ᵐ⁻ⁿ
- (iii) {(a/b)ᵐ }ⁿ = (a/b)ᵐⁿ
- (iv) {(a/b) x (c/d)}ⁿ= (a/b)ⁿ x (c/d)ⁿ
- (v) (a/b)⁻ᵐ = (b/a)ᵐ
- (vi) (a/b)⁰ = 1
How are exponents used in everyday life?
Another example of using exponents in real life is when you calculate the area of any square. If you say “My room is twelve-foot by twelve-foot square”, you’re meaning your room is 12 feet × 12 feet. 12 feet multiply by itself — which can be written as (12 ft)². And that simplifies to 144 square feet.
How do you solve problems with exponents?
When you multiply two exponents with the same base, you can simplify the expression by adding the exponents. Do NOT add or multiply the base., you simply have to solve the exponents separately and then multiply the two numbers.
What are the 3 rules of exponents?
- To multiply identical bases, add the exponents.
- To divide identical bases, subtract the exponents.
- When there are two or more exponents and only one base, multiply the exponents.
How are exponents added?
Add the coefficients of the variables leaving the exponents unchanged. Only terms that have same variables and powers are added. This rule agrees with the multiplication and division of exponents as well.
What do you learn in Exercise 10.1 of class 8 Mathematics NCERT textbook?
In exercise 10.1 of Chapter 10 from the Class 8 NCERT Maths textbook, students can learn about the concepts of Exponents and Powers. These concepts are divided into two exercises. Such concepts are:
- Understanding the concept of an exponent and how it relates to powers of numbers.
- Exponents to express large numbers in a compact form.
- The laws of exponents, such as the product of powers, quotient of powers, power of a power, power of a product, and power of a quotient.
- Simplifying expressions with exponents and powers to evaluate the numbers and equations.
- Moreover, it will also provide you with an understanding of the relationship between exponents and multiplication and division.
Is exercise 10.1 of 8th Mathematics NCERT easy?
Exercise 10.1 of chapter 10 Exponents and powers from Class 8 Maths NCERT solutions is considered easier than other exercises. It requires a sound understanding of the concepts of powers and exponents. To make it simpler you can break down the concepts into different smaller question that makes it more manageable. A fine grip of multiplication and division is useful here.
How many questions are there in exercise 10.1 of class VIII mathematics?
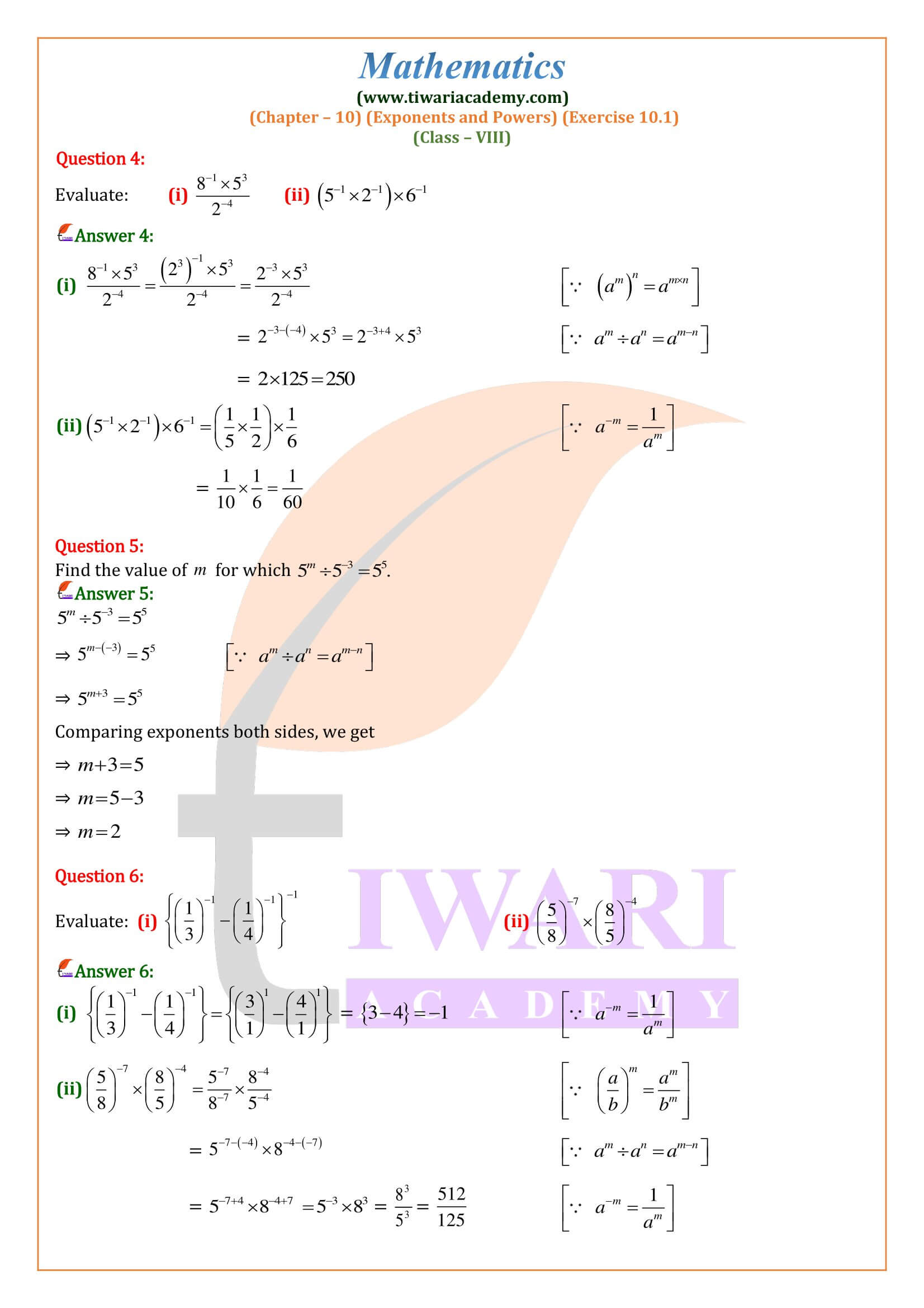
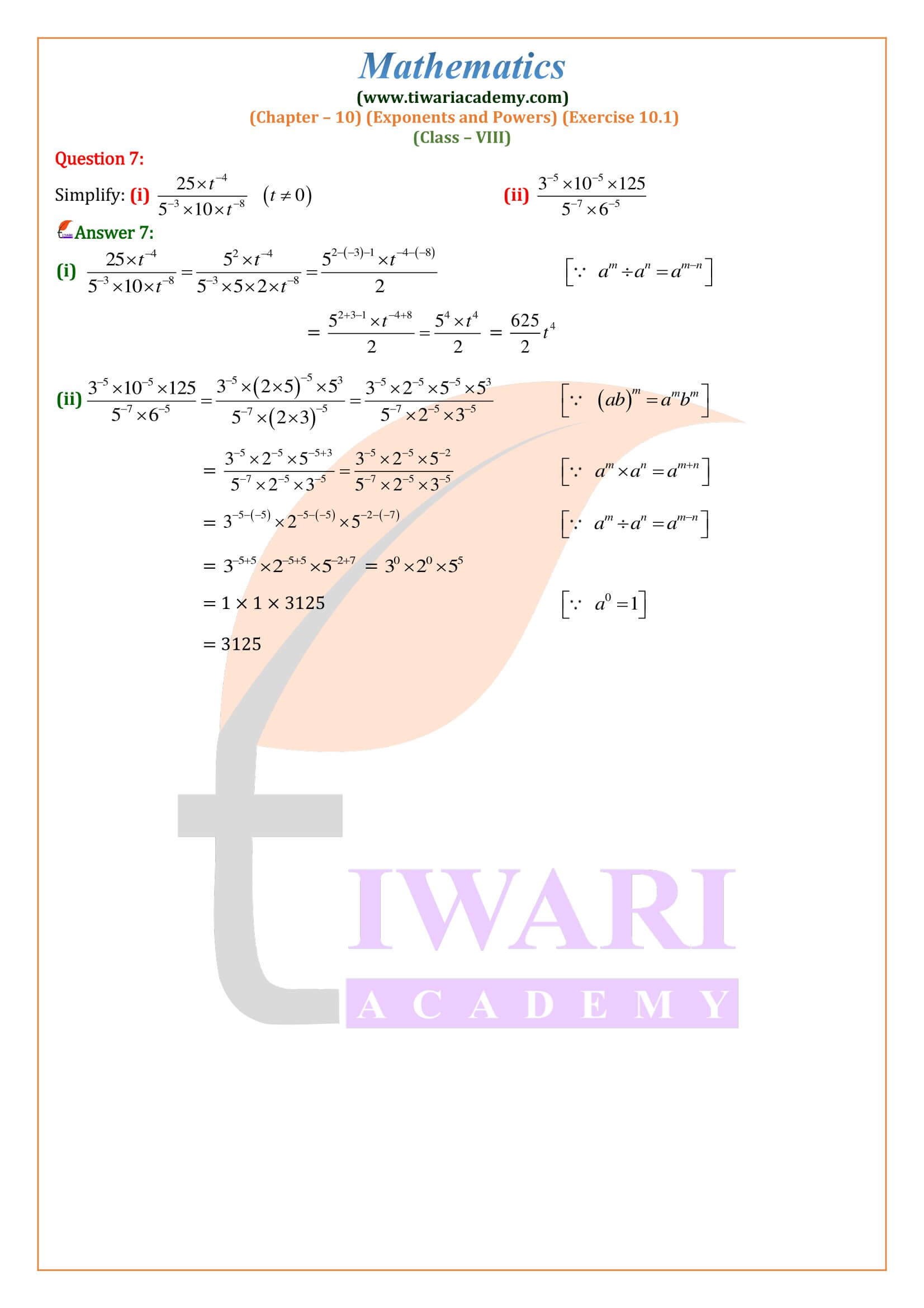
There are 7 questions in exercise 10.1 of 8th Maths NCERT solutions. These questions have different parts and are based on example numbers 1, 4, 6, and 7.