NCERT Solutions for Class 8 Maths Chapter 1 Exercise 1.2 Rational Numbers in Hindi and English Medium for CBSE and State Boards. Get expert NCERT solutions for Class 8 Maths Chapter 1 Exercise 1.2 on Rational Numbers. Available in Hindi and English at Tiwari Academy for both CBSE and state board students.
8th Maths Exercise 1.2 Solution in Hindi and English Medium
Class 8 Maths Chapter 1 Exercise 1.2 Solution in Hindi and English
Class VIII Mathematics NCERT book Ex. 1.2 question answers are available in Hindi and English Medium free to use or download. If someone is facing difficulty in solving questions, he can use videos solutions available on website and Apps. Exercise 1.2 solutions of 8th standard Maths are updated for new academic year based on latest CBSE books.
| Class: 8 | Mathematics |
| Chapter: 1 | Exercise: 1.2 |
| Topic Name: | Rational Numbers |
| Content Type: | Text and Videos Format |
| Medium: | Hindi and English Medium |
Additive Identity
Check the result if you add 0 to a whole number, the sum is again that whole number. This holds for integers as well as rational numbers also. For example, a + 0 = 0 + a = a, where a is a whole number, b + 0 = 0 + b = b, where b is an integer, c + 0 = 0 + c = c, for a rational number c. Here, zero (0) is the identity for the addition of rational numbers. It is the additive identity for integers as well as whole numbers also.
Rational Numbers between two Numbers
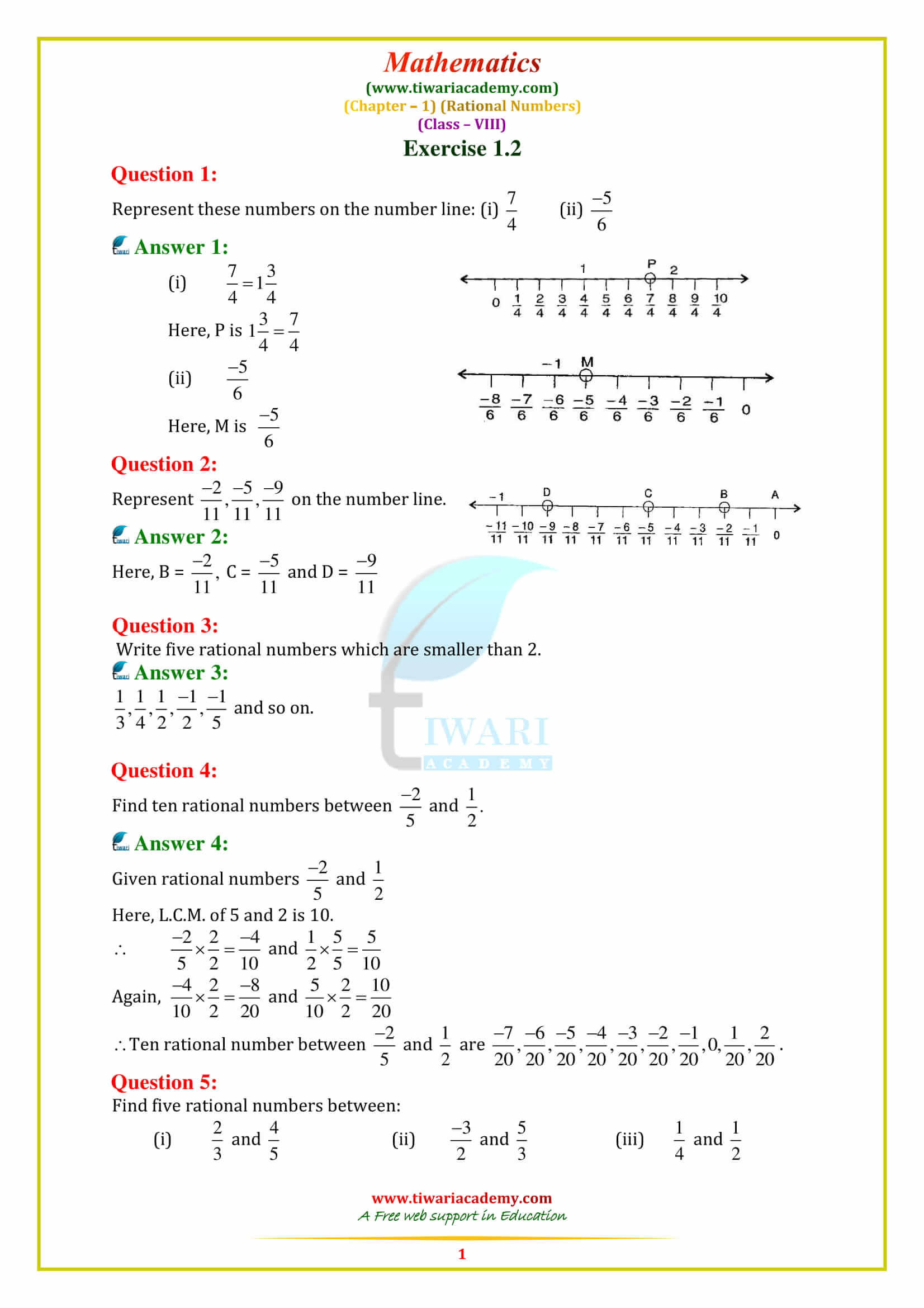
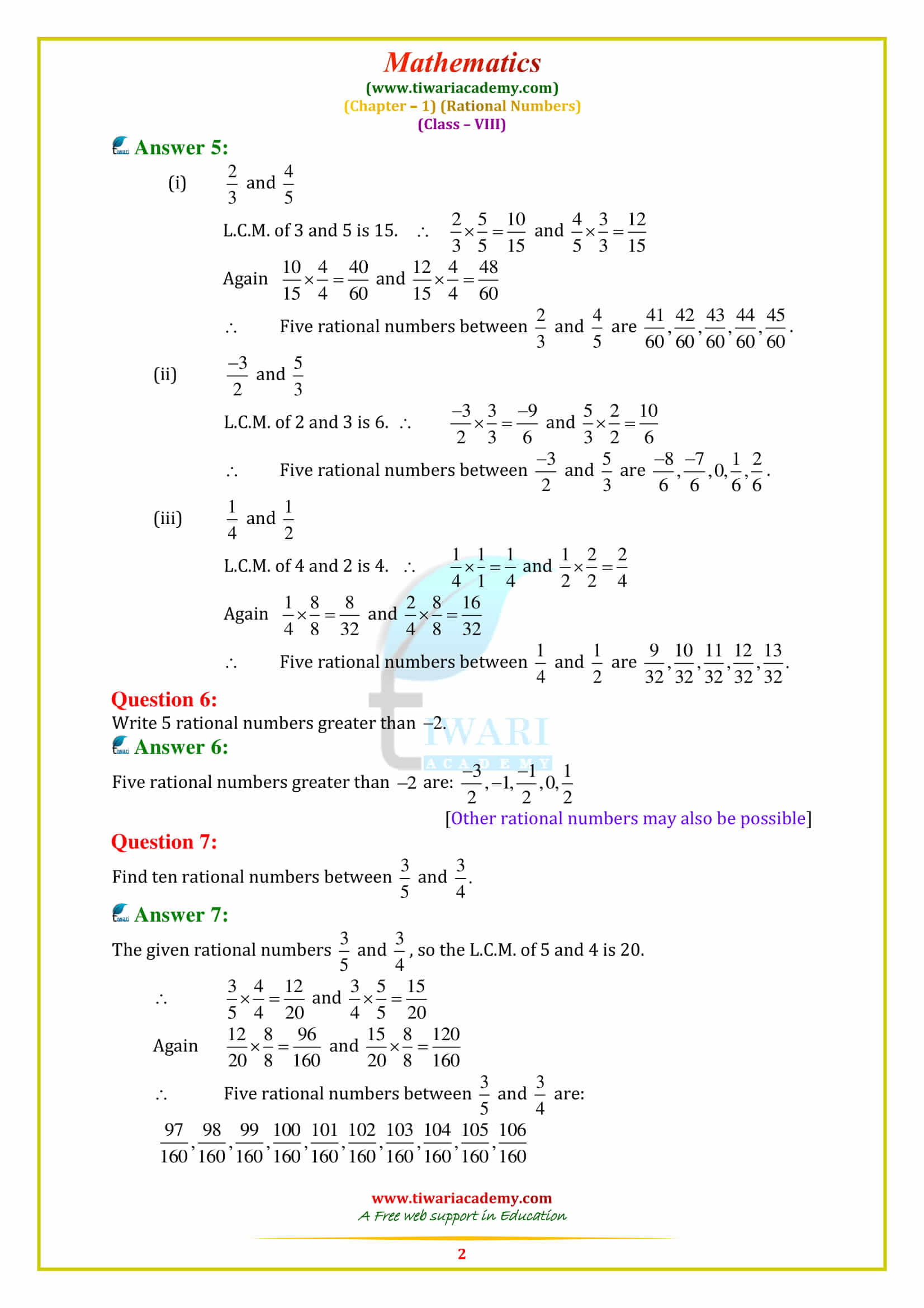
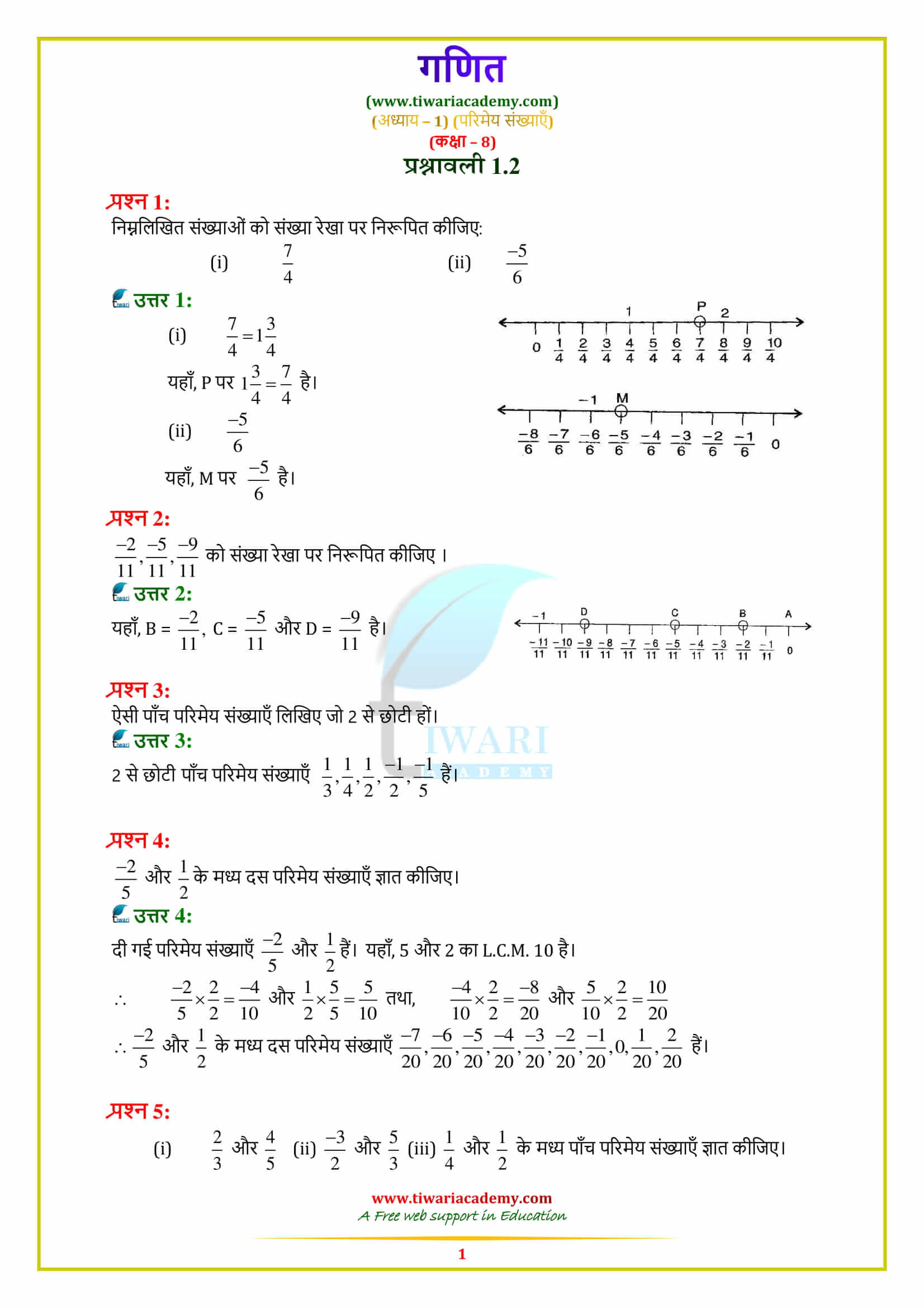
We can represent any rational number on a number line. In a rational number, the number below the bar, that is, the denominator indicates the number of equal parts into which the first unit is divided. The number above the bar, that is, the numerator, shows “how many” of these parts are considered. You know, we get innumerable rational numbers between two given rational numbers. Using the concept of the mean we can find rational numbers between two rational numbers. We know that between two given numbers, we don’t need to obtain a whole number, but there will always be a rational number.
Multiplicative Identity
We will find that when we multiply any rational number with 1, we get back the same rational number as the product. We can check this for a few more rational numbers. Naturally, we will find that, a × 1 = 1 × a = a for any rational number a. So, in this way for rational numbers 1 is the multiplicative identity.
Class 8 Maths Exercise 1.2 Important Questions
How many rational numbers are there between two rational numbers?
There are infinite number of rational numbers are there between two rational numbers.
Other than NCERT, which book is best for practicing rational numbers.
In NCERT Exemplar Problem, a lots of questions are given for practicing rational numbers. Students must refer this book for a better practice.
Is chapter 1 of class 8 Maths useful for class 9 Maths also?
Yes, in class 9 Maths, about 20 percent of chapter 1 is same as in class 8 Maths chapter 1 rational numbers.
How is Tiwari Academy helpful in studies as compared to other websites?
Tiwari Academy is free to use and no login is required. They never call or message to the uses to compel buying their product. Tiwari Academy website or apps just support a hassle free education.
Closure Property for Integers
| Operation | Numbers | Remarks |
|---|---|---|
| Addition | – 6 + 5 = – 1, an integer, Is – 7 + (–5) an integer? Is 8 + 5 an integer? In general, a + b is an integer for any two integers a and b. | Integers are closed under addition. |
| Subtraction | 7 – 5 = 2, an integer. Is 5 – 7 an integer? – 6 – 8 = – 14, an integer – 6 – (– 8) = 2, an integer Is 8 – (– 6) an integer? In general, for any two integers a and b, a – b is again an integer. Check if b – a is also an integer. | Integers are closed under subtraction. |
| Multiplication | 5 × 8 = 40, an integer. Is – 5 × 8 an integer? – 5 × (– 8) = 40, an integer. In general, for any two integers a and b, a × b is also an integer. | Integers are closed under multiplication. |
| Division | 2 ÷ 3 or 2/3, which is not an integer. | Integers are not closed under division. |