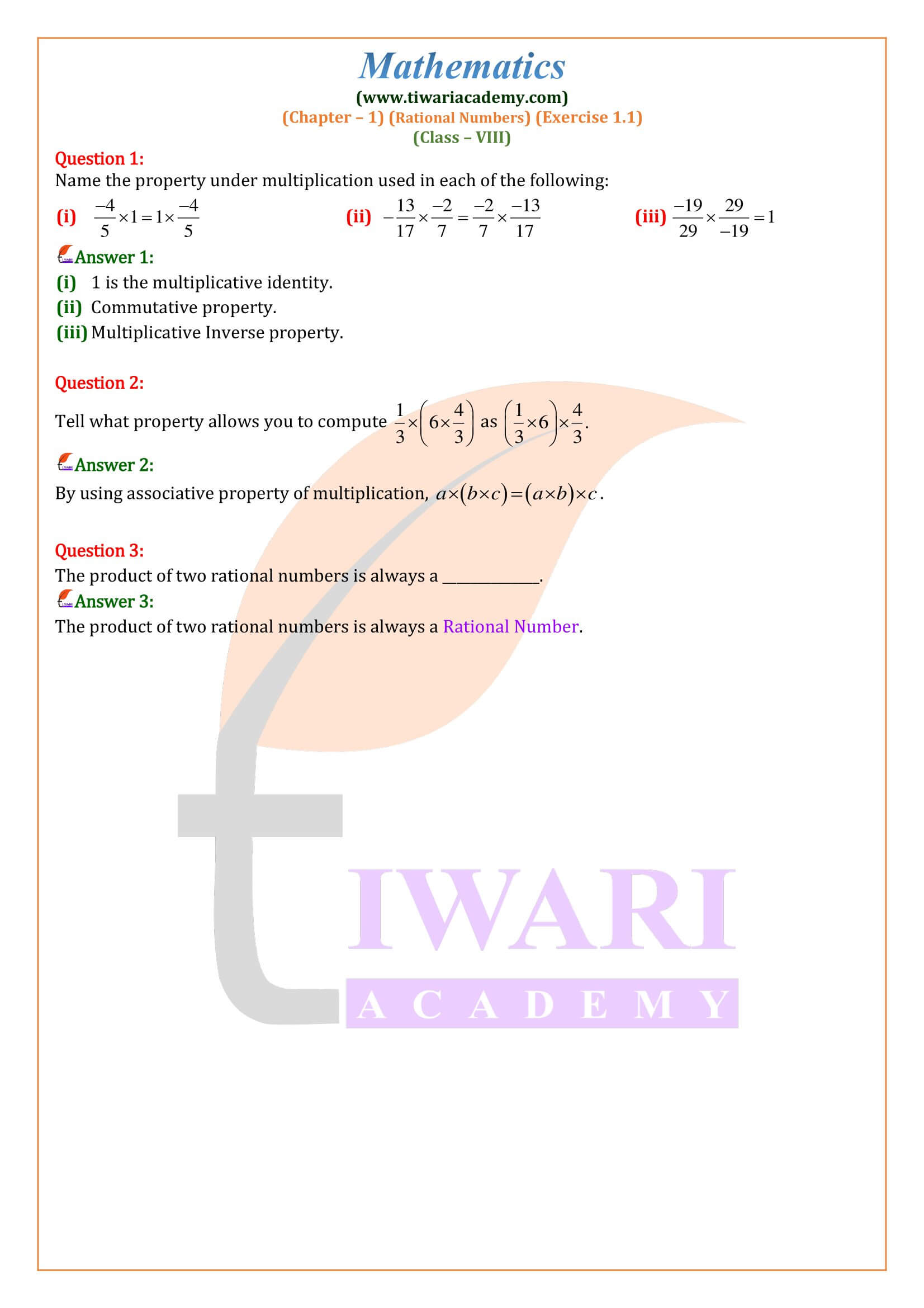
NCERT Solutions for Class 8 Maths Chapter 1 Exercise 1.1 Rational Numbers for academic year 2025-26 in Hindi and English Medium. According to new syllabus and latest NCERT books issued for 2025-26, there is only one exercise in 8th Maths chapter 1 Rational Numbers.
Class 8 Maths Exercise 1.1 Solutions
Class 8 Maths Exercise 1.1 in Hindi Medium
Class 8 Maths NCERT Book Download
Class 8 Maths Chapter 1 Solutions
Class 8 Maths NCERT Solutions
Class 8 all Subjects Solutions
8th Maths Exercise 1.1 Solution in Hindi and English Medium
Standard 8 Mathematics NCERT Ex. 1.1 solutions are given here in Hindi and English Medium free to use online or download in PDF file format. All the contents are updated for academic session 2025-26 based on latest CBSE NCERT Textbooks. Videos related to exercise 1.1 of CBSE 8 Maths are also given, so that students can understand questions easily.
| Class: 8 | Mathematics |
| Chapter: 1 | Exercise: 1.1 |
| Chapter Name: | Rational Numbers |
| Medium: | Hindi and English Medium |
| Academic Session: | Year 2025-26 |
| Content: | NCERT Exercise Solutions |
In mathematics, we use simple equations to solve. When we solve an equation in x, the value of x satisfies the given equation. The solution can be a natural number, a whole number, a rational number or an integer. It all depends on the equation and coefficient of the variable. Here, we will discuss rational numbers and their operation through various properties.
Closure Properties of Whole Numbers
Here, we will learn how whole numbers pass in addition and multiplication, but not in subtraction and division. However, whole numbers come under addition, subtraction and multiplication, but not under division.
Class 8 Maths Exercise 1.1 Important Questions
Are the rational numbers are closed under the operations of division?
No, because the division of 0 by a non-zero number is not possible.
Can you find the additive identity for a rational number?
Zero (0) is the additive identity for rational numbers.
What is the multiplicative identity of whole numbers?
The number 1 is the multiplicative identity for whole numbers.
What is the distributive law of rational numbers?
Distributive law of rational numbers:
For all rational numbers a, b and c,
a(b + c) = ab + ac and a(b – c) = ab – ac
Associative Property of Rational Numbers
The addition is associated with rational numbers. So, for any three given rational numbers a, b, and c, a + (b + c) = (a + b) + c. Subtraction is not a companion to rational numbers. Multiplication is a companion to rational numbers. So, for any three given rational numbers a, b, and c, a × (b × c) = (a × b) × c. The division is not an ally for rational numbers.
Closure of assets rational number
Also, the rational numbers are close. so, the product of any two rational numbers a and b, a b is also a rational number. Similarly, the rational number is closed by subtraction. The difference a-b, of any two rational numbers a and b, is also a rational number. After examining the product, we find that the rational numbers are closed with multiplication. That is, for any two rational numbers a and b, × b is also a rational number, but the rational number does not stop for the division.
Closure Property of Whole numbers
| Operation | Numbers | Remarks |
|---|---|---|
| Addition | 0 + 5 = 5, a whole number 4 + 7 = … . Is it a whole number? In general, a + b is a whole number for any two whole numbers a and b. | Whole numbers are closed under addition. |
| Subtraction | 5 – 7 = – 2, which is not a whole number. | Whole numbers are not closed under subtraction. |
| Multiplication | 0 × 3 = 0, a whole number 3 × 7 = … . Is it a whole number? In general, if a and b are any two whole numbers, their product ab is a whole number. | Whole numbers are closed under multiplication. |
| Division | 5 ÷ 8 = 5/8, which is not a whole number. | Whole numbers are not closed under division. |